Reasoning, convincing and proving

problem
Road maker

Age

14 to 18

Challenge level

Which of these roads will satisfy a Munchkin builder?
problem
Big and small numbers in physics - group task

Age

16 to 18

Challenge level

Work in groups to try to create the best approximations to these physical quantities.
problem
Impossible square?

Age

16 to 18

Challenge level

Can you make a square from these triangles?
problem
Consecutive negative numbers

Age

11 to 14

Challenge level

Do you notice anything about the solutions when you add and/or subtract consecutive negative numbers?
problem
T for tan

Age

16 to 18

Challenge level

Can you find a way to prove the trig identities using a diagram?
problem
9 weights

Age

11 to 14

Challenge level

You have been given nine weights, one of which is slightly heavier than the rest. Can you work out which weight is heavier in just two weighings of the balance?
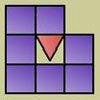
problem
Overlaps

Age

7 to 11

Challenge level

What does the overlap of these two shapes look like? Try picturing it in your head and then use some cut-out shapes to test your prediction.
problem
The great weights puzzle

Age

14 to 16

Challenge level

You have twelve weights, one of which is different from the rest. Using just 3 weighings, can you identify which weight is the odd one out, and whether it is heavier or lighter than the rest?
problem
Gutter

Age

14 to 16

Challenge level

Manufacturers need to minimise the amount of material used to make their product. What is the best cross-section for a gutter?
problem
Honey bees

Age

14 to 16

Challenge level

How many bees could fly 1000 miles if they had 10 gallons of honey?