Inequalities

problem
In between

Age

16 to 18

Challenge level

Can you find the solution to this algebraic inequality?
problem
Biggest enclosure

Age

14 to 16

Challenge level

Three fences of different lengths form three sides of an enclosure. What arrangement maximises the area?
problem
Eyes down

Age

16 to 18

Challenge level

The symbol [ ] means 'the integer part of'. Can the numbers [2x]; 2[x]; [x + 1/2] + [x - 1/2] ever be equal? Can they ever take three different values?
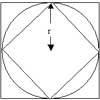
problem
Approximating pi

Age

14 to 18

Challenge level

By inscribing a circle in a square and then a square in a circle find an approximation to pi. By using a hexagon, can you improve on the approximation?
problem
Mediant madness

Age

14 to 16

Challenge level

Kyle and his teacher disagree about his test score - who is right?
problem
Plutarch's boxes

Age

11 to 14

Challenge level

According to Plutarch, the Greeks found all the rectangles with integer sides, whose areas are equal to their perimeters. Can you find them? What rectangular boxes, with integer sides, have their surface areas equal to their volumes?
problem
Comparing continued fractions

Age

16 to 18

Challenge level

Which of these continued fractions is bigger and why?
problem
Unit interval

Age

14 to 18

Challenge level

Take any two numbers between 0 and 1. Prove that the sum of the numbers is always less than one plus their product?
problem
Giants

Age

16 to 18

Challenge level

Which is the bigger, 9^10 or 10^9 ? Which is the bigger, 99^100 or 100^99 ?
problem
Inequalities

Age

11 to 14

Challenge level

A bag contains 12 marbles. There are more red than green but green and blue together exceed the reds. The total of yellow and green marbles is more than the total of red and blue. How many of each colour there are in the bag?