3 out of 3
From this sum of powers, can you find the sum of the indices?
3 out of 3
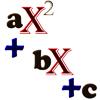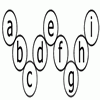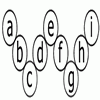Weekly Problem 26 - 2008
If $n$ is a positive integer, how many different values for the remainder are obtained when $n^2$ is divided by $n+4$?
3 out of 3
However did we manage before calculators? Is there an efficient way to do a square root if you have to do the work yourself?
3 out of 3
Tina has chosen a number and has noticed something about its factors. What number could she have chosen? Are there multiple possibilities?
2 out of 3
The nth term of a sequence is given by the formula n^3 + 11n. Find the first four terms of the sequence given by this formula and the first term of the sequence which is bigger than one million. Prove that all terms of the sequence are divisible by 6.
2 out of 3
In a snooker game the brown ball was on the lip of the pocket but it could not be hit directly as the black ball was in the way. How could it be potted by playing the white ball off a cushion?
2 out of 3
Take a few whole numbers away from a triangle number. If you know the mean of the remaining numbers can you find the triangle number and which numbers were removed?
1 out of 3
If x + y = -1 find the largest value of xy by coordinate geometry, by calculus and by algebra.
1 out of 3
The sum of any two of the numbers 2, 34 and 47 is a perfect square. Choose three square numbers and find sets of three integers with this property. Generalise to four integers.
1 out of 3
Show that for natural numbers x and y if x/y > 1 then x/y>(x+1)/(y+1}>1. Hence prove that the product for i=1 to n of [(2i)/(2i-1)] tends to infinity as n tends to infinity.
