Similarity and congruence

problem
Pythagoras proofs

Age

11 to 16

Challenge level

Can you make sense of these three proofs of Pythagoras' Theorem?
problem
Triangle in a trapezium

Age

11 to 16

Challenge level

Can you find and prove the relationship between the area of a trapezium and the area of a triangle constructed within it?
problem
The square under the hypotenuse

Age

14 to 16

Challenge level

Can you work out the side length of a square that just touches the hypotenuse of a right angled triangle?
problem
Kite in a square

Age

14 to 18

Challenge level

Can you make sense of the three methods to work out what fraction of the total area is shaded?
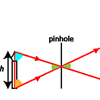problem
Pinhole camera

Age

11 to 14

Challenge level

Make your own pinhole camera for safe observation of the sun, and find out how it works.
problem
Stadium sightline

Age

14 to 18

Challenge level

How would you design the tiering of seats in a stadium so that all spectators have a good view?
problem
Fit for photocopying

Age

14 to 16

Challenge level

Explore the relationships between different paper sizes.
problem
It depends on your point of view!

Age

14 to 16

Challenge level

Anamorphic art is used to create intriguing illusions - can you work out how it is done?
problem
Folding fractions

Age

14 to 16

Challenge level

What fractions can you divide the diagonal of a square into by simple folding?
problem
Making sixty

Age

14 to 16

Challenge level

Why does this fold create an angle of sixty degrees?