Modular arithmetic

problem
Grid lockout

Age

14 to 16

Challenge level

What remainders do you get when square numbers are divided by 4?
problem
Differences

Age

11 to 14

Challenge level

Can you guarantee that, for any three numbers you choose, the product of their differences will always be an even number?
problem
Euler's officers

Age

14 to 16

Challenge level

How many different ways can you arrange the officers in a square?
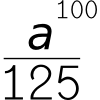problem
Remainder hunt

Age

16 to 18

Challenge level

What are the possible remainders when the 100-th power of an integer is divided by 125?
problem
Prime AP

Age

16 to 18

Challenge level

What can you say about the common difference of an AP where every term is prime?
problem
Novemberish

Age

14 to 16

Challenge level

a) A four digit number (in base 10) aabb is a perfect square. Discuss ways of systematically finding this number. (b) Prove that 11^{10}-1 is divisible by 100.
problem
Mod 7

Age

16 to 18

Challenge level

Find the remainder when 3^{2001} is divided by 7.
problem
Mod 3

Age

14 to 16

Challenge level

Prove that if a^2+b^2 is a multiple of 3 then both a and b are multiples of 3.
problem
Days and dates

Age

11 to 14

Challenge level

Investigate how you can work out what day of the week your birthday will be on next year, and the year after...
problem
Old nuts

Age

16 to 18

Challenge level

In turn 4 people throw away three nuts from a pile and hide a quarter of the remainder finally leaving a multiple of 4 nuts. How many nuts were at the start?