Area - circles, sectors and segments
-

-
 problem
problemF'arc'tion
At the corner of the cube circular arcs are drawn and the area enclosed shaded. What fraction of the surface area of the cube is shaded? Try working out the answer without recourse to pencil and paper.
-
 problem
problemThe pillar of Chios
Semicircles are drawn on the sides of a rectangle. Prove that the sum of the areas of the four crescents is equal to the area of the rectangle.
-
 problem
problemFloored
A floor is covered by a tessellation of equilateral triangles, each having three equal arcs inside it. What proportion of the area of the tessellation is shaded? -
 problem
problemRound and round
Prove that the shaded area of the semicircle is equal to the area of the inner circle. -
 problem
problemSquare pegs
Which is a better fit, a square peg in a round hole or a round peg in a square hole? -
 problem
problemCompare areas
Which has the greatest area, a circle or a square, inscribed in an isosceles right angle triangle?
-
 problem
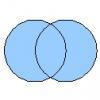
problemTwo circles
Draw two circles, each of radius 1 unit, so that each circle goes through the centre of the other one. What is the area of the overlap? -
 problem
problemGet cross
A white cross is placed symmetrically in a red disc with the central square of side length sqrt 2 and the arms of the cross of length 1 unit. What is the area of the disc still showing? -
 problem
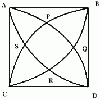
problemQuadarc
Given a square ABCD of sides 10 cm, and using the corners as centres, construct four quadrants with radius 10 cm each inside the square. The four arcs intersect at P, Q, R and S. Find the area enclosed by PQRS.
