2D shapes and their properties

problem
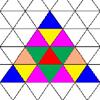
Where are they?

Age

7 to 11

Challenge level

Use the isometric grid paper to find the different polygons.
problem
Same shapes

Age

5 to 7

Challenge level

How can these shapes be cut in half to make two shapes the same shape and size? Can you find more than one way to do it?
problem
Count the trapeziums

Age

7 to 11

Challenge level

How many trapeziums, of various sizes, are hidden in this picture?
problem
Squaring the circle and circling the square

Age

14 to 16

Challenge level

If you continue the pattern, can you predict what each of the following areas will be? Try to explain your prediction.
problem
Playground snapshot

Age

7 to 14

Challenge level

The image in this problem is part of a piece of equipment found in the playground of a school. How would you describe it to someone over the phone?
problem
Squaring the circle

Age

11 to 14

Challenge level

Bluey-green, white and transparent squares with a few odd bits of shapes around the perimeter. But, how many squares are there of each type in the complete circle? Study the picture and make an estimate.
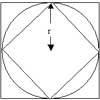
problem
Semi-square

Age

14 to 16

Challenge level

What is the ratio of the area of a square inscribed in a semicircle to the area of the square inscribed in the entire circle?
problem
Crescents and triangles

Age

14 to 16

Challenge level

Can you find a relationship between the area of the crescents and the area of the triangle?
problem
Approximating pi

Age

14 to 18

Challenge level

By inscribing a circle in a square and then a square in a circle find an approximation to pi. By using a hexagon, can you improve on the approximation?
problem
Blue and white

Age

11 to 14

Challenge level

Identical squares of side one unit contain some circles shaded blue. In which of the four examples is the shaded area greatest?