Volume and capacity

problem
Concrete calculation

Age

14 to 16

Challenge level

The builders have dug a hole in the ground to be filled with concrete for the foundations of our garage. How many cubic metres of ready-mix concrete should the builders order to fill this hole to make the concrete raft for the foundations?
problem
Plutarch's boxes

Age

11 to 14

Challenge level

According to Plutarch, the Greeks found all the rectangles with integer sides, whose areas are equal to their perimeters. Can you find them? What rectangular boxes, with integer sides, have their surface areas equal to their volumes?
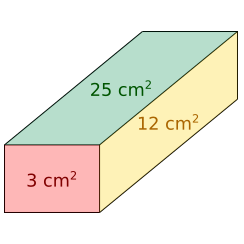
problem
Boxed in

Age

11 to 14

Challenge level

A box has faces with areas 3, 12 and 25 square centimetres. What is the volume of the box?
problem
Reach for polydron

Age

16 to 18

Challenge level

A tetrahedron has two identical equilateral triangles faces, of side length 1 unit. The other two faces are right angled isosceles triangles. Find the exact volume of the tetrahedron.
problem
2D-3D

Age

16 to 18

Challenge level

Two circles of equal size intersect and the centre of each circle is on the circumference of the other. What is the area of the intersection? Now imagine that the diagram represents two spheres of equal volume with the centre of each sphere on the surface of the other. What is the volume of intersection?
problem
Three cubes

Age

14 to 16

Challenge level

Can you work out the dimensions of the three cubes?
problem
Plane to see

Age

16 to 18

Challenge level

P is the midpoint of an edge of a cube and Q divides another edge in the ratio 1 to 4. Find the ratio of the volumes of the two pieces of the cube cut by a plane through PQ and a vertex.
problem
Double your popcorn, double your pleasure

Age

7 to 11

Challenge level

We went to the cinema and decided to buy some bags of popcorn so we asked about the prices. Investigate how much popcorn each bag holds so find out which we might have bought.
problem
Making cuboids

Age

7 to 11

Challenge level

Let's say you can only use two different lengths - 2 units and 4 units. Using just these 2 lengths as the edges how many different cuboids can you make?
problem
Making boxes

Age

7 to 11

Challenge level

Cut differently-sized square corners from a square piece of paper to make boxes without lids. Do they all have the same volume?