Conjecturing and generalising
-

-
 problem
problemStars
Can you work out what step size to take to ensure you visit all the dots on the circle? -
 problem
problemSquare it
Players take it in turns to choose a dot on the grid. The winner is the first to have four dots that can be joined to form a square.
-
 problem
problemHundred square
A hundred square has been printed on both sides of a piece of paper. What is on the back of 100? 58? 23? 19?
-
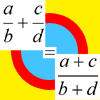 problem
problemCan it be?
When if ever do you get the right answer if you add two fractions by adding the numerators and adding the denominators? -
 problem
problemLots of lollies
Frances and Rishi were given a bag of lollies. They shared them out evenly and had one left over. How many lollies could there have been in the bag?
-
 problem
problemShare bears
Yasmin and Zach have some bears to share. Which numbers of bears can they share so that there are none left over? -

-
 problem
problemThe bridges of Konigsberg
Investigate how networks can be used to solve a problem for the 18th Century inhabitants of Konigsberg.
-
 problem
problemTourism
If you can copy a network without lifting your pen off the paper and without drawing any line twice, then it is traversable. Decide which of these diagrams are traversable.
