Tourism
If you can copy a network without lifting your pen off the paper and without drawing any line twice, then it is traversable. Decide which of these diagrams are traversable.
Problem

If you can copy a network
- without lifting your pen off the paper
- without drawing any line twice
then it is traversable.
Decide which of these diagrams are traversable.
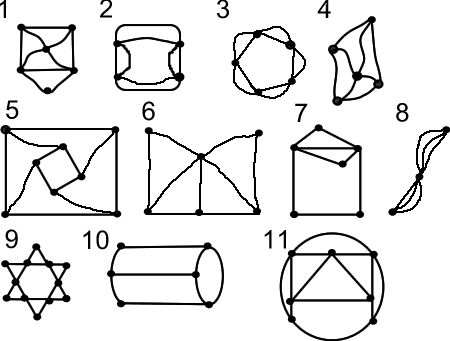
If you end up where you started when you draw a traversable diagram it is called a traversing circuit .
If you don't end up where you started when you draw a traversable diagram it is called a traversing path.
Can you give a set of criteria for determining whether a diagram is traversable by a path or a circuit or neither?
Getting Started
Have a look at the nodes (the points where the lines meet).
Student Solutions
We received a number of good responses to this problem.
Eva from Benenden School and Georgina both worked out that the traversable diagrams are: 1, 2, 3, 7, 8, 9, 11.
An anonymous contributor explained that:
"The rule to find whether a network is traversable or not is by looking at points called nodes.
Nodes are places where two or more lines meet. On these networks, the nodes are clearly shown by the black points in the diagrams.
Now you are probably wondering what this has to do with the network being traversable or not.
The node either would have an odd or even number of lines connected to it. Do not count the nodes with an even number of lines connected to it. Count the number of nodes with an odd number of lines connected to it.
If there are no odd nodes or if there are two odd nodes, that means that the network it traversable.
Networks with only two odd nodes are in a traversable path and networks with no odd nodes are in a traversable circuit."
Georgina added that:
"If a node has an even number of lines coming from it, this means that your pen will be able to both enter and leave that node. If not, the pen must start or finish at the node. As you can start and finish at only twonodes (start at one, finish at the other) any network with more than 2 odd nodes is not traversable.
If there are no odd nodes this means that the network can be drawn without taking your pen off the paper, starting and finishing at the same place."
Sarah from Colyton (and Andrei from School No. 205 in Bucharest) noticed that:
"For Curcuits:
Every point must have an even number of lines coming out of it."
Jonathan and Thomas from Heversham St Peters Primary School and Amy from Farlingaye High School listed their results as follows:
"1 is a traversable path
2 is a traversable circuit
3 is a traversable circuit
4 is not traversable
5 is not traversable
6 is not traversable
7 is a traversable circuit
8 is a traversable path
9 is a traversable circuit
10 is not traversable
11 is a traversable path"
Yanqing from LCC School summarised her findings as follows:
"Look at the nodes: if the node has an even number of lines coming from it, we call it an even node; if it has an odd number of lines, call it an odd node. If the network has NO odd nodes (all even nodes), it is a traversable circuit; if the network has 2 odd nodes, it is a traversable path; if the network has more than 2 odd nodes, it is not traversable."
Thank you all for your solutions.
Teachers' Resources
A good opportunity to develop geometric reasoning, test ideas and formulate hypotheses and generalisations.
