
Which is quicker?
Which is quicker, counting up to 30 in ones or counting up to 300 in tens? Why?

Which is quicker, counting up to 30 in ones or counting up to 300 in tens? Why?

I'm thinking of a number. My number is both a multiple of 5 and a multiple of 6. What could my number be?
A game for two people, or play online. Given a target number, say 23, and a range of numbers to choose from, say 1-4, players take it in turns to add to the running total to hit their target.
Ten cards are put into five envelopes so that there are two cards in each envelope. The sum of the numbers inside it is written on each envelope. What numbers could be inside the envelopes?
How many balls of modelling clay and how many straws does it take to make these skeleton shapes?

Can you make square numbers by adding two prime numbers together?
What is the greatest number of counters you can place on the grid below without four of them lying at the corners of a square?
Here are the six faces of a cube - in no particular order. Here are three views of the cube. Can you deduce where the faces are in relation to each other and record them on the net of this cube?
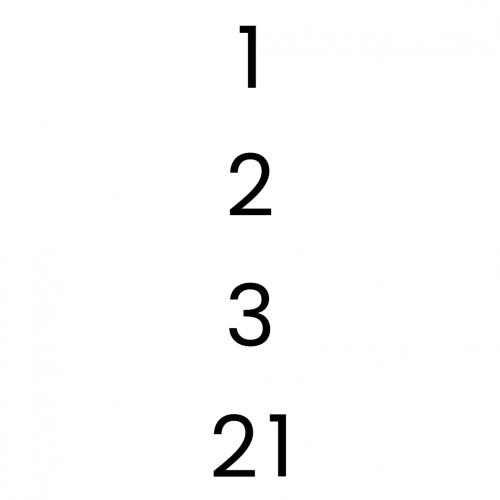
Arrange the four number cards on the grid, according to the rules, to make a diagonal, vertical or horizontal line.