3D shapes and their properties

There are 49 NRICH Mathematical resources connected to 3D shapes and their properties

problem

Guess what?

Age

7 to 11

Challenge level

Can you find out which 3D shape your partner has chosen before they work out your shape?

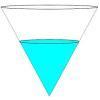problem

Fill me up too

Age

14 to 16

Challenge level

In Fill Me Up we invited you to sketch graphs as vessels are filled with water. Can you work out the equations of the graphs?

problem

Building blocks

Age

7 to 11

Challenge level

Here are some pictures of 3D shapes made from cubes. Can you make these shapes yourself?

problem

Shadow play

Age

5 to 7

Challenge level

Here are shadows of some 3D shapes. What shapes could have made them?

problem

Tet-trouble

Age

14 to 16

Challenge level

Is it possible to have a tetrahedron whose six edges have lengths 10, 20, 30, 40, 50 and 60 units?

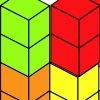problem

Hamiltonian cube

Age

11 to 16

Challenge level

Weekly Problem 36 - 2007
Find the length along the shortest path passing through certain points on the cube.

problem

Cola can

Age

11 to 14

Challenge level

An aluminium can contains 330 ml of cola. If the can's diameter is 6 cm what is the can's height?

problem

Nine colours

Age

11 to 16

Challenge level

Can you use small coloured cubes to make a 3 by 3 by 3 cube so that each face of the bigger cube contains one of each colour?

problem

Funnel

Age

14 to 16

Challenge level

A plastic funnel is used to pour liquids through narrow apertures. What shape funnel would use the least amount of plastic to manufacture for any specific volume ?

problem

Marbles in a box

Age

11 to 16

Challenge level

How many winning lines can you make in a three-dimensional version of noughts and crosses?

Or search by topic

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners

3D shapes and their properties

Guess what?

Fill me up too

Building blocks

Shadow play

Tet-trouble

Hamiltonian cube

Cola can

Nine colours

Funnel

Marbles in a box