Using National Young Mathematicians' Award tasks to develop problem-solving and group-working skills
NRICH has worked with Explore Learning since 2010 to hold the annual National Young Mathematicians' Award (NYMA). Each year, teams of four primary children from schools across the UK compete at regional events before five are selected to come to NRICH headquarters in Cambridge for the final. Throughout the competition, teams are judged on two things: how well they use their problem-solving skills to think like mathematicians and how well they work as a team (including their communication skills).
The purpose of this article is to support teachers in developing learners' problem-solving and group-working skills, using the NYMA tasks. The article is therefore split into two sections to address each of these aspects.
Developing key problem-solving skills using NYMA tasks
In our article Using NRICH Tasks to Develop Key Problem-Solving Skills, we suggest there are seven key problem-solving skills:
- Trial and improvement
- Working systematically (and remember there will be more that one way of doing this: not just the one that is obvious to you!)
- Pattern spotting
- Working backwards
- Reasoning logically
- Visualising
- Conjecturing
As learners progress towards a solution to the task they are working on, they may take the mathematics further (stage 3 of the problem-solving process) and two more problem-solving skills become important:
- Generalising
- Proving
This article will look at each problem-solving skill in turn and suggest NYMA tasks which offer a useful context in which to focus on that skill.
Trial and improvement
Trial and improvement involves trying something out, which will always give more insight into the context and therefore gives the solver a better idea of what to try next. Trial and improvement is often the start of working systematically. The NYMA task Display Boards particularly lends itself to a focus on trial and improvement. In order to fully understand the constraints of the challenges, it is almost necessary just to 'have a go' and then to tweak that original arrangement of boards to satisfy all the conditions. Similarly, Walking the Squares offers opportunities to use trial and improvement. In this case, the first path tried gives some insight into possible strategies for finding the longest path (which is the aim of the task). Altering the strategy slightly based on this first attempt will lead to new insight and so on, and so on ...
Working systematically
Working systematically can be thought of as working in a methodical and efficient way which could clearly show others that a pattern or system is being used. Systematic working is particularly important when a task entails finding all possibilities, which is the case in Make Those Bracelets and in Dice in a Corner. In both these tasks, following a system means that no solution is left out. Working systematically is also extremely useful in other types of problems. In Inky Cube, for example, trying out possible answers in a methodical way gives you insight into the situation which ultimately leads to a full solution. (More details about supporting learners to develop this skill can be found in our article, Encouraging Primary Children to Work Systematically.)
Pattern spotting
Being able to identify patterns can save time during the problem-solving process. However it is by then asking why the pattern occurs, and by trying to answer this question, that learners gain greater insight into mathematical structures and therefore deepen their conceptual understanding.
In Button-up Some More, learners might notice a relationship between, for example, the number of ways of doing up a coat with two buttons and the number of ways of doing up a coat with three buttons. Similarly, they may notice that the same relationship holds for three buttons compared with four buttons and for four buttons compared with 5 buttons etc. By trying to find out, and explain, why this pattern occurs, learners will come to a much deeper understanding of the structure of the task.
While working on Six Ten Total, there are several things that learners might notice. Perhaps they notice that the totals are always a multiple of four. Maybe they notice that the difference between the totals for inverses is always eight. Or perhaps they spot that the difference between consecutive totals is always 16. Whatever pattern they notice, they can then be encouraged to use the dice and/or diagrams combined with verbal/written explanation to explore why it occurs.
Working backwards
Starting from the end might sound counterintuitive, but it can be an efficient way of solving a problem. We are not sure that any of the NYMA tasks particularly lend themselves to working backwards, but do let us know if one of your learners uses this approach to tackle one of these activities. (For other tasks in which working backwards might be useful, see this list for lower primary and this for upper primary suggestions.)
Reasoning logically
At NRICH we believe that reasoning is fundamental to knowing and doing mathematics (see our Reasoning Feature for more information). Reasoning logically as a problem-solving skill is just a small part of reasoning and involves connecting information together in a sequence of steps. Six Numbered Cubes is a wonderful context in which to challenge learners to reason logically. In each part of the challenge, they can be asked to explain how they know they have the highest/lowest total. This will involve considering the number of visible faces of each cube and the particular numbers showing on each face. In Curious Number, learners are encouraged to use their knowledge of divisibility to reason why the digits can/cannot be in that particular order.
Visualising
Picturing what is happening in your mind's eye, or imagining what is happening or what might happen, is a skill which is perhaps not talked about very much in the classroom. Specifically drawing attention to instances when it might be used will raise learners' awareness of this skill so that they might use choose to use it themselves. The task Building with Rods offers a good context in which to discuss visualising as some learners might well be picturing a new solution in their head before physically making it. At a more sophisticated level, some might well be 'seeing' a process of working systematically to find all the possible solutions to this task, for example by moving particular cubes in turn, in a methodical way. In Cover the Tray, learners might get a feel for the way in which the size of the cut-out square affects the dimensions of the tray that can be covered, by beginning to visualise the wrapping process.
Conjecturing
Conjecturing, or asking "What if..?" questions, is an important problem-solving skill. Knowing what to ask means that a learner understands something about the structure of the problem. So It's Times! is an ideal context in which to give learners experience of conjecturing as the task focuses on finding highest and lowest totals without actually doing all the calculations. You can encourage learners to 'play around' with the various arrangements of the square to get some insight into the structure (trial and improvement) before asking them to express their conjecture. Of course, a conjecture then needs to be shown to be true or false (as opposed to a scientific hypothesis which can only ever be refined as further evidence becomes available), which leads us on to ...
Generalising
Being able to see similarities and differences gives you insight into what will always be true in that situation and this means you are starting to generalise. Six Numbered Cubes is a great context in which to develop learners' ability to generalise. Building on the logical reasoning described above, the generalisation is rooted in the way in which a cube's position affects how many of its faces are visible and therefore the possible total. Having reasoned why the '6' cube should go in a particular place to make the highest total in the staircase arrangement, learners can use this to generalise about the position of the '6' cube when looking for the highest total, no matter what the arrangement of cubes.
Proving
We suggest that being able to prove is the highest step on the reasoning journey (see our Reasoning Feature and particularly our article Reasoning: the Journey from Novice to Expert), following on from convincing and justifying. The key to proof is being able to articulate a watertight argument. In our Mastering Mathematics: Developing Generalising and Proof Feature, we discuss generalising and proof in much more detail, and we suggest that there are several types:
- Proof by exhaustion - this depends on there being a small number of results so that it is manageable to find all possibilities; it is about working systematically.
- Proof by counter example - an example that doesn't work disproves the conjucture.
- Proof by contradiction - we assume that what we want to prove is not true, and then show that the consequences of this are not possible.
- Proof by logical argument - the chain of reasoning is complete, with no room for ambiguity and no missing steps.
- Generic proof - a carefully selected example enables anyone to see, in that example, the general structure.
Six Ten Total offers a great opportunity for proof and is an example of proof by logical argument. Learners take sixteen dice - six of one colour and ten of another - laid out like this (here we have chosen red and blue dice):
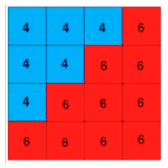
All the blue and all the red dice need to have the same number on the top and there always needs to be six of one colour and then ten of the other. The red dice have to display a different number to the blue and there is always a difference of 2 between the numbers on the blue and red dice. The challenge is for learners to find the possible arrangements when they choose your own numbers for the dice, using the rules above. They are then asked to consider what they notice and try to prove what they notice.
One of the things learners may notice as they tackle this task, is that the total of the dice is always a multiple of 4. This can be proven algebraically, for example:
Then (a+2) is the value on the red dice (in the above case 6).
Then the total of all dice = 6 lots of a + 10 lots of (a+2)
Total = 6a + 10(a + 2)
Multiply out the bracket
Total = 6a +10a + 20
Collecting like terms together
Total = 16a + 20
Both these terms are multiples of 4
So total = 4(4a +5).
So, whatever the value of a, the total will always be a multiple of 4.
However the same result can be proven visually, for example:
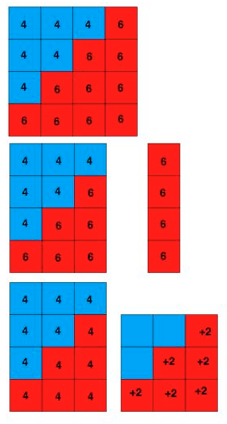
In the top picture we have the original situation.
1. We split off the last column so that we have twelve in a rectangle and four in a column.
2. We look at the rectangle and realise that we can split this up into twelve of the same number plus six '+2's as the number on the red dice is two more than the number on the blue dice. This will work the other way round if the number on the blue dice is two more than the number on the red dice.
3. So we can rearrange the dice into three blocks, each of which is a multiple of four:
• a column of four of the larger number
• a rectangle of twelve of the smaller number
• a set of six +2s which is twelve
These blocks are multiples of four regardless of the numbers on the red and blue dice so this is true for all solutions.
The beauty of a visual proof is that it can sometimes be accessible to younger learners in a way that algebra is not.
Developing group-working skills using NYMA tasks
In her article Group-worthy Tasks and Their Potential to Support Children to Develop Independent Problem-solving Skills, Jennie Pennant outlines the benefits of working on mathematics as a group, arguing that group work also supports children's individual problem-solving abilities. It is essential, therefore, that we help children get better at working on mathematics in groups. We suggest learners need to acquire a set of skills which relate to working collaboratively:
- Listening to each other
- Finding out what others think
- Reflecting on and making use of what has been said
- Allowing everyone to contribute
- Helping each other
- Supporting each other to stay focussed on the task in hand
- Sharing knowledge and reasoning
- Giving reasons for ideas
- Being concise
- Pulling ideas together
- Finding out if the group is ready to make a decision.
For the purposes of this article, we will discuss these skills in four sets and suggest NYMA tasks which will offer opportunities to focus on the particular skills in each set.
Listening to each other
Finding out what others think
Reflecting on and making use of what has been said
Allowing everyone to contribute
The interesting element here is valuing the collective wisdom of the group and knowing that together we can come up with a good solution. Children often need support to make the journey from 'my way is best' to 'together we can build some helpful ideas and ways to solve this challenge'.
In the NYMA final we see this working well when children take time to stop what they are doing and listen to what another team member is saying. The effective team members take time to really understand what their fellow team member is saying. We hear them saying things such as, "tell me again how that works", "I got lost when you said ...", "I think what you mean is ...". We also notice the way in which they engage with each other - turning, looking and nodding to show they are listening.
While working on Ribbon Squares, one learner found that some of the squares could be tilted and had to work hard to explain what that meant. This certainly required the rest of the team to listen carefully and value what was being said. The following extract from a Paddington Bear story in which Paddington is answering questions for a TV quiz show also serves to remind us how vital it is that learners really work hard to understand what each other is thinking:
"For fifty pounds here is question number two, and it's a two part question. Listen carefully."
"If," he said, "you had a piece of wood eight feet long and you cut it in half, and if you cut the two pieces you then have in half again, and if you then cut all the pieces into half again how many pieces would you have?"
"Eight," said Paddington promptly.
"Very good, bear," said Ronnie Playfair approvingly.
"Here is the second part of the question. How long will each of the pieces be?"
"Eight feet," said Paddington almost before the Master of Ceremonies had time to start the clock. "Eight feet?" repeated Ronnie Playfair. "You're sure you won't change your mind?"
"No, thank you, Mr Playfair," said Paddington firmly.
"In that case ... I must ask you for the £5 back, the answer is one foot."
"Oh no, Mr Playfair," said Paddington politely, "I'm sure that's right for your piece of wood but I cut mine the other way."
"But if you're asked to cut a plank of wood in half," stuttered Ronnie Playfair, "you cut it across the middle not down the middle. It stands to reason."
"Not if you're a bear," said Paddington, remembering his efforts at carpentry in the past.
"If you're a bear it's safer to cut down the middle."
Each year during the NYMA final, we observe some teams stopping to orchestrate a 'pooling of ideas' where they share their initial thoughts on a problem and see how they can move forward in the light of everyone's ideas. Sometimes this sharing can generate a light bulb moment where someone in the team exclaims, "hey, what about trying this ...?".
Our Developing Group-working Skills Feature gives ideas on how you can support children to develop this way of working. Activities like Fraction Match help children to develop the idea of everyone contributing and needing to take everyone's thinking into account. You may like to try out the idea of each member of the group taking on a distinct role and therefore needing to contribute. You can find details of this in the Teachers' Resources section of the task Planning a School Trip.
Helping each other
Supporting each other to stay focussed on the task in hand
Here again, children need to learn the benefits of working together which may not come naturally. All too often one child can 'run off' with an idea, work on it and then declare they have 'done it'. Others then often feel very discouraged and give up even if they were on the way to a solution. In the NYMA final 2014 we saw a child help another who was struggling to add up a string of numbers. He knew how to solve that part of the problem yet was having trouble with the arithmetic. What was powerful about the way in which the other team member helped him was that she explained how she would do it and helped him find the 'bonds to ten' and the multiples rather than 'just doing it'. This is the way that we need to encourage children to help each other, and to do this they may well need it modeled by us as teachers and Teaching Assistants.
In the NYMA final 2013, we noticed one child in the group became disengaged after a while. Another child in the team stopped to ask him what the problem was. It turned out he had tried out an idea, got stuck and was now rather at a loss to know what else to try. Together they were able to share their thinking and move forward. The boy was 'on task' again and contributing to the group.
While working on Dice in a Corner, some children helped others in the team physically make a 'corner' for the dice to stand in after they found they had been adding up too many dice faces.
Staying focused on the task in hand proved tricky for some learners when they tackled Make Those Bracelets as they were distracted by wanting to create 'nice-looking' patterns. Other members of the team had to remind them of the mathematical challenge at hand!
Returning to our Developing Group-working Skills Feature you may like to try an activity such as Counters in the Middle which uses the premise that pulling everyone's ideas together is important in producing the final product. You may also like to model in class the conversation that could take place when you notice that someone is stuck or 'off task', drawing attention to useful statements such as, "tell me what you've done so far" and "tell me where you are stuck".
Sharing knowledge and reasoning
Giving reasons for ideas
Being concise
Both dice activities, Dice in a Corner and Dice Train, provoked some learners to instantly recall that opposite faces of a dice add to 7, which helped the team/s with subsequent reasoning.
In the 2014 NYMA final we heard some great reasoning from the children as they worked on Six Numbered Cubes. One team member offered a very clear explanation for the final part of the problem in which the challenge was to prove by logical reasoning that whatever the order of cubes you cannot produce a total of 80.
Children often need help to get to this stage of being able to express their thinking clearly and concisely. When children first start explaining their reasoning it can be very long-winded and lack accurate mathematical vocabulary. This is a great start, yet we need to help them refine their sentence structure and introduce appropriate mathematical vocabulary. Strategies that we use in Literacy lessons can be very helpful here. We also need to help them give reasons for their thinking and this needs modeling for them. You can read more about how you can support children to develop their reasoning in our article Reasoning: The Journey from Novice to Expert.
You can see here in the NYMA final 2013, Cover the Tray, the team can very clearly explain their thinking in logical stages yet still need to give reasons for their choices:

Pulling ideas together
Finding out if the group is ready to make a decision
In the NYMA finals we see this working very well when the children are coming to the end of one of the challenges. Before they are able to move onto the next challenge, they need to be able to, as a group, agree and express their solution to the challenge they have been working on. Often we see a 'chair' emerge who leads this discussion and helps the group to gather all their thinking together. Sometimes we notice that the team has allocated different aspects of the challenge to different members of the team and they need to pool what they have discovered. We saw this clearly in the 2014 final where some children worked on different arrangements of the cubes and they then shared their findings. This was an effective way of using the time and helping them to explore more about the problem more quickly.
Conclusion
Being able to work well on mathematics in a group will help children get better at individual problem solving. Therefore supporting children in developing both group-working skills and key problem-solving skills will be beneficial to their development as mathematicians. In this article we have drawn attention to a range of activities, all created for the National Young Mathematicians' Award, which offer interesting contexts in which to work on these skills. Focusing on one key element for a sustained period can be a good way of giving children a breadth of experience so that they have opportunities to apply a newly-acquired skill to other unfamiliar contexts.
The following features and articles on NRICH offer further guidance:
Problem Solving Feature, including the article Using NRICH Tasks to Develop Key Problem-solving Skills
Developing Group-working Skills Feature, including the article Group-worthy Tasks and Their Potential to Support Children to Develop Independent Problem-solving Skills and the article Developing Good Team-working Skills in Primary Schools
Finally, if you are keen to find out more about future National Young Mathematicians' Awards, which take place in November/December of each academic year, go to http://www.explorelearning.co.uk/
Here is a PDF version of this article.
