Working systematically

problem
Sums and differences 2

Age

7 to 11

Challenge level

Find the sum and difference between a pair of two-digit numbers. Now find the sum and difference between the sum and difference! What happens?
problem
Sums and differences 1

Age

7 to 11

Challenge level

This challenge focuses on finding the sum and difference of pairs of two-digit numbers.
problem
Unit differences

Age

5 to 7

Challenge level

This challenge is about finding the difference between numbers which have the same tens digit.
problem
Picturing the world

Age

14 to 16

Challenge level

How can we make sense of national and global statistics involving very large numbers?
problem
Round the dice decimals 1

Age

7 to 11

Challenge level

Use two dice to generate two numbers with one decimal place. What happens when you round these numbers to the nearest whole number?
problem
Round the three dice

Age

7 to 11

Challenge level

What happens when you round these three-digit numbers to the nearest 100?
problem
Round the dice decimals 2

Age

7 to 11

Challenge level

What happens when you round these numbers to the nearest whole number?
problem
Round the four dice

Age

7 to 11

Challenge level

This activity involves rounding four-digit numbers to the nearest thousand.
problem
Shape draw

Age

7 to 11

Challenge level

Use the information on these cards to draw the shape that is being described.
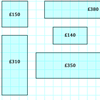
problem
Through the window

Age

7 to 11

Challenge level

My local DIY shop calculates the price of its windows according to the area of glass and the length of frame used. Can you work out how they arrived at these prices?