Tessellations
-
-
problemSemi-regular tessellations
Age11 to 16Challenge level
Semi-regular tessellations combine two or more different regular polygons to fill the plane. Can you find all the semi-regular tessellations?
-
-
problemNapoleon's theorem
Age14 to 18Challenge level
Triangle ABC has equilateral triangles drawn on its edges. Points P, Q and R are the centres of the equilateral triangles. What can you prove about the triangle PQR? -
problemShapely tiling
Age7 to 11Challenge level
Use the interactivity to make this Islamic star and cross design. Can you produce a tessellation of regular octagons with two different types of triangle? -
problemBow tie
Age11 to 14Challenge level
Show how this pentagonal tile can be used to tile the plane and describe the transformations which map this pentagon to its images in the tiling. -
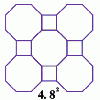
problemSchlafli tessellations
Age11 to 18Challenge level
are somewhat mundane they do pose a demanding challenge in terms of 'elegant' LOGO procedures. This problem considers the eight semi-regular tessellations which pose a demanding challenge in terms of 'elegant' LOGO procedures.
-
problemLOGO challenge - tilings
Age11 to 16Challenge level
Three examples of particular tilings of the plane, namely those where - NOT all corners of the tile are vertices of the tiling. You might like to produce an elegant program to replicate one or all of these.
-
problemLOGO challenge - triangles-squares-stars
Age11 to 16Challenge level
Can you recreate these designs? What are the basic units? What movement is required between each unit? Some elegant use of procedures will help - variables not essential.
-
problemLOGO challenge 5 - patch
Age11 to 16Challenge level
Using LOGO, can you construct elegant procedures that will draw this family of 'floor coverings'?