Factors and multiples game

Age

7 to 16

Challenge level

Primary

Number - Multiplication, Division and Ratio

Properties of Numbers: Multiples, Factors, Primes, Square and Cube Numbers

Secondary

NUMBER

Factors, Multiples & Primes

Exploring and noticing Working systematically Conjecturing and generalising Visualising and representing Reasoning, convincing and proving

Being curious Being resourceful Being resilient Being collaborative

Factors and Multiples printable worksheet - game

Factors and Multiples printable worksheet - puzzle

On this resources page there are printable 1-100 square grids.

You may like to have a go at Dozens before having a go at this challenge.

This is a game for two players.

The first player chooses a positive even number that is less than 50, and crosses it out on the grid.

The second player chooses a number to cross out. The number must be a factor or multiple of the first number.

Players continue to take it in turns to cross out numbers, at each stage choosing a number that is a factor or multiple of the number just crossed out by the other player.

The first person who is unable to cross out a number loses.

Play a few times to get a feel for the game.

Do you have any winning strategies?

Here is an interactive version of the game in which you drag the numbers from the left hand grid and drop them on the right hand grid. Alternatively, click on a number in the left hand grid and it will transport to the earliest empty location in the right hand grid. You can rearrange the numbers in the right hand grid by dragging and dropping them in position. The integer in the top right hand corner grows with the number of factors/multiples you have in a row.

Tablet version Install in home page

Alternatively, you can print out some 1-100 square grids.

Now for a collaborative challenge...

Rather than playing against a partner, it's time to work together with them to find the longest sequence of numbers that can be crossed out.

Can you cross out more than half the numbers? How are you approaching this challenge to try and make your sequence as long as possible?

You might find this sheet useful, which includes the instructions for this collaborative task with a 100 square.

You may now enjoy having a go at Xavi's T-shirt.

This activity featured in an NRICH video in June 2020 and an NRICH student webinar in November 2020.

Many thanks to everyone who shared their solutions with our team. We were very impressed by your resilience and perseverence to produce the chains that you submitted to NRICH. Several of you sent in more than one solution, substituting an earlier chain with an even longer one after spending more time on the challenge. Well done to you all!

We've published below a selection of increasingly long chains which have a screenshot showing the full chain, or a list of its factors and multiples. We hope this list inspires you to keep working on your own solutions and perhaps consider the length of the longest possible chain, and the reasons for your answer.

Let's begin with Montsaye Community College in Northamptonshire where their students took on the challenge of generating the longest possible chain.

Gabrielle and Lauren reached a total of 50 numbers:

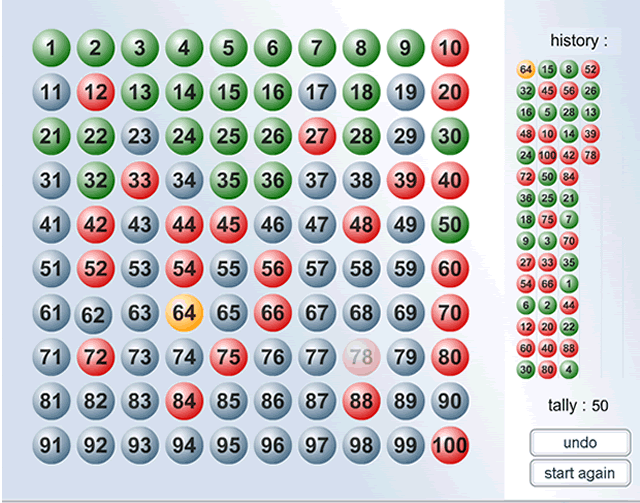
Aravindan, from GHS in India, shared this chain of 51 numbers:

Makenzie, from Mountsaye Community College, managed to improve on this with a chain of 55 numbers:

Sophie and Tasmin, also from Mountsaye, managed to improve on that with this chain of 56 numbers:

Then, Gabrielle and Lauren managed to improve on their earlier effort with this new chain featuring 59 numbers:

Aravindan improved on his previous effort to produce this chain of 60 numbers:

Abigail from Ridgewood School managed a chain of 61 numbers:

Alfie, Manuel, Jack and Emilio from Newhall School in Chelmsford, Essex, worked as a team to also produce a chain of 61, and Harry from Farmborough Church Primary School created the same chain:

A.H. from Manorfield Primary School has improved on this by finding a chain of 63 numbers:

90-9-99-33-66-11-44-22-88-8-80-40-10-100-20-60-30-15-75-25-50-5-35-7-70-14-56-28-84-21-42-6-78-39-13-26-52-4-68-34-17-51-1-46-92-23-69-3-57-19-38-76-2-24-72-18-36-12-48-16-24-32-96

This solution came in from Ralph and Max at the Institut International de Lancy in Switzerland. Their teacher said they did not use a computer or calculator. They made a chain of 65, which seems to be a very popular answer.

Evie from Deansfield Primary School created a chain of 65 numbers:

James from Ridgewood School went one better with this chain of 66 numbers:

Linda from Bohunt School used 68 numbers:

A group of Year 9 students from The Perse School for Girls in Cambridge worked together and managed an even longer chain of 71 numbers:

And Claire, of Blackheath High School in London, has managed to improve on that with this chain of 73 numbers:

Jacky, from Princethorpe College in Rugby, has managed to go one better with a chain of 74:

Jesse from Moriah College in Sydney, Australia also managed a chain of 74 numbers:

Izaak, from Hills Rd VI Form College, used some computer programming techniques to try to make the longest chain he could. Below is his 76 long chain. If you want to read about his program and see his code, he has shared it on Github.

We've also received a few chains using 77 numbers!

Millie from Hursthead Junior School in the UK sent in this solution:

Well done Millie! Jakob from Haberdashers Adams School in the UK also found the same solution.

We received a different chain of 77 numbers from Michelle at The Coopers' Company and Coborn School in the UK:

Ahaan from Newcastle Under Lyme School found the same chain, and Sarisha from Newcastle Under Lyme School found this chain of 77 numbers:

Can you see how this is related to Michelle's chain above?

Miraya from Heckmondwike Grammar School shared these strategies for developing longer and longer chains:

First of all not use the numbers one to ten since they are the common multiples or factors of any given number between one to hundred. Next , the other thing you have to remember is to try not to use prime numbers as the prime numbers have factors that are only 1 you can use multiples but they will not last very long. Try to find out the common multiple factors as well. Also, try to use the bigger numbers since they will have the most factors. Finally , try to use the common numbers with the most multiples under 100 for example 10 or 11 since they have multiples in every ten numbers.

Well done to all of you, and to those of you who found chains of 77, congratulations, you have found the longest chain.

Thank you to Josh Tutin who sent us a link to The On-Line Encyclopedia of Integer Sequences, and the table below, which lists the longest possible chains using the numbers from 1 to n, where n is smaller than 200. Click to see the table. Can you produce some of these chains?

n	Longest chain
1	1
2	2
3	3
4	4
5	4
6	6
7	6
8	7
9	8
10	9
11	9
12	11
13	11
14	12
15	13
16	14
17	14
18	16
19	16
20	17
21	18
22	19
23	19
24	21
25	21
26	22
27	23
28	24
29	24
30	26
31	26
32	27
33	28
34	28
35	29
36	30
37	30
38	30
39	31
40	32
41	32
42	34
43	34
44	36
45	37
46	37
47	37
48	39
49	39
50	41
51	42
52	43
53	43
54	44
55	45
56	46
57	47
58	47
59	47
60	49
61	49
62	49
63	50
64	51
65	51
66	53
67	53
68	54
69	55
70	57
71	57
72	58
73	58
74	58
75	59
76	60
77	61
78	63
79	63
80	64
81	65
82	65
83	65
84	66
85	66
86	66
87	67
88	69
89	69
90	70
91	71
92	72
93	73
94	73
95	73
96	74
97	74
98	75
99	76
100	77
101	77
102	79
103	79
104	81
105	82
106	82
107	82
108	83
109	83
110	85
111	86
112	87
113	87
114	89
115	89
116	90
117	91
118	91
119	92
120	93
121	93
122	93
123	94
124	95
125	95
126	96
127	96
128	97
129	97
130	99
131	99
132	100
133	100
134	100
135	101
136	103
137	103
138	105
139	105
140	106
141	106
142	106
143	106
144	107
145	108
146	108
147	110
148	111
149	111
150	112
151	112
152	113
153	115
154	116
155	116
156	117
157	117
158	117
159	117
160	118
161	119
162	120
163	120
164	121
165	123
166	123
167	123
168	124
169	124
170	126
171	127
172	128
173	128
174	131
175	132
176	133
177	133
178	133
179	133
180	134
181	134
182	135
183	135
184	137
185	137
186	139
187	139
188	140
189	141
190	142
191	142
192	143
193	143
194	143
195	144
196	145
197	145
198	147
199	147
200	148

There are many different possible longest chains - at the OEIS you can see the 12 different possible chains, of length 11 (the longest chain - see below), which use numbers from 1 to 13.

Josh then investigated numbers which should not go in chains, and exploited these to develop some winning strategies for playing the game. Josh also created some longest-possible chains using numbers 1 to n, for several values of n. Click here to see Josh's work, and here for a later addition to Josh's research.

Why play this game?

This game can replace standard practice exercises on finding factors and multiples. In order to play strategically, learners think about numbers in terms of their factors, utilising primes and squares to develop winning moves. The switch from a competitive to a collaborative game gives an opportunity for learners to work together and try to find the longest chain, a problem that they could keep coming back to over and over again!

The game also offers the chance to focus on any of the five key ingredients that characterise successful mathematicians. The collaborative version lends itself particularly to fostering a positive attitude to mathematics as learners' resilience may be tested!

Possible approach

The following printable worksheets may be useful: Factors and Multiples Game, Factors and Multiples Puzzle, resources page for 1-100 square grids.

This activity featured in an NRICH video for students in June 2020 and an NRICH student webinar in November 2020. It also featured in an NRICH webinar for teachers in June 2020.

Dozens may be a good problem for students to tackle, before embarking on this challenge.

Play the game as a class, on the board, to introduce the rules, perhaps dedicating the last twenty minutes of each lesson for a week, to playing in pairs. When pupils have finished a game, they could play the next game against someone they've not yet played. At the end of each game, ask pairs to analyse why the last few moves led to its end - working out better moves that could have been made.

To start with you could choose not to mention the initial rule that restricts the starting number to a positive even number that is less than 50. When pupils discover that the first player can win after just three numbers have been crossed, discuss the need to restrict the initial number to an even number smaller than 50.

As learners are playing the game, listen out for those who are considering the probable next few moves when placing a counter/crossing out a number. Game strategies form a natural context for developing deductive logic. You may want to invite a pair of pupils to play against another pair. This gives a 'reason to reason' as each player will need to justify their choice of next move in order for their partner to agree it is the best way to proceed.

The collaborative challenge could run for an extended period: the longest sequence can be displayed on a wall or noticeboard and pupils can be challenged to improve on it. Any improved sequences can be added (perhaps once they have been checked by someone else!). You could then set aside some time in a future lesson to discuss the longest sequence. Does the class think that it is possible to create a longer one? Why or why not?

Key questions

Do you have any winning strategies?

Are there any numbers you should try to avoid?

Possible support

Use a smaller number board, eg 1-50 (or 1-49 in a square). Here is a large 1-50 grid and here is a sheet of smaller grids which could be given to pupils. This makes the mental calculations much easier, without watering down the mathematics. The lesson could focus on accuracy of calculation - with teacher interventions to get pupils sharing their mental strategies.

Handouts for teachers are available here (Word document, pdf), with the problem on one side and the notes on the other.

Possible extension

For the collaborative version of the game, ask pupils to explain why their choice of numbers is good.

Xavi's T-shirt is a suitable follow-up problem

Or search by topic

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners