Xavi's T-shirt
How much can you read into a T-shirt?
Problem

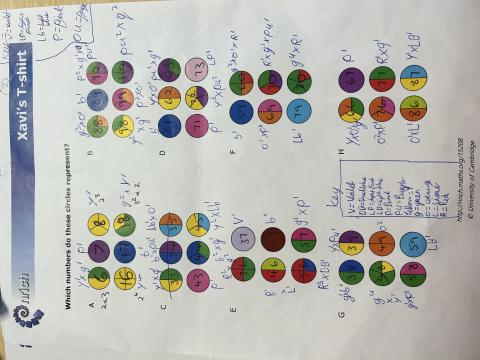
Xavi, a friend of NRICH, was showing off his T-shirt, with circles representing the numbers from 2 to 100.
We thought we could turn it into a problem...
Take a look at the T-shirt image (using different colours), or at the T-shirt image with some of the numbers identified.
You could also download the printable sheet without numbers or the printable sheet with some of the numbers.
What do you notice? Can you explain what you notice?
Here are some questions you may like to consider:
What is special about the circles that are not split up?
Can you explain what is happening in the top row?
What do you notice about the colours in the fifth column?
What is happening in the far right column?
Take a look at the colours of the first two circles (2 and 3).
What is special about the circles/numbers that have these two colours?
What is special about the circles/numbers that have only these two colours?
What do you notice about square numbers?
Why do multiples of 11 appear on a diagonal line?
Why do multiples of 9 appear on a diagonal line?
Look at the circle that represents 8. The three parts are all the same colour. How many other circles/numbers will also be split into three identical colours?
On the bottom row, 93, 94 and 95 appear as three consecutive circles/numbers, each split into two, and no colour is repeated.
Can you find a similar set of four consecutive circles/numbers where no colour is repeated? If not, why not?
What other patterns can you see? Can you explain why they occur?
Imagine you are wanting to create your own version of this T-shirt.
Before worrying about the colours, you need to decide how the circles are going to be split...
Using either a sheet of blank circles, a sheet with the prime numbers identified, or a sheet with all the numbers identified, sketch how you would need to split the circles. Start at the top and work your way downwards. Stop when you get to 40.
Can you anticipate how many circles in the whole T-shirt will be split into six sections?
You should have found only two:
2x2x2x2x2x2 = $2^6$ = 64
2x2x2x2x2x3 = $2^5 \times 3$ = 96
Can you anticipate how many circles will be split into five sections?
You should have found four:
2x2x2x2x2 = $2^5$ = 32
2x2x2x2x3 = $2^4 \times 3$ = 48
2x2x2x2x5 = $2^4 \times 5$ = 80
2x2x2x3x3 = $2^3 \times 3^2$ = 72
Can you anticipate how many circles will be split into four sections?
You should have found twelve.
You may want to continue creating your version of the T-shirt, but we think that the insights you have gained will prepare you for some more interesting challenges...
If we 'zoom in' on part of the T-shirt, do six circles offer you sufficient clues to figure out where you are on the T-shirt?
Can you figure out where you are on the T-shirt with fewer clues...?
Either take a look at these eight challenges or have a go at the T-shirt interactivity below, which gives you feedback.
Click on the purple cog (Settings) and try each of the games in turn.
In each case, you will be offered an image of part of the T-shirt.
Can you figure out which numbers the circles represent?
Imagine Xavi also had a scarf, with all the numbers from 2 to 100 in a straight line.
Either take a look at these ten scarf challenges or have a go at the T-shirt interactivity below.
Click on the purple cog (Settings) and try each of the games in turn.
In each case, you will be offered an image of part of the scarf.
Can you figure out which numbers the circles represent?
When there is more than one possibility, the challenge is to find the sequence which contains the lowest possible number for the selected circle (indicated by an arrow when you click 'I know what the circles stand for').
We hope many of you will share screenshots of the challenges you have worked on, along with your reasoning of how you used the 'clues' to solve the problem.
Getting Started
Notice that when you hover over each of the circles of the full T-shirt in the interactivity, you are told which number it represents.
6 = 2 x 3
12 = 2 x 2 x 3
How is this reflected in the circles which represent 6 and 12?
Student Solutions
We received a large number of solutions to this problem, and there was a lot of variety in the ages and approaches that we saw. Well done to everyone who worked on this problem and submitted a solution.
Lots of people began by exploring the numbers in the T-shirt. Bonnie identified the missing numbers on the T-shirt and shaded some blank circles with numbers on. Click here to see Bonnie's work.
Billy from Tanglin Trust School in Singapore, Ahsan from Pristine International School in the UAE, Noah and Max and Sam from The Cavendish School, Clancy from Kingsmead School, Dia, Shaunak, Nishika, Ruhaan, Neelmadhav, Kivisha, Deethya, Mithravinda, Abheer, Udit, Uday, Vidyut, Adhrit, Arjun and Aditya from Ganit Kreeda in India, Isabelle and Jessica (and someone else) from Alderman Payne Primary School, Bob from Evandale Primary in Tasmania (Australia), Zac who is educated at home, Millie from Oakmoor, Humphrey from Twyford, Lily, Jenny from Lydgate Junior School, Evani from High March, three students from Wembrook primary School and Joshua, all in the UK, explained what is special about the circles that are not split up. Noah and Max wrote:
The whole circles are prime numbers and that explains why we don’t have one on the grid
Humphrey explained about 1 in a bit more detail:
1 is neither prime or composite.
Billy, Joshua, Ahsan, the students from Ganit Kreeda, Zac, Millie, Lily, Jenny, Evani and student GD from Wembrook used this to explain what is happening in the top row. This is Lily's answer:
The different sections in each circle are factors of that number. E.g. 6 is made up of two sections of different colours which shows 2x3, and 10 has factors of 2 and 5.
Bob explained the reasoning to get there:
It goes 2, 3 (because it said it goes 2 to 100) which are prime numbers, and then the next one in order is split. It is red then red and I multiplied 2 and 2 (which is 4) because it had 2 reds, but I also realised I could’ve added. So I went to the fifth which is yellow but it wasn’t split so I went to the next one and saw green and red. So I added that which was 5, but it couldn’t have been five because the yellow one was 5. So I multiplied it and it equalled 6 because 2 times 3 equals 6 and it was 6th in line so I knew I was on the right track.
Jenny explained why the first row is special:
On the top row, the circles that are split up only include the colors of the prime colors on the row (2,3,5,7), which makes the top row unique (the other rows have the colors of 2,3,5 in them)
Ahsan, the students from Ganit Kreeda, Zac, Sam, Isabelle, Jessica, Lily, Evani, Dhruv and the students from Wembrook Primary School described what they noticed about the fifth column and the far right column. Dhruv wrote about the colours:
They all have yellow, and [in the final column] they all have red and yellow.
Zac described how this is related to the numbers in those columns:
The numbers in the fifth column all have yellow in them because they are all multiples of 5. The other colours represent the number(s) that you have to multiply 5 by to get a number that ends in 5 because they are all in the 5 times table.
The number at the top of the far right hand column is 10. This is represented by red & yellow because they are the numbers 2 & 5 because they multiply to make 10. 10 can’t have its own number because it isn’t a prime. They all have at least one red & yellow segment to represent the number 10 (2 $\times$ 5) and a variation of other segments to equal the next number in the ten times table. For example, 70 has three segments, red, yellow & blue this represents 2 $\times$ 5 $\times$ 7=70.
Saad from Pristine International School, Clancy, the students from Ganit Kreeda, Ahsan, Sam, Isabelle, Lily, Jenny, Evani, Dhruv and student TB from Wembrook described the circles/numbers which have the colours of the first two circles (2 and 3). Clancy described some examples:
For example three is a prime number and nine will have a line down the middle as it is a multiple of three and not a prime number. The two halves will be colour-coded the same colour as they are representing three, as it is a factor of nine. This works the same as any other number. Twenty-four will have the colours of two, two, two and three. Twelve will have the colours of two, two and three.
Shaunak from Ganit Kreeda explained what is special about these circles:
6 multiplied by a number which is not 2, 3 or a multiple of 6 will give a circle which has another colour excluding red and green.
So, the special thing about these circles/numbers is if they are 6 $\times$ 2 or 3 or a number whose prime factors are 2 and/or 3 only. E.g. 6, 12,18, 24, 36, 48…
TB from Wembrook Primary School commented:
These two colours appear a lot as most numbers are either in the two or three times table.
Saad, Ahsan, the students from Ganit Kreeda, Noah and Max, Sam, Isabelle, Millie, Lily, Jenny, Evani and Dhruv explained why multiples of 11 and 9 appear on diagonal lines. The students from Ganit Kreeda wrote:
11 is 10 + 1 and adding 10 to a number on this board will place one on the same column, but on the next row. Adding 1 will put one in the next column but on the same row. So, adding 11 to a number will place one 1 row below and one column right. Doing this repeatedly, one sees that one gets a diagonal line toward the bottom right.
9 is 10 $-$ 1 and adding 10 to a number on this board will place one on the same column, but on the next row. Subtracting 1 will put one on the previous column but on the same row. So, adding 9 to a number will place one 1 row below and one column left. Doing this repeatedly, one sees that you get a diagonal line toward the bottom left.
Ahsan, William from England, the students from Ganit Kreeda, Sam, Isabelle and student GD from Wembrook thought about which circles would be split into three identical colours. Sam wrote:
27 and 8 are made of pieces the same colour because they are cube numbers of a prime number. All of the powers of a [prime] number that can fit in the grid have unicolour patterns in their circles.
William wrote:
Only 8, 27 are this pattern as any larger prime has a cube above 100.
The students from Ganit Kreeda explained why 4$^3$ = 64 doesn't count:
But, 4$^3$ is equal to 2$^6$, so the circle for 64 will be split into 6 identical colours rather than 3 identical colours. And 5$^3$ is 125 which is bigger than 100.
Student GD from Wembrook Primary School expressed this idea using algebra:
Any number that can be written as $n^3,$ where $n$ can be any number such that $N^3\le100$ (note that, as the students from Ganit Kreeda explain, $n$ needs to be prime)
On the bottom row, 93, 94 and 95 appear as three consecutive circles/numbers, each split into two, and no colour is repeated. William, the students from Ganit Kreeda, Noah and Max, Sam, Isabelle and Jenny explained why it isn't possible to find four consecutive circles/numbers where no colour is repeated. Isabelle wrote:
I don't think you could have four consecutive numbers because the fourth number would be even like the second and have the [common] factor of two.
Some people described other patterns they noticed. Noah and Max noticed three patterns:
- all green comes in diagonal stripes
- all red is in columns
- In these numbers (we know it doesn’t work infinitely) numbers ending in 3 (with an exception of 3) the pattern on the grid goes prime, prime, not prime repeat at least until 100 but given no one has ever managed to find a pattern for primes that works infinitely this has an about 0% chance of working infinitely
Isabelle also noticed something else about the prime numbers:
In the three column there are lots of prime numbers as well as the seven and nine columns. Also most of the prime numbers appear down the columns that have odd numbers in the units. The only even prime number is two!
Henry and Emma from Holywell C of E Primary School in England found some more patterns including more diagonal lines. They also explained a lot of the patterns above. Click here to see Henry's work and here to see Emma's work.
Shaunak from Ganit Kreeda and William investigated how many numbers/circles have 6, 5 and 4 sections. Shaunak's method involved finding all the numbers:

William described the same method in words:
6 Sections:
Numbers with 6 prime factors.
Smallest is 2$^6$=64
Then by replacing each factor by the next prime factor we can get all.
Replacing 1 of the 2s by 3 gives 2$^5\times$3=96
Replacing any more goes too big so 96 is the largest.
Therefore 2
5 Sections:
Numbers with 5 prime factors.
Smallest is 2$^5$=32
Then by replacing each factor by the next prime factor we can get all.
32,48,72,80
4 Sections:
Same method as above, just takes a bit more time.
16,24,36,40,54,56,60,81,84,88,90,100
If we 'zoom in' on part of the T-shirt, do six circles offer you sufficient clues to figure out where you are on the T-shirt?
The students from Ganit Kreeda, Zac from Longridge Towers in the UK, Freddie from Rainford High School and Ong from Kelvin Grove State College Brisbane in Australia worked on the eight challenges. Chris from the United States sent in some general strategies:
I found that if you look at two circles two apart, if they share a factor, then the common factor is the factor for two. If they don't, look at two different circles two spaces apart and the common factor is two. Then look at circles three apart and see if there is a match at all. If there is then the common factor is 3. If not, then the middle circle must contain the number three as a factor somewhere. Knowing this, if there is a circle only made up of the 2 and 3 colors, then figure out what that is and use that to figure out what the numbers are. If there isn't one, then look if there are twin primes. If there are, brute force to figure it out. If there isn't, then make an educated guess of what 5 is. Exactly one multiple of 5 is in the section, so use that to your advantage. After that, just try every possibility.
This is Zac's work for challenge A:

Ong used similar ideas but different notation:

For challenges B and C, the students from Ganit Kreeda sent in two different methods. In both cases, the first method is very similar to Zac's method. Here is the Ganit Kreeda students' work for challenges B and C:

For D, E, F and G, Zac's method is slightly different to both of the methods from Ganit Kreeda. Here is Ganit Kreeda's work, followed by Zac's work, for each of the challenges, plus the Ganit Kreeda's students' work for H.

Freddie used algebraic notation to find the numbers in the challenges. Click to see Freddie's work.
Lily used the interactivity to generate windows. Here is Lily's work:
The yellow circle is a square number, because it’s got two identical factors.
The pink circle, which is a prime number (because it’s a single colour) has to be 10 more than the yellow circle. So prime numbers which are 10 more than a square number could be:
9 and 19
or
49 and 59
The green circle is also prime and has to be two less than the square number which is the yellow circle.
Two less than 9 is 7, which is prime.
Two less than 49 is 47, which is also prime. (So it still could be either pair from above.)
However. if the top line was 7, 8, 9 the middle circle should have just three sections all the same colour (2x2x2) and it doesn’t. So the top middle circle has to be 48 and the sections which are orange represent the factor 2 and dark blue represents the factor 3.
So the numbers are: 47, 48, 49, 57, 58, 59
Shaunak from Ganit Kreeda sent in several more examples:

Several students also worked on examples of Xavi's scarf using the interactivity. Chesna from Ripon Grammar School in the UK sent in this example:

Rosaleen from Bangkok Patana School in Thailand sent in this example (click on the image to see a larger version):

William sent in these examples:
Green is 2
Yellow is 3 as no other colour repeats.
5 Must occur and can’t be the primes
1st is 5: 10,11,12,13,14 no as twelve doesn’t fit
3rd is 5: 58,59,60,61,62 works!
Blue is 2
Green is 3
5 Must occur and can’t be the prime
1st is 5: 30,31,32,33,34 no as 32 doesn’t fit
3rd is 5: 18,19,20,21,22 no as 18 doesn’t fit
4th is 5: 12,13,14,15,16 no as 14 doesn’t fit
Thus, yellow is 5.
1st is multiple of 6 ending in 6: 6,36,66,96
6 has 2 prime factors
36 has 4 prime factors
66 has 3 prime factors
96 has 4 prime factors
Thus, 66,67,68,69,70
Yellow is 2
Thus: 4,5,6,7,8
Orange is 2
Blue is 3
Thus: 96,97,89,99,100
Yellow is 2
Cyan is 3
Thus: 92,93,94,95,96
Shaunak from Ganit Kreeda sent in these examples:

Thank you to everyone who submitted a solution, and thank you to those of you who told us what you enjoyed when working on the problem. Here is a selection of what you said:
Looking at this challenge made me think, for I'd never seen anything like it before! It was full of strange circles and different colours that left me puzzled for a while. Xavi's T-shirt is a 2-step problem, so you have to figure out what each circle means before you can even consider most of the questions.
It was such a nice experience to work on this live task. I was really overwhelmed when kids slowly discovered that these are showing the prime factorization of a number.
Solving puzzles and working on new puzzles from interactivity was a huge bonus for us.
Teachers' Resources
Why do this problem
This problem offers an engaging context in which to search for patterns, gain insights into the structure of numbers, and lay the foundations for an appreciation of the prime factorisation of numbers.
Possible approach
This task was the focus of an NRICH webinar in September 2023.
The way that the problem has been set out suggests a structure for a lesson, and assumes that students will have opportunities to work in pairs, and then share their insights and strategies with the whole class.
In the Settings menu of the T-shirt, you can specify a circle you'd like to include in a Window/Block/Zigzag - that number will appear top left. In Explorer mode, the number will appear in the middle.
In the Settings menu of the scarf, the number you specify will appear at the start (far left).
Possible support
Notice that when you hover over each of the circles of the full T-shirt in the interactivity, you are told which number it represents.
Some students could be asked to annotate or colour the sheet in which the circles have already been split.
Possible extension
Some students may wonder whether each 3 by 2 'window' on the T-shirt is unique. Can they convince themselves that they are? The first repeat in the 3-row 2-column window requires the T-shirt to be extend by just one row! Can students identify the two 'portrait' windows that have the same structure when the T-shirt is extended to 110?
Dancing factorisation might provide a good follow-up activity.