Polygon rings
Join pentagons together edge to edge. Will they form a ring?
Problem
Here is a pattern made of regular pentagons:

If the pattern continued, do you think it will form a complete loop or will the pentagons overlap?
Try it using the Tessellation Interactivity below.
If you've never used the interactivity before, there are some instructions and a video.
Once you've had a chance to explore, here are some questions you might like to consider.
How many pentagons form a ring?
How many decagons would form a ring?
Why do they fit together so neatly without overlapping or leaving a gap?
What about other polygons?
Can you always make a ring?
Is there a way to predict how many polygons you need to form a ring?
With thanks to Don Steward, whose idea inspired this problem.
Getting Started
You might find it useful to think about tessellations as well as the angle properties of regular polygons.
Student Solutions
Letizia from Bangkok Patana School in Thailand, Rose from Channing School in the UK, Caroline, Marcos and Jayne from Kings' School Al Barsha in UAE and Alex from Leighton Park School in the UK worked out how many pentagons form a ring.
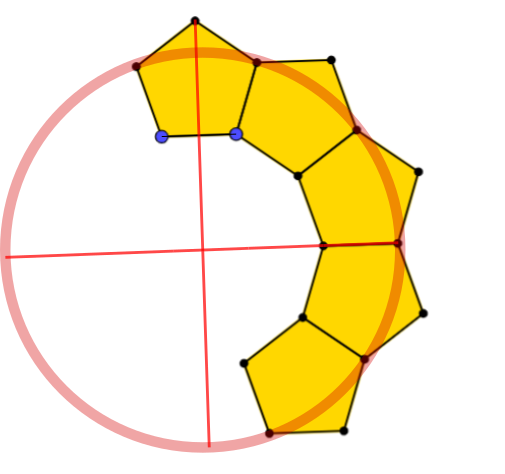
Marcos used the diagram to imagine a full ring:
Well if you were going to think of a circle you can divide it into 4 equal sizes in one of the is equal to two pentagons and a half. If you add all is equal to a loop full of pentagons [which makes 10 pentagons].
Letizia and Rose used the interactivity to make full pentagon rings. This is Rose's ring:
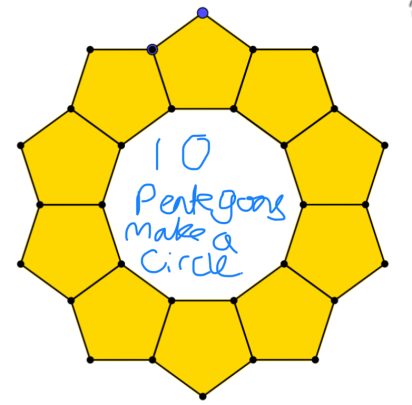
Norawee from King Georve V School in Hong Kong and Alex used angles to explain why 10 pentagons make a ring without overlapping.
Below is Alex's explanation and diagram.
Click here to see Norawee's work, which goes into more depth than Alex's.
Because the ring is made out of regular pentagons, we can work out that each of the interior angles of each pentagon is 108* degrees. By extending the lines that two pentagons share, assuming they will all meet in the middle, it will create a triangle.
As we know each angle of a [regular] pentagon is 108* degrees, we know the two base angles of the triangle would equal to 72** degrees which leaves the top angle to be 36 degrees.
As [the sum of] angles at a point is 360 degrees, and 36 is divisible by 360, it will make a complete ring.
Also, as 360 $\div$ 36 = 10, we know that the ring will be made out of 10 pentagons.
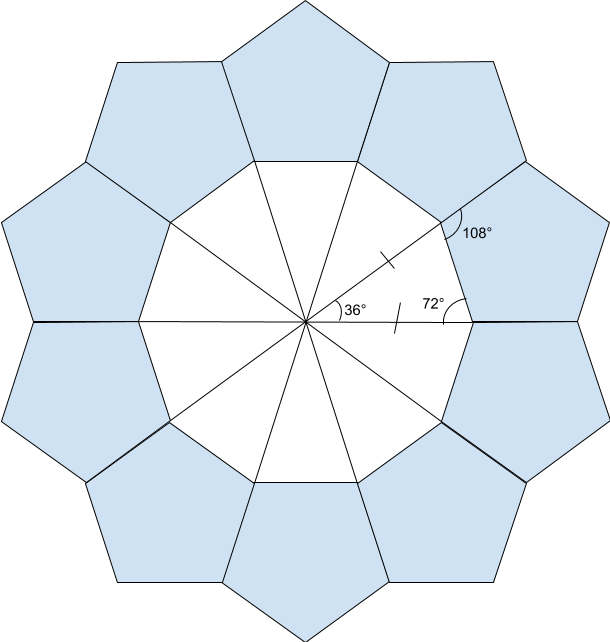
Pentagons above are regluar pentagons.
*as formula to calculate the the size of a interior angle of a polygon is $(n \times 180 - 360) \div n$ (for $n$ being the number of sides that the polygon has).
**because the triangle is made by extending the lines, and angles on a line is $180$ degrees, $180 - 108$ (an interior angle of a pentagon) $= 72.$
Caroline said 5 decagons would form a ring, and Jayne said 10 decagons would form a ring. Letizia said 5 decagons would form a ring, but sent in this picture of 10 decagons in a ring (top). Below this is a ring made from 5 decagons.
In the 10-decagon ring, the shape in the middle uses 3 sides from each decagon. In the 5-decagon ring, the shape in the middle uses 2 sides from each decagon.
If we force the shape in the middle use only 1 side of each decagon (like we did with the pentagons), then decagons cannot form a ring, because they overlap:
Which polygons can make rings around a shape which uses only 1 side of each polygon?
Pinyo from Thailand, Mimi, Denis, Jomkwan and Jessica from Headstart International School Phuket in Thailand and Iqra from Uphall Primary School in the UK sent answers to this question. Here is Jessica's work:
In a polygon ring, some particular polygons can form a ring with another polygon inside of it. Not all polygons can do this. The only weird thing about it is that the shape inside the ring has to only use one of the exterior sides.
Hexagon (6-gon)

We can seein the hexagon ring is that the shape that has formed on the inside of the ring is a hexagon. In this diagram, one side of the shape is used to make the inside shape.
Octagon (8-gon)

In this octagon, 4 put side by side made a square in the middle. Therefore this fits my investigation on polygon rings.
Dodecagon (12-gon)

In this dodecagon, 3 of each shape was used to make a polygon in the middle. As we can see the shape is a triangle and only used 1 side of each shape.
Iqra found that this also works with squares:
You can also use squares to make a ring. I used 12 squares but you can make it as big as you want.
Daniel from Moor End Academy in the UK and Mimi looked at the angles of the polygon which is formed in the middle. This is Daniel's work:
There are only regular polygons being used, so we can assume that the interior angle of every [polygon] used in the diagram [is] $\dfrac{(n-2) \times 180}n$
Where two regular [polygons] connect, the sum of the two interior angles, and $x$ (where $x$ the interior angle of the polygon in the middle), is equal to $360.$
When solving the equation $2\times \dfrac{(n-2) \times 180}n + x = 360$, we find $x$.
We should find the exterior angle to find the amount of sides. We do this by taking [$x$] away from $180$.
When we divide $360$ by [the exterior angle], we get the number of sides the polygon in the center has.
The final equation for how to make a ring out of any shape is: $$\frac{360}{-180+\left(\frac{(n-2)\times360}n\right)}=\text {sides, or number of polygons needed}$$
The denominator can be written as:$$\begin{split}-180+\left(\dfrac{(n-2)\times360}n\right)&=\dfrac{-180n}n+\dfrac{(n-2)\times360}n\\
&=\dfrac{-180n+360n-720}{n}\\
&=\dfrac{180(n-4)}{n}\end{split}$$
Which means the formula for the number of sides can be rewritten as:$$\begin{split}\text{sides, or number of polygons needed}&=\frac{360}{-180+\left(\frac{(n-2)\times360}n\right)}\\
&=\frac{360}{\frac{180(n-4)}{n}}\\
&=\frac{360n}{180(n-4)}\\
&=\frac{2n}{n-4}\end{split}$$
Florence from Walthamstow Hall Junior School pointed out that this must be a whole number. This is enough to find how many different rings there are:
$s=\frac{2n}{n-4}\Rightarrow s(n-4)=2n$
| $s$ | formula for $n$ | possible? |
| $3$ | $3(n-4)=2n\\ 3n-12=2n$ | $n=12$ (seen) |
| $4$ | $4n-16=2n\\ 2n=16$ | $n=8$ (seen) |
| $5$ | $5n-20=2n\\ 3n=20$ | no |
| $6$ | $6n-24=2n\\ 4n=24$ | $n=6$ (seen) |
| $7$ | $7n-28=2n\\ 5n=28$ | no |
| $8$ | $8n-32=2n\\ 6n=32$ | no |
| $9$ | $9n-36=2n\\ 7n=36$ | no |
| $10$ | $10n-40=2n\\ 8n=40$ | $n=5$ (seen) |
- $s$ can't be smaller than $3$ so the dodecagons are the largest polygons which can form a ring (around a triangle)
- Continue searching using $n$ since below $5$, $n=3$ or $n=4$
| $n$ | $s=\frac{2n}{n-4}$ | comment |
| $4$ | division by zero | This fits with Iqra's observation that you can use as many squares as you like |
| $3$ | negative | In fact triangles do make a ring, but there is no shape in the middle |
Which polygons can make rings around shapes which use more than 1 side of each polygon?
Mimi found the following polygon rings:
5 decagons use 2 sides each, 10 decagons use 3 sides each, 14 heptagons use 3 sides each, 18 nonagons use 3 sides each, 22 hendecagons use 4 sides each.
Teachers' Resources
Why do this problem?
This problem starts with the context of regular polygons and poses a question that will lead students on an exploration involving angles, factors, multiples and constructions. The interactive environment provides a playground for students' curiosity, and allows them to generate lots of examples in order to notice patterns, make predictions, and prove their conjectures.
You can read more about the use of digital manipulatives in the article that accompanies this feature.
Possible approach
As well as using the interactivity, you might wish to use physical tiles to investigate this problem. The Association of Teachers of Mathematics sell a range of ATM MATs which could be used. Alternatively, there are some pages of shapes that can be printed out on our Semi-regular Tessellations page.
Show the image from the problem:

"Here is a pattern made from regular pentagons. What do you think will happen if we continue to add pentagons?"
Some students might predict that 10 will form a closed loop, others might think it will not line up or that it will overlap. Take some time to discuss their ideas, focussing on vocabulary such as symmetry, parallel lines, and angles. Then resolve the discussion using the interactivity in the problem, showing that 10 regular pentagons form a closed loop with a regular decagon inside.
"How can we be sure that it's actually a closed loop and not just very close?" Students might need reminding how to find the interior angles of regular polygons, and then perhaps the class could work together on a proof that the angle between the two pentagons is in fact the interior angle of a regular decagon: $108^{\circ} + 108^{\circ}+ 144^{\circ} = 360^{\circ}$.
Students could then go on to explore polygon rings with other shapes. If laptops or tablets are available, they could create diagrams using the interactivity.
After students have worked with other shapes, you could collect together their findings in a table on the board:
| Shape | Number needed to form a ring |
| Pentagon | 10 |
| Hexagon | 6 (or 3, for a ring without a hole in the middle!) |
| Heptagon | 14 |
| Octagon | 4 or 8 |
This could lead students to explore the following key questions:
Can you make a ring with any regular polygon?
Is there a way to predict how many n-sided polygons you need to form a ring?
Possible support
Encourage students to create a polygon ring using the interactivity, and then take a screenshot to print out a large version. Then they can annotate the diagram with the angles that they know, and use it to work out other angles.
Possible extension
Students could prove what would happen with polygons with too many sides to draw using the interactivity.
Students could also explore rings made from more than one type of regular polygon, like these ones:


Semi-regular Tessellations and Which Solids Can We Make? could be explored next.
