Limits

problem
Lower bound

Age

14 to 16

Challenge level

What would you get if you continued this sequence of fraction sums? 1/2 + 2/1 = 2/3 + 3/2 = 3/4 + 4/3 =
problem
Witch of Agnesi

Age

16 to 18

Challenge level

Sketch the members of the family of graphs given by y = a^3/(x^2+a^2) for a=1, 2 and 3.
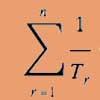problem
Reciprocal triangles

Age

16 to 18

Challenge level

Prove that the sum of the reciprocals of the first n triangular numbers gets closer and closer to 2 as n grows.
problem
There's a limit

Age

14 to 18

Challenge level

Explore the continued fraction: 2+3/(2+3/(2+3/2+...)) What do you notice when successive terms are taken? What happens to the terms if the fraction goes on indefinitely?
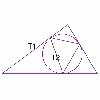
problem
Triangle incircle iteration

Age

14 to 16

Challenge level

Keep constructing triangles in the incircle of the previous triangle. What happens?
problem
Rain or shine

Age

16 to 18

Challenge level

Predict future weather using the probability that tomorrow is wet given today is wet and the probability that tomorrow is wet given that today is dry.
problem
Converging product

Age

16 to 18

Challenge level

In the limit you get the sum of an infinite geometric series. What about an infinite product (1+x)(1+x^2)(1+x^4)... ?
article
Fractional calculus III

Fractional calculus is a generalisation of ordinary calculus where you can differentiate n times when n is not a whole number.
article
Fractional calculus II

Here explore some ideas of how the definitions and methods of calculus change if you integrate or differentiate n times when n is not a whole number.
article
Fractional calculus I

You can differentiate and integrate n times but what if n is not a whole number? This generalisation of calculus was introduced and discussed on askNRICH by some school students.