Fractal

problem
Paper curves

Age

7 to 11

Challenge level

This is a simple paper-folding activity that gives an intriguing result which you can then investigate further.
problem
How long is the Cantor set?

Age

11 to 14

Challenge level

Take a line segment of length 1. Remove the middle third. Remove the middle thirds of what you have left. Repeat infinitely many times, and you have the Cantor Set. Can you find its length?
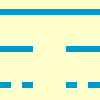problem
The Cantor set

Age

11 to 14

Challenge level

Take a line segment of length 1. Remove the middle third. Remove the middle thirds of what you have left. Repeat infinitely many times, and you have the Cantor Set. Can you picture it?
problem
Von Koch curve

Age

16 to 18

Challenge level

Make a poster using equilateral triangles with sides 27, 9, 3 and 1 units assembled as stage 3 of the Von Koch fractal. Investigate areas & lengths when you repeat a process infinitely often.
problem
Squareflake

Age

16 to 18

Challenge level

A finite area inside and infinite skin! You can paint the interior of this fractal with a small tin of paint but you could never get enough paint to paint the edge.
problem
Sierpinski triangle

Age

16 to 18

Challenge level

What is the total area of the triangles remaining in the nth stage of constructing a Sierpinski Triangle? Work out the dimension of this fractal.
problem
Smaller and smaller

Age

7 to 14

Challenge level

Can you predict, without drawing, what the perimeter of the next shape in this pattern will be if we continue drawing them in the same way?
article
Where art and maths combine

In this article, Rachel Melrose describes what happens when she mixed mathematics with art.
article
How many elements are there in the Cantor set?

This article gives a proof of the uncountability of the Cantor set.