Describing patterns and sequences
-
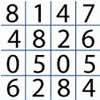 problemInvestigate and explain the patterns that you see from recording just the units digits of numbers in the times tables.
problemInvestigate and explain the patterns that you see from recording just the units digits of numbers in the times tables. -
 problem
problemSquares in rectangles
A 2 by 3 rectangle contains 8 squares and a 3 by 4 rectangle contains 20 squares. What size rectangle(s) contain(s) exactly 100 squares? Can you find them all?
-
 problem
problemChairs and tables
Make a chair and table out of interlocking cubes, making sure that the chair fits under the table!
-
 problem
problemThree ball line up
Use the interactivity to help get a feel for this problem and to find out all the possible ways the balls could land.
-
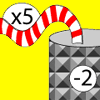 problem
problemFunction machines
If the numbers 5, 7 and 4 go into this function machine, what numbers will come out? -
 problem
problemCarrying cards
These sixteen children are standing in four lines of four, one behind the other. They are each holding a card with a number on it. Can you work out the missing numbers?
-
 problem
problemSets of four numbers
There are ten children in Becky's group. Can you find a set of numbers for each of them? Are there any other sets? -
 problem
problemHundred square
A hundred square has been printed on both sides of a piece of paper. What is on the back of 100? 58? 23? 19?
-
 problem
problemPainted cube
Imagine a large cube made from small red cubes being dropped into a pot of yellow paint. How many of the small cubes will have yellow paint on their faces?
-
 problem
problemCoordinate patterns
Charlie and Alison have been drawing patterns on coordinate grids. Can you picture where the patterns lead?
