Area - squares and rectangles
-

-
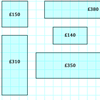 problem
problemThrough the window
My local DIY shop calculates the price of its windows according to the area of glass and the length of frame used. Can you work out how they arrived at these prices?
-
 gameFavourite
gameFavouriteDicey perimeter, dicey area
In this game for two players, you throw two dice and find the product. How many shapes can you draw on the grid which have that area or perimeter? -
 problem
problemFencing
Arrange your fences to make the largest rectangular space you can. Try with four fences, then five, then six etc. -
 problem
problemFence it
If you have only 40 metres of fencing available, what is the maximum area of land you can fence off?
-
 problem
problemPerimeter possibilities
I'm thinking of a rectangle with an area of 24. What could its perimeter be?
-

-

-

-
 problem
problemCan they be equal?
Can you find rectangles where the value of the area is the same as the value of the perimeter?
