Fruity totals
In this interactivity each fruit has a hidden value. Can you deduce what each one is worth?
Problem
Fruity Totals printable sheet - extra fruity grids
In the interactivity below, each fruit has a value between 1 and 15 inclusive.
The sum of the values of the fruit in each row and column is shown.
Can you work out the value of each individual fruit?
Once you have had a go, you might like to try a harder level. There are five levels to choose from, and you can access them through the Settings menu by clicking on the purple 'cog' in the top right-hand corner. Don't worry if you get stuck on one of the higher levels; you can always click on a question mark to reveal the hidden total.
We would love to hear about the strategies you use to solve each grid. You could send us a screen shot, or copy the code that appears in the Settings menu, along with an explanation of how you deduced the values.
You may like to explore Different Deductions (for younger students) or What's it Worth? (for older students).
This activity featured in an NRICH student webinar in November 2021.
You may also be interested in the other problems in our Is there a better way? Feature.
Getting Started
Which row/s and/or column/s give you useful information?
Can you combine or compare rows and/or columns?
Which fruit is the easiest to work out the value of first?
Student Solutions
Children from Sheriffhales Primary School sent in the following (you can view larger versions by clicking the images):
From left to right, you can see Tom and Will's, Curtis', Monty's and Joseph's solutions:




Lily May from St Ethelwold's in Wales wrote:
I used 'Explain Everything' to solve this problem and uploaded the video:
Mr G from Lily's school also gave this problem to his friends!
Kristin and Leslie from Westridge School For Girls in USA (California) sent in the following:


Molly from Guildford High School in the UK solved the following Fruity Totals grid, and explained the method for solving it in four steps:
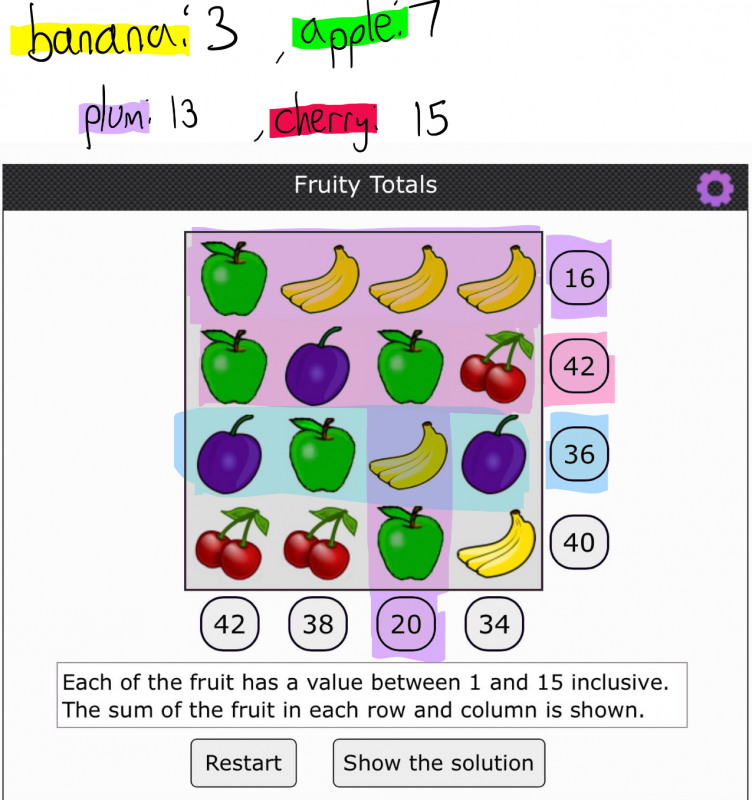
1) When finding the fruits, it helps to start with the lowest totals, highlight the two lowest. Also highlight rows and columns with the fewest amount of different fruits, these will be easiest to find the numbers the fruits represent.
2) Try to find patterns in your highlighted rows + columns and find links to the totals too.
(The picture below can be clicked on to make it bigger.)
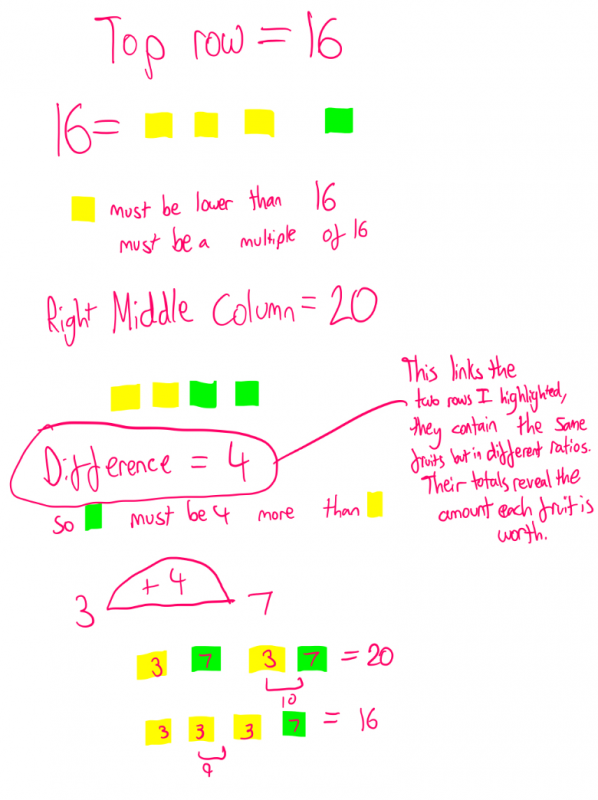
3) Now we have the banana and apple, we can figure out the other fruits like so.
Find a row where there is one type of fruit you don't know.

Find the total.
36
Subtract the numbers you know from the total.
36 - 3 - 7 = 26
If needed, divide the product until you have the individual value of the fruit.
26 ÷ 3 = 13
Plum = 13
4) Repeat Step 3 until all values are reached.
7, 13, 7, cherry
Total: 42
42 - 13 - 7 - 7 = 15
Cherry = 15
Thank you, Molly - it was very interesting to see the full method you used. I'm not sure I agree that the number for the banana must be a multiple of 16, but I really enjoyed seeing how you compared the top row and the right middle column to work out what the apple and the banana would represent.
In Step 1, Molly said that they focused on the rows and columns with the smallest totals, and also on the ones which featured the fewest amount of different fruits. I wonder which of these two focuses would be more helpful?
Isabelle from Skipton Girls' High School in the UK, Eric from the Biritsh School Manila in the Philippenes and Huu Huyen Tran from British Vietnamese International School in Vietnam used algebra.
Isabelle wrote:
Give each fruit a letter - apple ($a$), banana ($b$), cherry ($c$), plum ($p$).
Write out each sum using these letters. Find two lines with three of their fruits the same and write them as an equation:
eg. $1p+2a+1b+4=1p+1a+1c+1b$
simplify the equation
$1a+4=1c$
repeat this until you have a formula for each fruit
eg. $1b =1c+6$
$1a=1c+3$
$1p=1c+7$
pick any line and write it out using the formulas
eg. $2c+c+6+c+3=17$
simplify the equation and solve for $c$
$4c+9=17$
$c=2$
use this to work out other fruits
$c=2$, $a=5$, $b=8$, $p=9$
Teachers' Resources
Why do this problem?
This interactivity provides an opportunity for students to become familiar with an unknown quantity being represented by a symbol and to begin developing the reasoning skills needed when manipulating algebraic expressions. For older students, it is an engaging context in which to practise solving simultaneous equations.
Often, when presented with a problem like this, some students may not be able to solve the problem in the time available. As the interactivity can generate countless new grids, students can share their thinking about solution strategies and then use the insights they have gained to solve further examples. This supports students in becoming resilient problem solvers.
Possible approach
This activity featured in an NRICH student webinar in November 2021.
This task follows on from Different Deductions (for younger students) or What's it Worth? (for older students) which focus on the many different strategies that can be used to solve this type of problem.
Ideally, students would have access to computers or tablets so they can explore the interactivity with a partner. Give the class a short amount of time (five minutes or so) just to get used to the interactivity and 'play'. (See the article Using Digital Manipulatives and Interactivities to Develop Curiosity.) Bring them together and invite general comments or noticings and to address any misconceptions about the task.
"In a little while I'm going to stop you again and I want you to be able to explain a strategy that you have developed that helps you to find the values of the fruits."
Give the group some more time to work. While they are working, circulate and listen out for good reasoning and useful strategies. Each grid generated by the interactivity has a unique code, so if there is a particular grid that you would like to share with the whole class, you can access the code in the Settings menu.
Click below to see a screenshot showing how to access the code in the Settings menu.
Access the Settings menu by clicking on the purple cog in the top right corner.
When you enter a code, you need to click on the box before re-starting the interactivity (the border of the box will become pink).

These are possible starting codes which usually generate plenty of discussion:
10d8-c28-590-91e-314c-64271
d6a-1151-8b7-975-7d84-04371
98c-ca1-928-4cf-56d4-64071
98a-d67-d5f-1088-de14-57202 (Level 2)
d5f-1147-925-52c-4d2c-50211
928-10ee-c3b-8c1-b748-26541
Bring the class together and invite students to share the strategies they have been using. Here are some examples of the sorts of strategies they might suggest:
The total of three apples and a cherry is 18 so I know that an apple is worth less than 6.
This row has two apples and two bananas so I can work out the value of apple+banana, and then use this to work out the value of a plum on the row that has two plums, an apple and a banana.
These two columns are the same except one has a cherry and one has an apple, so I can work out that a cherry is worth 2 more than an apple.
Having shared and discussed different strategies, give students an opportunity to put other people's strategies into practice. You may wish to do this by having everyone in the class work on the same example.
Key questions
Which row/s and/or column/s give you useful information?
Can you combine or compare rows and/or columns?
Which fruit is the easiest to work out the value of first?
Possible support
Trial and improvement is a strategy that will allow all students to access this task, although it would be good to encourage students to move towards more sophisticated strategies.
Possible extension
Once students are confident at solving Level 1 problems, there are four more levels for them to try, which can be accessed from the Settings menu. As the levels increase in difficulty, more of the row and column totals are hidden from the student so they have less information to solve the problem.
