Triangle in a triangle
Can you work out the fraction of the original triangle that is covered by the inner triangle?
Problem
Triangle in a triangle printable sheet
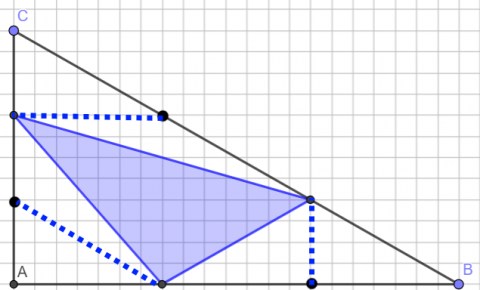
Draw a right-angled triangle $ABC$, and mark a point $\frac13$ of the way along $AB$, $\frac13$ of the way along $BC$ and $\frac13$ of the way along $CA$.
Join your three points together to form a new triangle.
Can you work out the fraction of the original triangle that is covered by your new triangle?
You may wish to explore using the interactive diagram below.
Try a few examples. What do you notice?
Can you explain why?
Perhaps it might help to add some extra lines... click below to see.
We started with a right-angled triangle... what about other triangles?

What fraction of the triangle is shaded purple?
Can you prove it?
You may wish to try Areas and ratios and Another triangle in a triangle next.
Getting Started
In the image below, how does the area of the pink triangle compare with the total area of triangle $ABC$?
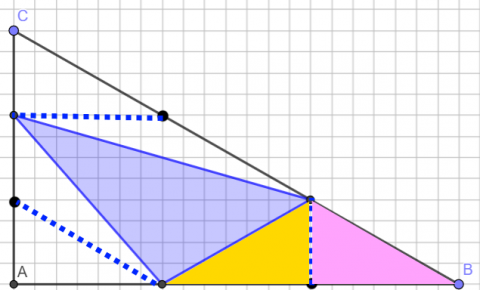
How does the area of the yellow triangle compare with the area of the pink triangle?
Student Solutions
Jaydon from Newington College in Australia found the proportion for a triangle with base 12 units and height 9 units:
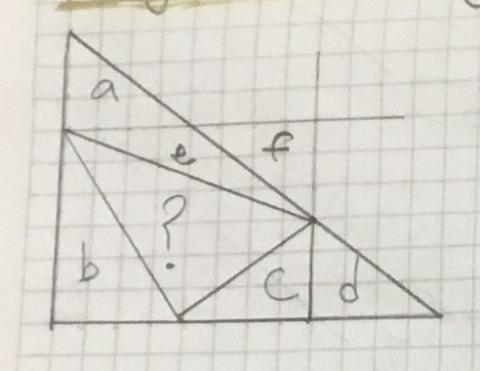
Formula to find shaded sections area:
$? = \textrm{whole}- a - b - c - d - e$
$\textrm{whole} = H\times B \times \frac12 = 9\times12\times\frac12 = 54$
$a = H\times B\times\frac12 = 3\times 4 \times\frac 12 = 6$
$b = H\times B \times\frac12 = 6\times 4\times\frac12 = 12$
$c = H\times B \times\frac12 = 3\times 4\times\frac12 = 6 $
$d = H\times B\times\frac12 = 3\times 4 \times\frac12 = 6$
To find $e$:
Take $e$ and $f$ as one shape and do the formula as per usual, but then subtract [$f$ from] the number you get from the formula to eliminate the section that is not part of the shape.
$e+f = 3\times 8 \times\frac12$
$12 - f = e$
$12 -\frac{3\times4}2 = 12 - 6 = 6$
$e = 6$
Plug numbers into formula
$? = 54 -6 - 12 - 6 - 6 - 6$
$? = 54 - 36$
$? = 18$
Ratio of ? to whole
= 18 : ?
= 18 : 54
= 1:3
Therefore as a fraction $\frac13$
Miranda and Sadaf from Greenacre Public School in Australia found a very simple solution to the general problem:
We looked at the second question part to see if we could find a pattern, and we did. We joined the dots to make 9 congruent triangles shown from the picture (1 at the top, 3 in the middle and 5 at the bottom). We then coloured in the purple-shaded triangle to work out the fraction out of 9. We saw that there were six shaded halves of the triangles and converted that into three whole triangles. This was $\frac39$ of the triangle, simplified to $\frac13$.
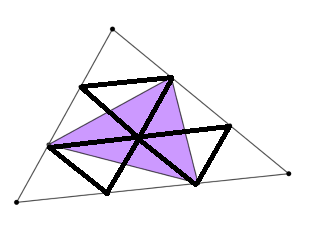
After finding the solution to the second problem, we tried the same strategy for the first part of this problem and it worked. It was harder to find how the smaller triangles would fit, but we soon found that 9 right angled triangles fit into the big triangle. We shaded the blue area and this time it was harder to measure the fraction, but we looked closer and found that it was $\frac13$ as well.
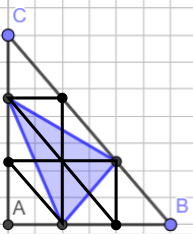
So both solutions to the equations are $\frac13$.
Thomas from Davenies found a general solution to the problem with a right-angled triangle:

In triangle ABC, there is one shaded triangle. Let us call the shaded triangle (starting at the left bottom and going round clockwise) DEF. Hence the three unshaded triangles are AED, ECF and DFB.
Let us first work out the total area of triangle ABC. Let us call the line AB $x,$ the line CA $w$ and the line BC $z.$ The [vertical] line going down from F to $x$ (in the triangle DFB) is called $y.$ As point F is one third of the line $z,$ hence line $y$ is one third of the line $w.$ Therefore triangle ABC = $3xy\div2.$
Using this same logic, we can work out the area of the non-shaded triangles. Triangle AED = $\frac13 x\times 2y \div 2=\frac13xy$
Triangle ECF = $y\times\frac23 x\div 2=\frac13xy$
Finally, triangle DFB = $y \times \frac23 x\div2$
Added together, the three unshaded triangles = $xy.$ As the total triangle = $1.5xy,$ the area of the shaded triangle will thus be $0.5xy.$ Therefore the shaded triangle represents a third of the total area of ABC.
Steven from City of Sunderland College and Tommy from the Costello School, both in the UK, used trigonometry to find the areas in a general triangle.
Tommy's working is very clear, and shows in detail how the area of each triangle was found:
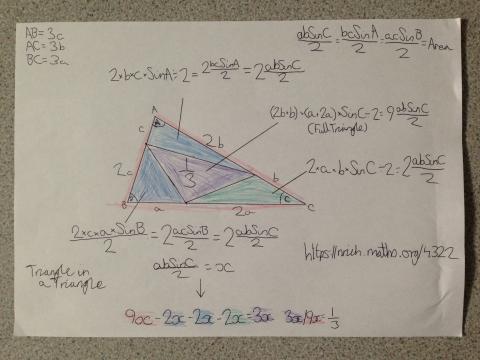
Steven used more algebra, and so in Steven's work, it is easier to see how this method is related to Thomas' method above:
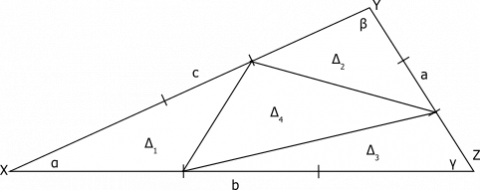
Let the area of triangle XYZ be $\Delta$, and the smaller triangles be $\Delta_1$ through $\Delta_4$, as above.
The area of the large triangle can be found as $\frac12bc\sin{\alpha}=\Delta$.
The proportions into which each side of the large triangle has been split means that, the base of $\Delta_1$ is $\frac13$ the length of the base of the large triangle, and the sloping side $\frac23$ that of the large triangle. So the area of $\Delta_1$ is $\frac12\frac b3\frac{2c}3\sin\alpha=\frac29\left(\frac12bc\sin\alpha\right)$. The same holds for triangles 2 and 3. So $\Delta_1=\Delta_2=\Delta_3=\frac29\Delta$
The area of the inner triangle, triangle 4, is therefore $\Delta-3\times\frac29\Delta=\frac13\Delta$.
Notice that, actually, it wasn't necessary to use trigonometry. Thomas' method can easily be extended to a general triangle.

This relies on the fact that multiplying the length of one of the sides by $\frac13$ or $\frac23$ has the effect of multiplying the area of the triangle by the same number.
This is because, while the base (any of the sides) remains unchanged, the perpendicular height is multiplied by $\frac13$ or $\frac23$.
A very clear method using this idea is shown below:
On the diagram the points that divide each of the sides into equal thirds can be marked. The lines connecting these points to the nearby vertex of the yellow triangle can also be drawn. This gives the following diagram:
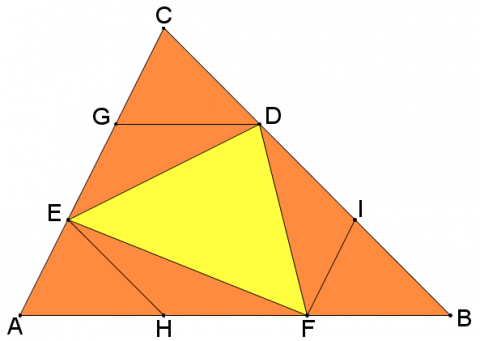
In this diagram, $AE=EG=GC$, $AH=HF=FB$ and $CD=DI=IB$, since the points trisect the sides.
Now, triangle $AHE$ is an enlargement of $ABC$ by scale factor $\tfrac{1}{3}$, as $AE=\tfrac{1}{3}AC$, $AH=\tfrac{1}{3}AB$ and $\angle EAH = \angle CAB$.
This means the area of $EAH$ is $\left( \tfrac{1}{3} \right)^2 = \tfrac{1}{9}$ of the area of $ABC$.
Since $HF = AH$, $EAH$ and $EHF$ have the same base length and the same perpendicular height (that of $E$ above $AB$), they have the same area: $\tfrac {1}{9}$ of the total area of $ABC$.
This process can be repeated at vertices $B$ and $C$, so each of the six orange triangles all have area $\tfrac{1}{9}$ of that of $ABC$.
Therefore the yellow area is $1-6\times \tfrac{1}{9} = \tfrac{1}{3}$ of the area of the whole triangle.
Teachers' Resources
Why do this problem?
In this problem, constructing some lines on a triangle divides the area in a surprising way. We hope that by trying some examples and calculating the areas, students will be intrigued by the result they notice, and curious to prove whether it will always work. Along the way, they will have plenty of opportunity to work with similar triangles, ratios and enlargements.
Possible approach
Begin by showing students a right-angled triangle such as the one below, with points marked $\frac13$ of the way along each side and joined to make a new triangle.
Invite them to draw some triangles, perhaps on squared paper, and calculate the areas. Once they have created some examples, bring the class together and share results. Perhaps students could add their results to a table on the board:
| Area of right-angled triangle | Area of triangle inside |
"What do you notice?" "The right-angled triangle's area is always three times the area of the inside triangle"
To prove the result, students might find it useful to add some extra lines to their diagram:

As the triangles are right-angled, students could use similar triangles to explain why the small triangles at A, B and C are each $\frac19$ of the area of the whole triangle. Students can then explore the triangles with the same length base that share an apex; this gives us another three triangles with area $\frac19$. Students could be encouraged to write up this argument as a proof.
Alternatively, students who are familiar with vectors may like to express the lengths in the diagram in terms of the vectors $\overrightarrow{AB}$ and $\overrightarrow{AC}$, and then calculate the relevant areas.
Key questions
Which areas are easiest to calculate?
Can we use these areas to work out the shaded area?
Are there any extra points or lines we could add?
Possible support
Students could investigate Two ladders to explore similar triangles.
Possible extension
The problem uses a right-angled triangle for ease of area calculation, but the result is true for any triangle. For some students, it might be appropriate to skip straight to thinking about this general case:

Students could then move on to Areas and ratios, and Another triangle in a triangle.
