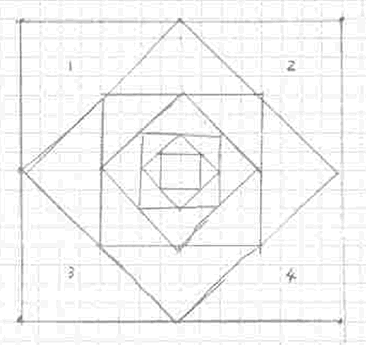
Inside seven squares

What is the total area of the four outside triangles which are outlined in red in this arrangement of squares inside each other?

Age

7 to 11

Challenge level

Being curious Being resourceful Being resilient Being collaborative

Problem

Seven squares are set inside each other. The centre points of each side of the outer square are joined to make a smaller square inside it and so on.

The centre square has the area of $1$ (one) square unit.

What is the total area of the four outside triangles which are outlined in red?

This problem is also available in French: Les 7 carrés

Click here for a poster of this problem.

Student Solutions

You have found several different ways of answering this problem which is great. Many of you, including Fiona at Tattingstone School and Douglas at Burgoyne Middle School, decided to draw the squares. However, your approaches weren't necessarily the same.

This is what Fiona says:

The first thing I did was to get some squared paper to draw the figure on. I found out I couldn't start the middle square as one square because I couldn't draw the other squares accurately so I did the middle square as a four and that gave me points for the rest of the figure.

Image	To find the total area of the numbered triangles: I noticed that $1$ and $4$ were two halves of a square and also $2$ and $3$ are two halves of a square.
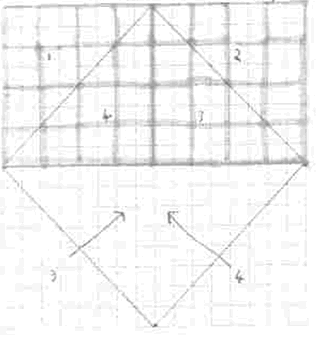
Image	Now I just have to count every square in this top rectangle as one unit: My answer is $32$ square units.

Courtney, Charlotte and Tyler from Gateway Primary also used this approach.

Here is what Douglas did:

1) I drew the squares starting with the inside square that measured $1$ cm by $1$ cm.

2) I measured the sides and calculated the area of each of the outside triangles: Height $4$ cm width $4$ cm Area of $1$ triangle = $8$ cm

Sally also sent us her solution. She spotted some interesting patterns: good work!

When I drew the squares, I knew the first one had side length $1$, then the third one had side length $2$, the fifth had side length $4$, and the seventh had side length $8$ (they kept doubling). I drew the picture on squared paper, and when I counted the squares, each square (all of them, not just the odd ones) had area twice as big as the one before. So the first one had area $1$, then the next one had area $2$, then $4$, then $8$, then $16$, then $32$, then $64$. The area of the triangles is the area of the biggest one take away the area of the next one down, so that's $64-32=32$.

Gemma and Nathalie from City of London Freemen's School, Roger, Mark and Sam from Spalding Grammar School and George from Strand on the Green Juniors also noticed this pattern and so they didn't need to do any drawing to find the solution. Nathalie wrote:

As each square is half of the square surrounding it the total area of the outside square is $64$. You then have to take away the square directly inside it to find the area of just the outside triangles. As the square directly inside it is simply half the outside square then we are left with $32$ as the area for the outside triangle.

Well done too to Callum from Arthur Mellows Village College and children from Truscott Street Public School.

Teachers' Resources

Inside Seven Squares

Seven squares are set inside each other. The centre points of each side of the outer square are joined to make a smaller square inside it and so on.

The centre square has the area of $1$ (one) square unit.

What is the total area of the four outside triangles which are outlined in red?

Click here for a poster of this problem.

Why do this problem?

This problem provides a challenge when you are focusing on drawing lines and shapes accurately, or finding areas either by counting squares or calculation. The problem calls on learners' understanding of squares and right-angled triangles, and requires working systematically, as well as visualisation and reasonably accurate drawing.

Possible approach

You could introduce this problem by making the beginnings of the design using paper folding. Each learner will need a square of paper. (This could be made in class by folding the end of the sheet diagonally, thus forming a square and cutting off the excess.)

Take the square of paper and fold it both ways diagonally so finding the centre. Fold each corner to the centre and crease it. Thus forming the second square (in red).

After this, fold each side to the centre and crease, forming the third square (in green).

Learners could be challenged to continue this process which becomes increasingly difficult and prone to inaccuracy. This introduction will show learners where the problem "is coming from".

Provide $1$ cm$^2$ paper, or even better, square dotty paper, sharp pencils and rulers for working on the actual problem. Learners would benefit from working in pairs so that they are able to talk through their ideas with a partner but should do their own drawing. This sheet, showing the diagram, could prove useful.

Learners could be left on their own to discover the best place to start the drawing and the best size to choose, or the whole group could discuss this together before they begin working individually.

At the end of the lesson learners can discuss both their methods for tackling the drawing and for finding the area of the four triangles. There are several different ways of answering the problem and it can be done without any drawing at all, so there should be plenty to talk about. It might be useful, if no one has suggested it, to cut out the four triangles and put them together as a square.

Key questions

What would be a good way to start, with the first, smallest square or the largest one?

How big should you draw the centre square to make it easier to draw the others?

Is that a $45^o$ angle?

Now you have done the drawing, how are you going to find the area of the four triangles?

Possible extension

Learners who find this problem straightforward could find the area of each of the successive squares in the diagram and predict the size of the next squares, or try the problem Fitted, and/or Baravelle.

Possible support

Children could start with a $4 \times 4$ square and then draw a second square at $45^o$ to this with each side centred on a corner of the first square. Each side of this second square will be placed diagonally across two squares. A third and successive squares can be drawn in the same way.

Or search by topic

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners