Magic crosses
Can you find examples of magic crosses? Can you find all the possibilities?
Problem
Here are pictures of two crosses:


What do you notice about the two crosses?
What is the same?
What is different?
Both crosses use the digits 1, 2, 3, 4, and 5.
The left cross has a horizontal total of 12 and a vertical total of 6.
The right cross has a horizontal total of 8 and a vertical total of 8.
We call the cross on the right a magic cross, because its horizontal total is equal to its vertical total.
Can you find any more magic crosses using the digits 1, 2, 3, 4 and 5?
Can you find all the possibilities?
Can you convince yourself you have found them all?
You may wish to use the Magic Cross Tester to try out your ideas.
Here are some questions you might like to consider:
Are there any magic crosses that use the numbers 2, 3, 4, 5, 6?
Or 3, 4, 5, 6, 7?
Or 98, 99, 100, 101, 102?
How many magic crosses can you find with the number 50 in the middle?
What if the numbers went up in 2s instead?
Or 3s?
Or 10s, or 20s, or 100s?
Perhaps you have some questions of your own that you would like to explore too!
Getting Started
You can use the magic cross tester below to find magic crosses of your own.
Choose five numbers and put them in the blue boxes.
A message will appear to tell you whether your cross is magic.
Press the reset button to start again.
Student Solutions
Well done to everyone who sent us a completed magic cross using 1, 2, 3, 4, 5.
Kristof from Moss Hall Junior School in the UK described a method for making a magic cross:
To find all possible magic crosses, a good technique is to think of numbers for a row. Then add the 1st and 3rd numbers but not the middle one. Then you find 2 numbers which also add up to that same number, but doesn't include any of the original 3 numbers. However, not all the ways to pick the [first] 3 numbers can make a magic cross.
Amelia from Slip End Village School in the UK sent in several similar crosses and an interesting comment:

This is a magic cross
I found it by moving numbers around



You could also do it like this but it is kind of cheating because I didn't change the middle numbers around
Notice that the 1 is always in the middle, the 5 is always opposite the 2, and the 3 is always opposite the 4. Does that mean Amelia is 'cheating' and they are all different versions of the same magic cross? Some people thought they were different crosses and others didn't.
Andrew from Island School in Hong Kong, Deana from Bangkok Patana School in Thailand, Iqra from Uphall Primary School and year 5 and year 6 at Tanners Brook Primary School in the UK tried putting different numbers in the middle.
Tanners Brook Primary School also considered the totals. Click here to see their work.
Andrew described a systematic approach:


Working systematically, I tried finding different crosses with 1 as the middle number. There were two magic crosses. I then tried to use 2 as a middle number. There was no magic crosses with 2 as a middle number. I hypothesized saying that the number in the middle needs to be odd in order for Magic Crosses to happen. Then I tried 3,4,5 and indeed, only 3 and 5 had magic crosses. My hypothesis was right. I also found that each middle odd number had 2 possible magic crosses however with the numbers on each horizontal side of the number becoming the vertical side numbers (as shown above).
Therefore there are technically 3 magic crosses as the other 3 is the same cross except for the numbers on the vertical side of the middle no. swapping with the numbers on the horizontal side of the number. (Andrew thinks that all of Amelia's magic crosses count as the same cross)
Libby, Isaac and Thea from Edward Bryant School, Amina, Fatimah and Yasmin from Castle Academy and Mr J's class from Banstead Prep School in the UK explained why you can't put an even number in the middle.
Edward made a video which explains this and some other concepts, which is here: https://youtu.be/9gsXurHs0V8
This explanation comes from Mr J's class:
We were not able to make magic crosses using 2 or 4 in the middle. These even numbers were not possible because you were left with three odd numbers and one even. We could not make pairs of numbers from these that were either two odds or two evens.
To make a magic cross, once you have removed/chosen your center number, you need an equal number of even and odd numbers remaining.
we found these crosses.
1 at the center = 2+1+5 and 3+1+4 (totals 8)
3 at the center = 5+3+1 and 2+3+4 (totals 9)
5 at the center = 1+5+4 and 3+5+2 (totals 10)
What about other crosses with the same number in the middle, which some people counted as different crosses?
Isaac said:
I realized that you can rotate the numbers on the outside around the one in the middle.
Amelia, Bea, Liadan, Hannah, Sophie and Lydia from Sandbach High School in the UK said
There are 8 different rotations or reflections for each different number in the middle.
Diya from International School of Brussels in Belgium sent in an image which explains how to find these 'rotations' and 'reflections'. Diya gets a total of 21: 3 rotations + 4 reflections, multiplied by 3 possible middle numbers. But Diya forgot the original unrotated version, so really there are 8 $\times$ 3 = 24. Click here to see Diya's work.
Iqra sent in some magic crosses using the numbers 2, 3, 4, 5, 6:
There are a lot more options so these are some of the ones that I thought of!
Shriya from International School Frankfurt in Germany sent examples for all of the sets of numbers given. Click here to see Shriya's first page and here to see Shriya's second page.
Owen and Jayseung from Dulwich College Beijing in China found all of the solutions using 2, 3, 4, 5, 6 using a method involving the median:
After experimenting for a long time, we found out that 2, 4, 6 are the only possible numbers in the middle if you do 2,3,4,5,6, because if you have 2, 4 or 6 in the middle, we will be able to pair up the leftover numbers so that their sum is the same.
After putting a number in the center (for example, 6), I lined up the 4 numbers from smallest to biggest (for example, 2 3 4 5).

After that, I found the median, which is 3.5. The distance 3 has from 3.5 is the same as the distance 4 has from 3.5 (0.5) so they can be a pair. The same goes for 2 and 5 so they can be a pair and the center number won't affect the total as it will be the same for the horizontal and vertical.
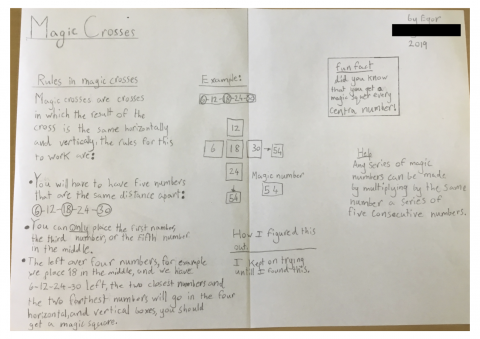
Scout from James Allen's Prep School in the UK described how a magic cross can be generated from any 5 consecutive numbers:
I want consecutive numbers 12, 13, 14, 15 and 16. Here's a little trick:
1. Put the numbers into numerical order. (12, 13, 14, 15 ,16)
2. Put the middle number (median) in the centre of the cross. (14)
3. Take the smallest and largest and put them together in a column or row. (12,16)
4. Take the two numbers closest to the median and put them in any order in
the remaining places. (13,15)
5. You have made a magic cross! Works every time!
Egor, Fede and TJ from International School of Brussels in Belgium, Deana, Thea and Libby extended their earlier ideas to other sets of numbers.
Thea said:
The second problem used the numbers 2, 3, 4, 5 and 6.
There were 8 solutions adding up to 11, 8 solutions adding up to 12 and 8 solutions adding up to 13.
The numbers used were mainly even, meaning an even number had to be in the centre square.
The third problem used the numbers 3, 4, 5, 6 and 7.
There were 8 solutions adding up to 14, 8 solutions adding up to 15 and 8 solutions adding up to 16.
The numbers used were mainly odd, meaning the number in the centre square had to be odd too.
Libby explained why if you only have 2 odd numbers, you can't put an odd number in the middle:
If you put an odd number in the middle, an odd number on top and an even number everywhere else (see picture a) it would not work because one answer is odd and one is even. When there are only 2 odd numbers, neither can fo in the middle.
It is the same with even (when there are 2 even numbers).
Deana found some numbers which could make magic crosses with the number 50 in the middle:
How many magic crosses can you find with the number 50 in the middle?
3 because if you need to find the number around it, which is
1. 50, 51, 52, 53, 54
2. 50, 49, 48, 47, 46
3. 50, 51, 52, 49, 48
Year 3 at Moulsham Junior School used numbers which were 10 apart. How are these crosses similar and different to the crosses using 1, 2, 3, 4, 5? Do you prefer the cross-representation, or the V representation?

TJ described a new method and made some magic crosses with repeated numbers:
Click here for a larger version of the image.

Fede made magic crosses with lots of different sets of numbers:
Click here for a larger version of the image.

Egor explained how to make a magic cross with any 5 numbers:
Click here for a larger version of the image.
A last minute solution came in from St. Swithun's School (Winchester)the teacher sent in a word doc of Abigail's work which extended the task quite a long way. Here is part the whole doc can be viewed here .docx
There are eight ways to do it with one number in the middle. Even numbers can't go in the middle because there will only be one even number and three odd numbers. I did this with all the numbers, and I figured out that one, three and five were the only possible numbers in the middle and there were three of them. I then figured out that that two and four were not possible because of the even reason. I figured out that 3 x 8=24 and that there were 24 ways.
A solution came in from Rishi at Orton Wistow Primary School, here is one short sentence of a very thorough solution.
What I've noticed in these crosses is that the numbers in both crosses are from 1-5. I have also noticed that in both crosses the digit 4 and the digit 2 remain stationary in the two images.
Rishi's full solution can be viewed here.docx.
Teachers' Resources
Why do this problem?
By choosing to represent the underlying patterns in different ways, such as Numicon, digit cards, the interactive Magic Cross Tester, or even algebra, we hope students will be able to persevere and find convincing arguments and proofs to explain the patterns they notice.
Possible approach
This problem featured in an NRICH webinar on December 12th 2018.
Show students the two crosses below:


"What do you notice about the two crosses? What's the same? What's different?"
Possible responses:
They both use the digits from 1 to 5
They both have a 4 on the left
They are the same shape
The one on the left has a horizontal total of 12 but the one on the right has a horizontal total of 8
The one on the right has an equal horizontal and vertical total
Establish that the cross on the right is a magic cross because the horizontal and vertical totals are the same.
"I wonder how many magic crosses there are, using the digits 1, 2, 3, 4 and 5."
Give students some time to work on the problem. Some students might ask whether it counts as a different cross if the horizontal and vertical lines are swapped, or if the left and right digits are swapped, for example. This is a good opportunity to discuss symmetry and commutativity, and to suggest that mathematicians would probably want to identify families of crosses rather than each individual cross within a family.
After students have had time to explore, bring them together to share the different crosses they have found, and pose the question "How can we be sure we've found them all?"
Here are some explanations students might come up with in order to convince themselves that there are only three magic crosses using the digits 1, 2, 3, 4 and 5:
- As the numbers from 1 to 5 add up to an odd total, the number in the middle must be odd.
- If we put a 2 or a 4 at the centre, we'd have an odd total left over to split between the two lines, so it can't be done equally.
- If there was an even number at the centre, there'd be three odds and one even left, so they can't be split to make the same total.
Next, invite the class to think of other questions they could explore. Here are some suggestions:
Are there any magic crosses that use the numbers 2, 3, 4, 5, 6?
Or 3, 4, 5, 6, 7?
Or 98, 99, 100, 101, 102?
How many magic crosses can you find with the number 50 in the middle?
What if the numbers went up in 2s instead?
Or 3s?
Or 10s, or 20s, or 100s?
Give students some time to work on the chosen questions, and then bring the class together to share any findings, convincing arguments and proofs.
Alternatively, you could set a challenge:
"In a while, I am going to choose a number bigger than 100, and challenge you to create a magic cross which contains my chosen number. You need to develop a strategy so that you can do this for any number that I choose!"
Then finish the lesson by challenging each pair of students to show that their strategy works!
Key questions
How do you know that is/is not a magic cross?
How will you keep track of what you have found so far?
How will you know when you have found all the possible solutions?
Possible extension
Possible support
Giving students digit cards or inviting them to write the numbers on small pieces of paper that can be moved around easily might help them to experiment with different arrangements.
