Surface and surface area

problem
Tiling into slanted rectangles

Age

7 to 11

Challenge level

A follow-up activity to Tiles in the Garden.
problem
Tiles in the garden

Age

7 to 11

Challenge level

How many tiles do we need to tile these patios?
problem
Changing areas, changing volumes

Age

11 to 14

Challenge level

How can you change the surface area of a cuboid but keep its volume the same? How can you change the volume but keep the surface area the same?
problem
Uniform units

Age

14 to 16

Challenge level

Can you choose your units so that a cube has the same numerical value for it volume, surface area and total edge length?
problem
Funnel

Age

14 to 16

Challenge level

A plastic funnel is used to pour liquids through narrow apertures. What shape funnel would use the least amount of plastic to manufacture for any specific volume ?
problem
Cola can

Age

11 to 14

Challenge level

An aluminium can contains 330 ml of cola. If the can's diameter is 6 cm what is the can's height?
problem
Peeling the apple or the cone that lost its head

Age

14 to 16

Challenge level

How much peel does an apple have?
problem
All wrapped up

Age

7 to 11

Challenge level

What is the largest cuboid you can wrap in an A3 sheet of paper?
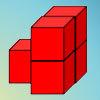problem
Brush loads

Age

7 to 11

Challenge level

How can you arrange the 5 cubes so that you need the smallest number of Brush Loads of paint to cover them? Try with other numbers of cubes as well.
problem
Tin tight

Age

14 to 16

Challenge level

What's the most efficient proportion for a 1 litre tin of paint?