Difference of two squares
-

-
 problem
problemDifference of two squares
What is special about the difference between squares of numbers adjacent to multiples of three?
-
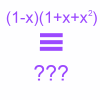 problem
problemParticularly general
By proving these particular identities, prove the existence of general cases. -

-
 problem
problemWhy 24?
Take any prime number greater than 3 , square it and subtract one. Working on the building blocks will help you to explain what is special about your results.
-
 problem
problemWhat's possible?
Many numbers can be expressed as the difference of two perfect squares. What do you notice about the numbers you CANNOT make?
-
 problem
problemPlus minus
Can you explain the surprising results Jo found when she calculated the difference between square numbers?
-
 problem
problemOdd differences
The diagram illustrates the formula: 1 + 3 + 5 + ... + (2n - 1) = n² Use the diagram to show that any odd number is the difference of two squares.
-
 problem
problem2-digit square
A 2-Digit number is squared. When this 2-digit number is reversed and squared, the difference between the squares is also a square. What is the 2-digit number?
-
 problem
problemDOTS division
Take any pair of two digit numbers x=ab and y=cd where, without loss of generality, ab > cd . Form two 4 digit numbers r=abcd and s=cdab and calculate: {r^2 - s^2} /{x^2 - y^2}.
