Odd differences

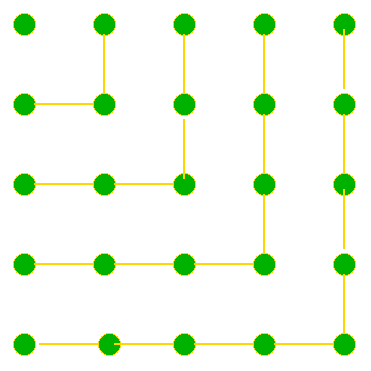
The diagram illustrates the formula: 1 + 3 + 5 + ... + (2n - 1) = nÂ² Use the diagram to show that any odd number is the difference of two squares.

Age

14 to 16

Challenge level

Exploring and noticing Working systematically Conjecturing and generalising Visualising and representing Reasoning, convincing and proving

Being curious Being resourceful Being resilient Being collaborative

Problem

The diagram illustrates the formula:

1 + 3 + 5 + ... + (2n - 1) = n ²

Use the diagram to show that any odd number is the difference of two squares.

Note that 15 = 8 ² - 7 ² as well as 4 ² - 1 ².

Write the number 105 as the difference of two squares in as many different ways as you can?

The number 1155 can be written as the difference of two squares in eight different ways, can you find them?

Student Solutions

Tom started us off with the following idea. Thanks Tom.

In the square of dots you can imagine the"L" of any odd number and if you take the square for the odd number below it from the square of the odd number you get the number you stated with. To find the two squares you have to find half the odd number and round it up and down. So half of $13$ is $6.5$ so the two squares have sides $7$ and $6$.

Here is a picture:

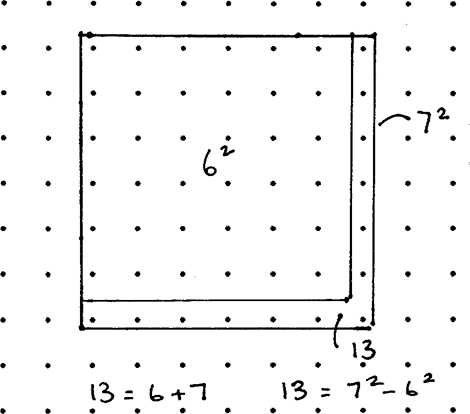
So, using the diagram you know that $$105= \frac{105-1}{2} + \frac{105+1}{2}$$

$$ 105 = 52 +53$$

$$ 105 = 53^2 - 52^2$$

You can always find one way using this method. So

$$1155= \frac{1155-1}{2} + \frac{1155+1}{2}$$

$$ 1155 = 577+578$$

$$ 105 = 578^2 - 577^2$$

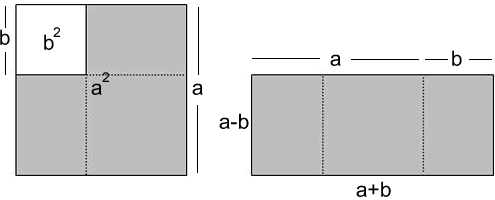
Alex, from the Grammar School at Leeds extends the algebra building on the second visualisation:

The task is to find eight pairs of numbers $(a,b)$, satisfying the following equation: $$a^2 + b^2 =115$$

The LHS can be factorized to $(a+b)(a-b)$, so now we can begin to find eight factor pairs of $1155$:

Using prime factorization,

$155 = 5 \times 231 = 5 \times7\times33 = 5 \times7 \times3 \times11$

Now we use different selections of these prime factors to build the list of eight factor pairs:

\begin{eqnarray} 1155 &=& 5 \times231 \\ &=& 7 \times165 \\ &=& 3 \times385 \\ &=&35 \times33 \\ &=&21 \times55 \\ &=& 15 \times77 \\ &=&11 \times105 \\ &=&1\times 115\end{eqnarray}

Then it is simply a matter of substituting each pair into the following procedure:

$1155 = (a+b)(a-b) = $ Large factor $\times $ small factor

$a + b =$ large factor

$a - b =$ small factor

$2b =$ large factor$-$ small factor

$b =$ (large $-$small)/$2$

$a = b + $small factor

Applying this to each pair: \begin{eqnarray} 1155 &=& 46^2 - 31^2 \\ &=&58^2- 47^2 \\ &=& 578^2 -577^2 \\ &=&118^2 - 113^2 \\ &=&86^2 - 79^2 \\ &=&194^2 - 191^2 \\ &=&34^2 - 1^2 \\ &=&38^2- 17^2\end{eqnarray}

Teachers' Resources

Why do this problem :

This connection between the set of odd numbers and squares is not often encountered in school and offers a very accessible example of visualisation.

The 'difference of two squares' is a key algebraic transformation and problems like this can lead students into a deeper appreciation of that form through a further visualisation.

Possible approach :

Start by giving learners time to make sense of the idea that any odd number can be written as the difference of two squares using the dotty grid. Encourage them to investigate with small numbers (for example, $9=5^2 - 4^2$ ) before generalising for larger numbers such as $1155$ and then devising a general rule for anyone to apply.

Remind them of the identity $a^2 - b^2 = (a + b)(a -b)$ and give them time to consider and see the connection with what they have already done. For example: $$9=5^2 - 4^2 = (5+4)(5-4).$$

At this point another visualisation might be useful in order to suggest how further differences might be found.

The problem of writing the number $105$ as the difference of two squares becomes a problem about factor pairs that make a product of $105$.

Draw out from the students how this transformation helps [we now seek factors of $105$ rather than guessing squares and calculating differences].

Moving to the last part, and even pushing beyond that to a general result, may require the group to spend time making sure that they are secure with an algorithm for producing prime factors.

Key questions :

Explain how this image shows us the sum of the first n odd numbers - what is that sum ?
For $105$ (and then for $1155$) how many ways might there be, and why do you think that ?

Possible extension :

Is it true that no number can be written as the difference of $2$ squares in exactly three ways, and if so why?

Possible support :

Perhaps try the problem Plus Minus first.

For students not yet ready for this problem, time spent on finding factors will be valuable. When the group are ready, check that they can use an algorithm to find prime factors and invite them to suggest how they can use that to assist factor-finding.

Or search by topic

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners