Vectors round a square
What is special about the relationships between vectors that define a square?
Problem
This resource is part of "Dotty Grids - Exploring Coordinates and Vectors"
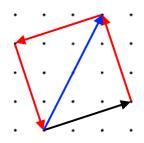
Here is a diagram showing how vectors can describe a journey round a square:
Image

The journey starts along the black vector $\pmatrix{3\cr 1}$
What vectors describe the rest of the journey?
There are many interesting mathematical questions about vectors that describe journeys.
Explore journeys round various squares of your own and see what you can find out.
If you would like some ideas of interesting questions to explore, take a look below.
What happens if I add vectors together?
What is special about opposite sides of the squares?
What is special about adjacent sides of the squares?
If I know the vector for the diagonal can I work out the other vectors?
Image
What about other shapes?
Getting Started
Student Solutions
James from Wilson's School told us something he'd noticed about the second, third and fourth vectors forming a square park:
The vector journey goes: $\pmatrix{3\cr 1} \pmatrix{-1\cr 3} \pmatrix{-3\cr -1} \pmatrix{1\cr -3}$
If the first vector has both top and bottom numbers positive, say $x$ and $y$, then the four vectors will be:
$$\pmatrix{x\cr y} \pmatrix{-y\cr x} \pmatrix{-x\cr -y} \pmatrix{y\cr -x}.$$
Elliott, also from Wilson's, and ,Niharika from Leicester High School for Girls, made some observations and completed the solution:
The coordinates of these vectors must only consist of four numbers, say $x, y, -x$ and $-y$. This is always the case as all the sides must have the same length and the angle formed by them with the vertical and horizontal lines that pass through the end point must be the same.
Also after traveling along the first vector you can either move left or right. From there, you have to do the opposite of the first move, then the opposite of the second one, to get back to the original position. This is why the third vector is minus the first one and the fourth one is minus the second one. Hence, we have the following solutions for a journey:
$$\pmatrix{x\cr y} \pmatrix{-y\cr x} \pmatrix{-x\cr -y} \pmatrix{y\cr -x} \text{ or }$$
$$\pmatrix{x\cr y} \pmatrix{y\cr -x} \pmatrix{-x\cr -y} \pmatrix{-y\cr x}.$$
Josephine from The Urswick School added:
The vectors must add up to zero. This happens because you must get back to the original position. So, if we look only at the $x$ coordinates, then if you travel away the original point a distance $d$ you have to travel it back in order to get to the original point. So you must travel a distance $-d$ as well. Therefore, the sum of the $x$ components must be $0$. Similarly, for the sum of the $y$ components. Hence, the vectors add up to zero.
Beth and Susie thought about the case when you know the diagonal vector.
The sum of the first two vectors gives the diagonal one. Therefore, if the diagonal vector is $\pmatrix{m\cr n}$, then either $$\pmatrix{m\cr n}= \pmatrix{x\cr y} + \pmatrix{-y\cr x} = \pmatrix{x-y\cr x+y} \text{ or } \pmatrix{m\cr n}= \pmatrix{x\cr y} + \pmatrix{y\cr- x}= \pmatrix{x+y\cr y- x}.$$
Case 1: $\pmatrix{m\cr n}= \pmatrix{x\cr y} + \pmatrix{-y\cr x}$
Then $x-y=m$ and $x+y=n$. By adding them we get $2x=m+n$, so $x=\frac{m+n}{2}$ and $y=\frac{n-m}{2}$. Hence, the four vectors are
$$\pmatrix{\frac{m+n}{2}\cr \frac{n-m}{2}} \pmatrix{-\frac{n-m}{2}\cr \frac{m+n}{2}} \pmatrix{-\frac{m+n}{2}\cr -\frac{n-m}{2}} \pmatrix{\frac{n-m}{2}\cr -\frac{m+n}{2}}.$$
Case 2: $\pmatrix{m\cr n}= \pmatrix{x\cr y} + \pmatrix{y\cr- x}$
Then $x+y=m$ and $y-x=n$. So, $y=\frac{m+n}{2}$ and $x=\frac{m-n}{2}$. Hence, the four vectors are
$$\pmatrix{\frac{m-n}{2}\cr \frac{m+n}{2}} \pmatrix{\frac{m+n}{2}\cr -\frac{m-n}{2}} \pmatrix{-\frac{m-n}{2}\cr -\frac{m+n}{2}} \pmatrix{-\frac{m+n}{2}\cr \frac{m-n}{2}}.$$
Well done to you all.
Teachers' Resources
For ideas on how this problem and others from the Dotty Grids Collections can be used in the classroom, you may be interested to read this article.
A printable version of this problem is available as a Word or Pdf file.
