Triangles in the middle
This task depends on groups working collaboratively, discussing and
reasoning to agree a final product.
Problem
This is one of a series of problems designed to develop learners' team working skills. Other tasks in the series can be found by going to this article.
Image

Image

What are you aiming to do?
For the task:
The team has to recreate a design comprising equilateral triangles (created by the designer) by asking as few questions as possible.As a team:
- Listening and finding out what others think
- Giving reasons for ideas
- Pulling ideas together
- Finding out whether the group is ready to make a decision.
Getting started
You will be working in a
team of four or five. You will need two sets of 12 triangles three
each of four colours. The image above uses 8 triangles of 3
different colours. You might wish to start with just two colours
and fewer triangles until you have some experience of the
task.
Choose someone in the
group to be the designer. If you try this task more than once, team
members should take turns at playing this role.
Give one set of triangles
to the designer and the other identical set to the rest of the
team.
You may also wish to
choose someone to be the observer (a really good idea if there
are more than four people in the team).
Tackling the problem
Without the rest of the
group seeing, the designer creates a design of touching triangles
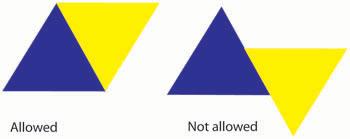
using some or all of the triangles available. Triangles must have
one side horizontal and touch at least one other triangle along at
least one side, with no overlap.
Image
Using the rules for
asking questions, and checking that they all agree first, the team
takes turns to ask the designer questions that will help them
recreate the triangle design.
When the team thinks they
have the correct design they can check with the designer and the
task ends.
At the end, the observer
gives feedback about the way the team worked together, highlighting
strengths and ways that they could improve next time. The team
discuss the feedback and how they think they worked.
Task Rules:
- You must agree any question before asking the designer.
- The team can only ask questions of the type listed in the rules.
- The team must take turns in asking questions.
- The designer can only answer "Yes", "No" or give a number or a colour.
Try to find the pattern using as few questions as possible.
Designer guidelines:
- You do not have to use all the triangles.
- Triangles must have a horizontal side.
- All triangles must touch at least one other triangle.
- The sides of adjacent triangles must touch along the whole of their length.
- You can only answer "Yes", "No", with a colour or a number.
- You only answer a question if the team has discussed and agreed it first.
Team question rules:
The team can ask
questions about:
- the number of triangles,
- the number of triangles of each colour,
- the number of "rows",
- the number of triangles in each "row'"
- the orientation of triangles,
- the colours of touching triangles (e.g. How many red triangles touch the yellow triangle?),
- the symmetry properties of the design,
- any "holes" in the design.
Note: you cannot ask the
designer to describe the position of a particular triangle.
Observer Guidelines:
Your role is to:
- identify the times when reasons for ideas are given by members of the team,
- check that everyone agrees before a question is asked,
- check that the questions fit those allowed in the rules,
- check that members of the team take it in turn to ask questions,
- count the number of questions the team asks.
Teachers' Resources
Why do this problem?
This task encourages the development of team-building skills such as sharing reasoning, allowing everyone to contribute and valuing those contributions, and coming to a consensus. This is one of a series of problems designed to develop learners' team working skills. Other tasks in the series can be found by going to this article. In addition learners are expected to use precision in describing an arrangement of objects.Possible approach
The problem is described
in terms of up to 12 triangles of four colours. However, if
learners have never worked on this sort of problem before it is
best to choose no more than eight triangles and two colours.
- You will need a screen or cloth to hide the designer's arrangement. (See ** below)
- If you do not have any triangles to hand, here is a sheet of triangles which you can print onto coloured car.
Arrange learners in
groups of four or five (fiveallows one to act as an
observer).
You may wish to ask teams
to record their questions before asking them. Then, as part of
their review of the task, the team can discuss what may have been a
more efficient set of questions to ask.
Cards for each role:
- The rule cards for questioning can be printed from this document.
- The role card for designers can be found here.
- The role card for observers can be found here.
When teams have finished
working on the task it is important that they spend time discussing
in groups, and then as a whole class, how well they worked as a
team, what they have learned from the experience and what they
would do differently next time. Your own observations, as well as
those of observers might inform the discussions.
**You may prefer to
prepare some images of an arrangement first to hand out to
designers. Designers then answer the team questions, making a note
of the number of questions asked. The team records each question
before asking it. When the groups have finished you might discuss
the number of questions used and ask the team with the fewest
questions to share their ideas with the rest of the group.
Key questions
- What things did you hear someone else say that you found really helpful?
- How well did you listen to others in your group?
- How easy was it to come to an agreement about the questions to ask?
- Did everyone always agree and what did you do if you did not?
Possible extension
Increase the number of
triangles and the colours available.
Learners may like to try
one of the other 'All for one' tasks. Other skill-building tasks
can be found by going to this article.
Possible support
Reduce the number of
triangles and colours available. Make a list of the allowed
questions, such as:
- How many triangles are there?
- How many red/yellow/green/blue triangles are there
- Is the triangle to the left of the yellow triangle blue?
- Is the triangle above the blue triangle green?
- Is the horizontal side of the red triangle at the bottom?
