The number crunching machine
Problem
Put a number at the top of the machine and collect a number at the bottom.
What do you get?
Which numbers get back to themselves?
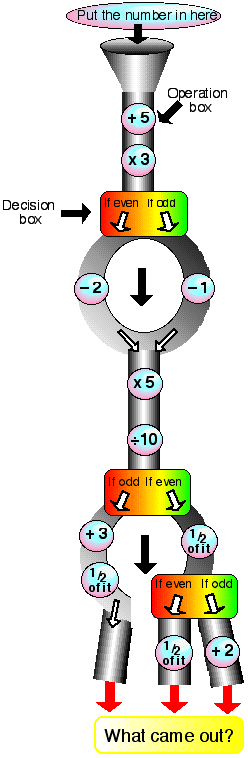
Getting Started
Try with different numbers below $50$. It may help to make a version going across the page with arrows or you could use this sheet.
Student Solutions
The Number Crunching Machine proved popular with you all and many of you noticed some wonderful number patterns. Charlotte, Julie and Lauren from Hillside County Primary School had lots to tell us.
Charlotte looked at the difference between the number that goes in and the number that comes out of the machine. She sent this table:
| Number in | Number out | Difference |
| 6 | 4 | 2 |
| 7 | 10 | 3 |
| 8 | 11 | 3 |
| 9 | 5 | 4 |
| 10 | 13 | 3 |
| 11 | 13 | 2 |
She noticed that the difference of 3 is repeated, then there is another difference (4), then 3 again, then another difference (2). I think you'd need to continue the table Charlotte to see if the pattern holds. Charlotte also found that for some larger numbers going into the number crunching machine, there seemed to be more repetition of certain numbers:
| Number in | Number out | Difference |
| 33 | 14 | 19 |
| 34 | 31 | 3 |
| 35 | 31 | 4 |
| 36 | 32 | 4 |
| 37 | 33 | 4 |
| 38 | 16 | 22 |
| 39 | 34 | 5 |
| 40 | 35 | 5 |
| 41 | 17 | 24 |
Charlotte pointed out the three fours that occur together. Again, this needs a bit more investigating to find an overall pattern I think.
Julie noticed another pattern, this time with the numbers that came out of the machine:
|
|
She explained:
The numbers that come out twice (in red above) are all odds, and they have a difference of 6. From this I can predict what the next doubles will be.
Lauren described how she was looking at numbers that come out odd and numbers that come out even. She thought that a number that came out even (for example 1 comes out as 2 which is even) could then be doubled and would come out odd (double 1 is 2, 2 comes out as 7 which is odd). However, I'm not sure that this holds all the time Lauren. Perhaps you could investigate further?
The question asked which numbers came out of the Number Crunching Machine as themselves. Several of you found one number that did: Ben from St Michael's who said he went through numbers in the 5 times tables, found 20 came out unchanged; Mollie also from St Michael's thought it might be numbers in the 20 times table, but only found 20 too; Maggie from St Anne's Convent School discovered that 19 come out the same and Ben, Rebecca and Luke from Moorfield Junior School agreed with the answer of 19.
So, we have 19 and 20 that come out as themselves. You shouldn't assume there was only one answer! Felicity from Hillside County Primary and Sheldon and Piers from Elmlea Junior School managed to find all the solutions. Sheldon and Piers say:
We have found 19, 20 and 21 so far. We don't think that there are any more numbers under 50. We can't find a pattern apart from the fact that they are adjacent numbers. We have tried 119, 120 and 121 but they didn't work and 190, 200 and 210 but they didn't work either. We would be interested to find out whether anyone has found any more.
Felicity wrote:
When you put in the numbers 1 to18 all the numbers come out bigger except four of the numbers which are: 6,9,14 and 17. Then when you put in 19, 20 and 21 they come out the same as when you put them in and from 22 upwards the numbers are always smaller than when you put them in.
Felicity also looked into some larger numbers. Thank you to everyone who sent in solutions for this problem. If anyone spots more patterns or thinks they can explain why only 19, 20 and 21 come out of the machine unchanged then email us and let us know.
