Name That Triangle!
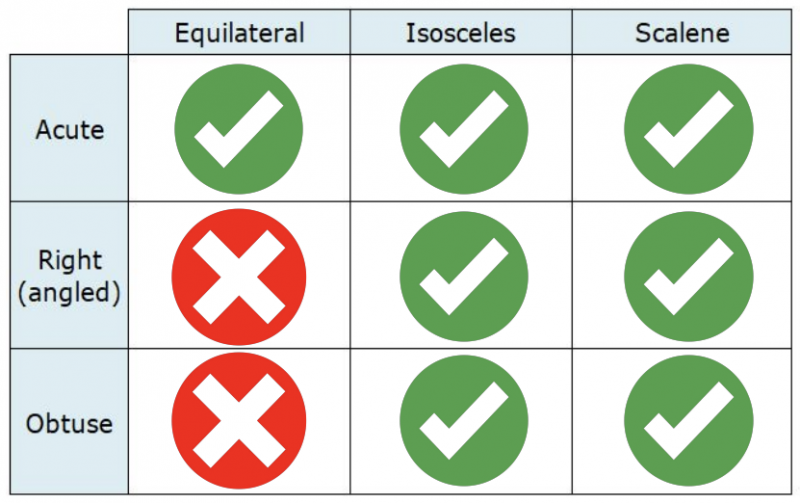
Can you sketch triangles that fit in the cells in this grid? Which ones are impossible? How do you know?
Problem
What different types of triangle can you think of?
See how many you can name, then click on the buttons below to find out what some other children have said.
Edd said:
"There are four types of triangle: equilateral, isosceles, scalene and right-angled."
Fleur said:
"There are only three types of triangle. These are equilateral, isosceles and scalene. Right-angled triangles don't count because all right-angled triangles are also isosceles or scalene triangles."
Harmony said:
"You can name a triangle using either the sides or the angles of the triangle. Using the sides, you can have equilateral, isosceles and scalene triangles. Using the angles, you get right-angled, acute or obtuse triangles."
Which of these children do you agree with? Why?
We normally name triangles by thinking about the lengths of the sides, but in this activity we will also be naming triangles using their angles. How could we do this? What do you think an 'acute triangle' or an 'obtuse triangle' might look like?
Have a look at the grid below.
This grid is to help us think about different properties of triangles. For example, a triangle drawn in the box at the top on the left, should be able to be described as both acute (when thinking about its angles) and equilateral (when thinking about its sides).
Look at each space on the grid and try to sketch some different triangles to go in the box.
Can you sketch a triangle for every box in the grid? Why/why not?
Getting Started
We normally name triangles by thinking about the lengths of the sides. If all the sides are equal, the triangle is an equilateral triangle. If two sides are the same length, we call the triangle an isosceles triangle. If none of the sides are the same length then this is a scalene triangle.
How would we name these triangles by thinking about the side lengths?
You might also have heard about naming triangles using their angles. The name 'right-angled triangle' or just 'right triangle' is used for any triangle with one right angle. If a triangle has all acute angles, it is called an 'acute triangle'. If there are any obtuse angles in the triangle, this is an 'obtuse triangle'.
How would we name these triangles by thinking about the angle sizes?
If you're stuck, you could put the triangles from this sheet into your table to start with, instead of drawing your own. Cut them out and look at one triangle at a time. Try rotating the triangle so that you can see it from different directions.
Look at the sides of the triangle - are any the same length as each other? How many are the same length? You might want to use a ruler to check!
Look at the angles of the triangle - are they all less than a right angle? Use the corner of a piece of paper to compare each angle to a right angle.
Student Solutions
Anabelle from Waverley Christian College in Australia sent in the following:
Felicity, Siya and Zara from North London Collegiate Junior School sent in this explanation:
Enclosed is our solution for Name that Triangle! that we did together in our after-school maths club.
Sofia, Jasmin, Misha, Trina, Mia and Melania, and Mohinika with Tejaswini, also from London Collegiate Junior School, sent in the following:
Scout from James Allen's Prep School began by explaining that the angles of any triangle must add up to 180 degrees and wrote explained carefully why each type of triangle is or is not possible:
Acute + Equilateral = Definite (The angles of an equilateral triangle are all 60 degrees which makes them all acute, meaning they are all smaller than 90 degrees.)
Acute + Isosceles = Possible (An isosceles triangle could be two acute or all acute. Here are some examples: 30 degrees, 30 degrees and 120 degrees or 75 degrees, 75 degrees and 30 degrees. One acute angle is not possible because you would have to have two obtuse or right angles and that alone would be more than the limit.)
Acute + Scalene = Possible (Because a scalene triangle's angles must be all different and here are some examples: 59 degrees, 60 degrees and 61 degrees or 50 degrees, 60 degrees and 70 degrees.)
Right angled + Isosceles = Possible (If you have 90 degrees, 45 degrees and 45 degrees that would work, but no other combination would. The two equal angles must be the same and in this case, we need a right angle; the equal angles cannot be 90 degrees, as 90 + 90= 180, which is the limit of triangles.)
Right angled + Equilateral = Impossible (The underlying principle of an equilateral triangle is that its angles are the same. To get them to be the same they all must be 60 degrees and a right angle is 90 degrees.)
Right angled + Scalene = Possible (If you have 90 degrees, 30 degrees and 60 degrees that is Scalene and is just one of many. In fact, with a right angle, you can just keep one angle 90 degrees and the other two can be anything if together they add up to 90 degrees and aren't both 45 degrees.)
Obtuse + Equilateral = Impossible (In order for all angles to be the same, each angle must be 60 degrees. 60 degrees is less than 90 degrees, so not obtuse.)
Obtuse + Isosceles = only possible with one obtuse angle (It depends whether you see obtuse as three obtuse or two obtuse (both impossible as it is over the limit: 180 degrees). One is possible: 120 degrees, 30 degrees and 30 degrees is just one example.)
Obtuse + Scalene = only one obtuse angle possible (A scalene can have only one obtuse angle to stay within the limit. For example, 120 degrees, 40 degrees and 20 degrees.)
Thank you, Scout, a very thorough explanation, well done!
Teachers' Resources
Name That Triangle!
What different types of triangle can you think of?
See how many you can name, then click on the buttons below to find out what some other children have said.
Edd said:
"There are four types of triangle: equilateral, isosceles, scalene and right-angled."
Fleur said:
"There are only three types of triangle. These are equilateral, isosceles and scalene. Right-angled triangles don't count because all right-angled triangles are also isosceles or scalene triangles."
Harmony said:
"You can name a triangle using either the sides or the angles of the triangle. Using the sides, you can have equilateral, isosceles and scalene triangles. Using the angles, you get right-angled, acute or obtuse triangles."
Which of these children do you agree with? Why?
We normally name triangles by thinking about the lengths of the sides, but in this activity we will also be naming triangles using their angles. How could we do this? What do you think an 'acute triangle' or an 'obtuse triangle' might look like?
Have a look at the grid below.
This grid is for matching up the different names for a triangle. For example, the box at the top on the left is for equilateral acute triangles - these are acute triangles with all three sides the same length.
Look at each space on the grid and try to sketch some different triangles to go in the box.
Can you sketch a triangle for every box in the grid? Why/why not?
Why do this problem?
This activity provides an engaging context for children to deepen their understanding of the ways of naming different types of triangles. Children are often taught about four types of triangles (equilateral, isosceles, scalene and right-angled) in isolation from each other and they may be curious to learn that this is not the whole story. We should be aiming for learners to be comfortable with the different names a triangle can have and to explore when these definitions can overlap. Discussing the characteristics of the triangles with others will improve pupils' shape vocabulary as there becomes a need to communicate efficiently.
Possible approach
Begin by discussing what pupils already know about naming triangles. How many types of triangle do children think there are? Can any triangle be more than one of these types? (Children might notice that right-angled triangles can be either isosceles or scalene.) Go through the different ideas under the 'show' buttons and give pupils the opportunity to support or challenge these ideas, giving reasons for what they think.
Recap on different types of angles and ask children what they think 'acute' and 'obtuse' triangles are. At this point in the lesson, pupils could be asked to draw some different examples of acuse, obtuse and right triangles and to explore whether or not a triangle can fall into more than one of these categories. Discuss the choice of names - why does an acute triangle need to have three acute angles, but an obtuse triangle only needs one obtuse angle? (Pupils should be encouraged to reason that at least two angles of a triangle always have to be acute, so we are only interested in what the third type of angle is. Challenging pupils to draw a picture of a triangle with two right angles or obtuse angles will help them grasp this concept.)
Once the different ways of naming a triangle have been discussed, give children the table (word, pdf) and ask them to work in pairs to draw at least one triangle in each box. If they believe it is impossible to draw a triangle for a particular box, ask them to write a sentence explaining why they think this is the case.
Bring the class back together when all pairs have finished, and discuss different triangles that pupils drew in each box. Which boxes can't have any triangles in them? Encourage children to articulate why they think this, building on each other's explanations.
Key questions
How many sides of the same length do you need?
How big will each angle be?
Can you find a triangle that goes in that box? Why not?
Possible extension
Children can see how many different triangles they can draw in each box - for example, all of the isosceles right-angled triangles will be similar triangles.
Possible support
Some children might find it simpler to cut out the triangles from this sheet (word, pdf) and put these into the table rather than drawing their own. It might be appropriate to have a go at the task Triangle or No Triangle? before tackling this one.