Magic Circles
Put the numbers $1$, $2$, $3$, $4$, $5$ and $6$ into the squares so that the numbers on each circle add up to the same amount.
Can you find the rule for giving another set of six numbers?
What does this tell you about the other four numbers?
You could draw the circles and use digit cards to move around the different positions.
Jason from Lark Rise Lower School has sent in a well explained answer.
The rule for giving another set of numbers is to put odd numbers at the top in ascending order and the even numbers in descending order.
Josh from Ampthill, UK has quite a different way of doing it.
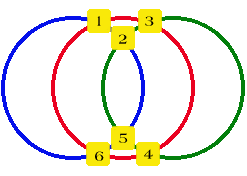
In the top three boxes there is $1$, $2$, $3$. In the bottom three there is $6$, $5$, $4$, so each of the three pairs of numbers adds up to seven. (That is; $1$&$6$, $2$&$5$, $3$&$4$.)
Another solution from Sonya from Rocky Elementary School, Canada:
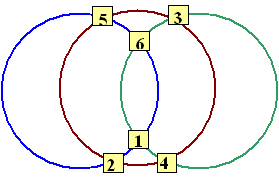
And another from Year 6 at Brookfield School, UK:
