Hexagon Transformations
Can you cut a regular hexagon into two pieces to make a
parallelogram? Try cutting it into three pieces to make a rhombus!
Age
7 to 11
Challenge level
Shown below is a regular hexagon.
Image
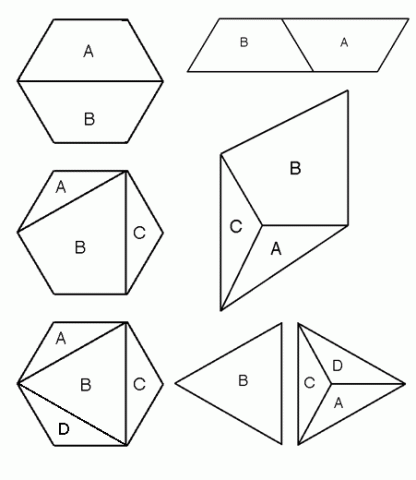
How could it be cut into two pieces which, when put together, make a parallelogram?
How could it be cut into three pieces which, when put together, make a rhombus?
How could it be cut into four pieces which, when put together, make two equilateral triangles?
You could print and cut out hexagons from this Word document or this jpg file.
What does a parallelogram/rhombus/equilateral triangle look like?
How could you cut the hexagon into two/three/four pieces?
Very good solutions to this problem were received from Melanie from West Flegg Middle School, Jack at Smithdon High School and Natalie, Jane and Paul from Methwold High School.
These pictures show how the hexagon can be split:
Image
Why do this problem?
This problem gives children the chance to explore properties of
shapes in a practical way and also encourages them to
visualise.
Possible approach
You may like to introduce
Cut and Make to the group before trying this problem. (It is a
similar idea but based on cutting up a square.)
It would be useful for learners to have access to lots of
hexagons which they can experiment with by cutting and rearranging
pieces. (Here is a Word
document of hexagons and here is a jpg file)
You could encourage children to visualise the rearrangements
before actually cutting up the hexagons. This could be organised by
asking them to talk in pairs about what they will do and then to
convince another pair that their cutting will work before they are
allowed to take scissors to paper.
Key questions
What does a parallelogram/rhombus/equilateral triangle look
like?
How could you cut the hexagon into two/three/four
pieces?