Four goodness' sake
Use 4 four times with simple operations so that you get the answer 12. Can you make 15, 16 and 17 too?
Problem
Write down the number $4$ four times, putting operation symbols between each $4$ to make a calculation.
For example, you could write $4 \times 4 \times 4 - 4 = 60$
Which symbols could you choose to make the answer $12$?
What about if you wanted the answer to be $15$? Or $16$ or $17$?
Once you've tried that, see if you can make every answer from $0$ to $10$. And once you've tried that, see if you can go all the way up to $20$!
Student Solutions
Thank you for the large number of interesting solutions that came in. Of course your answers depended upon what you decided was 'allowed'. For the first part, getting an answer of $12$, we had this variety of ideas:
$4^2 - 4 - 4 + 4$
$44+4\div4$
$(4-4/4)\times4$
$4+4+√4 + √4$
$4\times4 - √4 - √4$
From Year $3$ and $4$ Mathematics Extension Programme at Lumen Christi Catholic Primary School in Australia we had this very good solution:
To work out the solution to the problem we first tried using the four operations ($+, -, \times, \div$). This helped us find some of the solutions.
We then realised we would need to think about the order of operations to get different solutions. So we used our knowledge of brackets and BODMAS to find other solutions.
Finally we thought about other mathematical operators we could use to help us find more solutions.
We worked out that $4^2 = 16$; $4^3 = 64$ and $√4 = 2$ (square root $4$).
This information helped us find the remaining solutions except the answer.
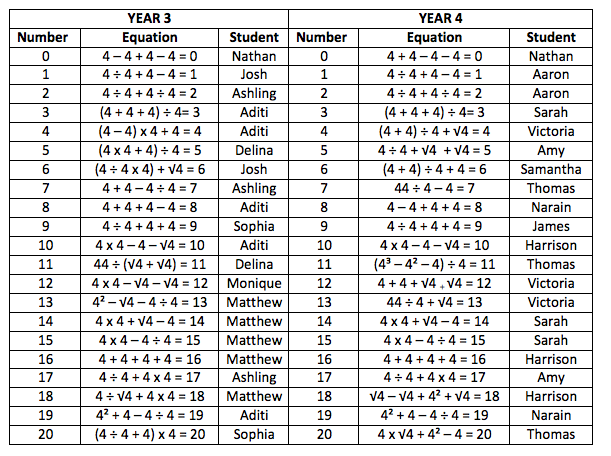
Below are the solutions for the Year $3$ and Year $4$ Mathematics Extension Programs. Each group of students worked separately on the tasks, but have found some similar solutions.
Our goal is now to find all the solutions from $0 - 100$.
Rosie Goodleigh C of E Primary School sent in the following which was accompanied by similar solutions from Paige, George, Tom, Eva and Jessica.
$0$. First I added $4+4$ which made $8$. Then $-4$ which made $4$ and then$-4$ again which uses four $4$'s and makes $0$
$1$. First I did $4$ divided by $4$ which equals $1$ so then $+4$ and then $-4$ which makes $1$.
$2$. In brackets do $4$ divided by $4$, do this once more, both of them should
equal $1$ each,add both 1s together which equal $2$.
$3$. First do $4+4+4=12$. Subsequently divide $12$ by $4$ which equals $3$.
$4$. First take $4$ away from $4=0$. $0$x$4=0$,then add $4$ which equals $4$.
$5$. First times $4$ by $4 =16$ $16 + 4 =20$. Divide $20$ by $4=5$.
$6$.First add $4$ and $4 =8$. Divide $8$ by $4$ equals $2$. $2 + 4=6$.
$7$. In brackets divide $4$ by $4=1$. Then add $4$ and $4=8$ $- 1 =7$.
$8$. Add $4$ and $4$ x $4=32$. Divide $32$ by $4= 8$.
$9$. Divide $4$ by $4=1$. Add $4$ and $4=8$ $+ 1 = 9$.
$10$. In brackets there is the square root of $4$ which is $2$. Add $4$ and $4$ and $4
=12$ $-2=10$.
$12$. In brackets do $4$ divided by $4=1$. $4 - 1 = 3$. $3$x$4=12$.
$15$. In brackets do $4$ divided by $4 =1$. Do $4$ times $4$ then minus the $1$ to
equal $15$.
$16$. Add $4$ and $4$ and $4$ and $4 =16$.
$17$. In brackets divide $4$ by $4 =1$. Times $4$ by $4$ then add $1 =17$.
Sam from The Lantern Primary School sent in a really good alternative set of answers as follows.
The first ones I did I found quite easy. I used the four simple operations.
$1=(4 \div 4) \times (4 \div 4)$
$12=(44+4) \div 4$
$3=(4+4+4) \div 4$
$6=((4+4) \div 4)+4$
$7=(44 \div 4)-4$
$8=(4 \times 4)-4-4$
$9=(4 \div 4)+4+4$
$15=(4 \times 4) - (4 \div 4)$
$16 = 4+4+4+4$
$17 =(4 \times 4)+(4 \div 4)$
Then I came to a problem. I couldn't do 4, 5 and 10. Then I realised that I had only used the simple operations, addition, subtraction, multiplication and division. Then I remembered about square root, factorial and powers. An example of factorial is:
$4!=4×3×2×1$
The first square root I tried was 4, then I realised I needed a few more. Taking these into account, I was able to do the last few problems.
$4 = \sqrt(4+4+4+4)$
$5 = \sqrt(4!+(4 \div 4)^4)$
$10 = \sqrt(4!+(4 \div 4) \times 4)$
When I checked through my answers, I realised I had forgotten to put brackets in, so I added them in in the appropriate places to make the solutions clearer.
Thank you all for your excellent ideas which I think used so much of your mathematical knowledge and skills.
Teachers' Resources
Four Goodness Sake
Write down the number $4$, four times.
Put operation symbols between them so that you have a calculation.
So you might think of writing $4 \times 4 \times 4 - 4 = 60$
BUT use operations so that the answer is $12$
Now, can you redo this so that you get $15$, $16$ and $17$ for your answers?
Need more of a challenge? Try getting answers all the way from $0$ through to $10$.
Why do this problem?
Possible approach
This is one of those problems that is harder than it looks. You could start off by presenting the question just as it is in the text. Alternatively start off by looking at all the different answers that can be gained by using just two $4$s and operation symbols first and building up to three and then four.
The children can see what answers they come up with and challenge one another to match the same solution. Challenging the children to find various numbers as solutions is interesting.
Key questions
How can you get the lowest possible answer?
Tell me about the way you are finding answers.
Can you see what target numbers you can make?
Are there any numbers that you can't make using four fours and various operations?
Possible extension
Challenge the children to make all the numbers to $64$ with four $4$s. What are the gaps? Can we fill any of them by allowing some other operation or an additional four?
The children can also set their own extension challenges.
Possible support