An easy way to multiply by 10?
Do you agree with Badger's statements? Is Badger's reasoning 'watertight'? Why or why not?
Problem
This task is best done with at least one other person so you can talk through your ideas with someone else.
In the interactivity below, you can click through a series of mathematical statements made by Badger.
When each statement is revealed, your challenge is to decide whether or not it is true and why.
Talk to someone else about your thinking. Mathematicians don't like to take your word for it, they like to see a watertight chain of reasoning that covers all possibilities. Has Badger provided that?
If you are happy with a statement, you can click on 'OK' and the next statement is shown.
If you click on 'Pause' you have an opportunity to see some other children's thinking, which might help you form your own mathematical argument. Clicking on any of the examples of children's thinking will reveal Badger's response.
We would love to hear about your reasoning at each step. Can you use what you know about number and calculations to put together a watertight chain of reasoning that would convince a mathematician?
And perhaps you could create your own series of statements like this which includes some reasoning which isn't quite right? If you send us your statements, you may see them appear as an interactive task on NRICH!
Getting Started
If you click on 'Pause' in the interactivity, you can see some examples of other children's thinking, which might help you form your mathematical arguments.
Using some hands-on equipment might be helpful.
Student Solutions
Thank you to everybody who sent in their ideas about Badger's statements. Orson sent us the following explanation:
I found that Badger's solution is wrong for 0 and all decimals and fractions.
0x10=0
6.5x10=65≠6.50
1111.33358x10=11113.3358≠1111.333580
Well done for checking Badger's reasoning with some different types of numbers! Orson has used the crossed out equals sign to show that the two numbers are not equal.
What would it look like if we added a zero to the end of 0? Would it give the correct answer to the question 0x10?
Ishita and Emily from Halstead Preparatory School in the UK had similar ideas about decimals. Emily explained:
If you add 0 to the end of a number when you times by 10 or 100 or 1000... it is not always trustworthy because if you add a 0 to a decimal you could think it would be 3.50 not 35.0
Ishita said:
0 is nothing if it is at the right end of a decimal number. If you add a 0 to the right end of 6.5 it will be the same value. But if you times it by ten it has to be 10 times larger than the original number.
It looks like adding a zero to the end of a decimal isn't the same as multiplying it by ten - well done to everybody who spotted that!
I wonder what reasoning Badger could use instead that would be true for multiplying all numbers by ten?
K from Crossflatts in the UK checked each step of Badger's reasoning carefully. K explained:
1. 10 x 2 = 20 - it is true because 10 lots of 2 is 20 and 2 lots of 10 has the answer of 20 too.
2. 10 x 74 =740 - it is true:
70 x 10 =700 - I know this because 70 = 7 x 10, and then all you have to do is multiply 70 by 10 which is 700
4 x 10 = 40 - I know 4 x 10 is 40 because I used the method for number 1 above.
So all you have to do is add them together 700 + 40 = 740.
3. 10 x 9051 = 90510 - I agree with the statement "I thought of this as ten lots of 9051, which is ten lots of 9000 added to ten lots of 51" - I used the same methods as I did above.
4. The rule will work on whole numbers but numbers with decimals might not work. e.g. 1.5 x 10 = 15 and you are not adding a 0 on.
I know this because 1 x 10 = 10 and 0.5 is half. So, 0.5 x 10 is half of 10 = 5. So 1.5 x 10 = 10 + 5 = 15.
5. 10 x 6.5 = 6.50 - this is not true. Because 6.5 is equivalent to 6.50. So adding a zero (or any amount of zeros) doesn't make a difference to the size of the decimal. So, 10 x 6.5 = 65.
Well done for checking through all of these statements, K! I wonder why 10 x 6.5 = 65? How can we work that out?
Ci Hui Minh Ngoc from Kong Hwa School in Singapore sent us the following picture to explain how to multiply by ten. You can click on the picture below to enlarge it:
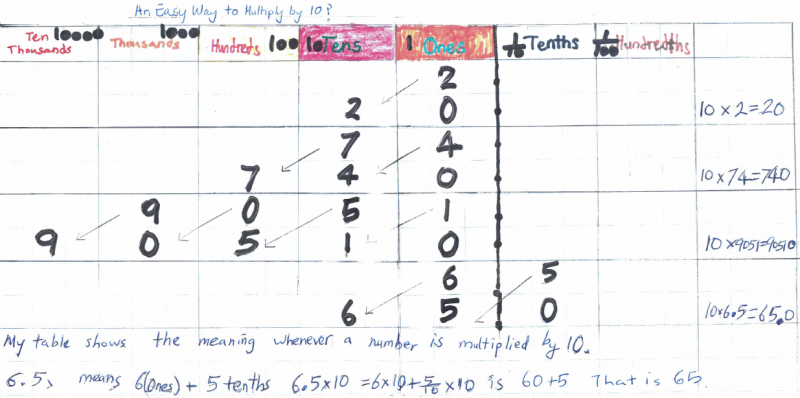
This is a very clear diagram - well done! This place value grid shows that when we multiply a number by 10, the digits move one column to the left. Ci Hui Minh Ngoc has checked the answer to 6.5 x 10 by working out 6 x 10 and $\frac{5}{10}$ x 10 separately and then adding them together.
Thank you as well to Rocky from Twyford School in England and Amélie from St Nicholas CE Primary School in England, who also drew place value charts to explain how to multiply numbers by ten.
Teachers' Resources
Why do this problem?
The idea of this task is to give children the opportunity to critique a chain of reasoning. Having this experience will help learners to appreciate what constitutes watertight mathematical reasoning, so they can create their own proofs using words (and images, where appropriate).
This particular example of flawed reasoning (that to multiply by 10 you just add a zero) is a common misconception amongst primary children, and so this task gives you the chance to tackle it and deepen children's understanding of place value.
The task Triangle in a Square offers an identical structure but in the context of geometry.
Possible approach
You may like to begin with a non-mathematical example of flawed reasoning:
Penguins are black and white.
Some old TV shows are black and white.
Therefore some penguins are old TV shows.
Give the class chance to talk in pairs about what is wrong with this, and use it as a springboard to introduce the task. Explain that being able to reason logically is a key skill for a mathematician, and the interactivity is going to give them the chance to 'unpick' someone else's reasoning.
Show the interactivity on the screen or whiteboard, with the Badger's first statement '10 x 2 = 20' showing. Invite learners to talk in pairs about whether they think that is true, and crucially, how they would use their mathematical knowledge to convince a mathematician that it was true, or not. Make sure everyone has access to manipulatives but try not to steer them in their choice initially.
Draw the whole class together to share ideas. If they are struggling to offer suggestions that convince you, click on the 'Pause' button and then reveal some other children's thinking. Do any of these examples give them a starting point? Which examples are not so helpful and why? Clicking on a particular example of children's thinking reveals Badger's response.
Continue in this way, giving everyone chance to consider each statement in turn. Only move on to the next statement when the class has a watertight mathematical argument to support or refute the previous one. In some cases, learners will come up with different chains of reasoning, not necessarily those in the examples, and that is fantastic. The key point is that the logic must be interrogated so that the class is satisfied it is correct.
Key questions
How do we know that statement is true (or not true)?
How could we check whether that statement is true (or not true)?
Are you sure that [this] follows on from [that]?
Possible support
Having a range of manipulatives available will support all learners to access this task. Try to encourage them to decide which representation might best suit the purpose. Sharing the children's thinking built into the interactivity will help those who are struggling.
Possible extension
Three Neighbours offers learners the chance to create their own proof from some suggested starting points.
