Compare the squares
In this problem you will do your own poll to find out whether your
friends think two squares on a board are the same colour or not.
Problem
Have a look at this picture of a chessboard. There is a cylinder placed on the board which is casting a shadow:
Image
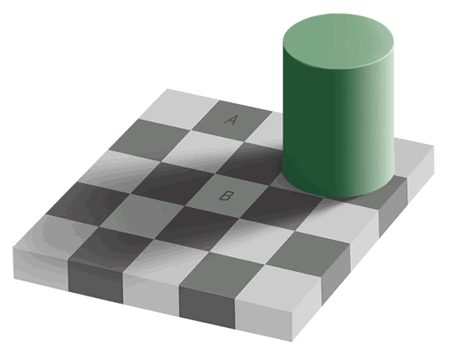
Look at the squares marked A and B.
Do you think they are the same colour or different colours?
Show this image to some other people. Perhaps you could ask other children in your class, or members of your family.
What do they think? Are the squares the same colour or different colours?
Keep a record of how many people think they are the same and how many think they're different.
When you've asked, say, 10 people (you might be able to ask more), have a look at your results.
What percentage of people you asked thought they were the same?
What percentage thought they were different?
Now, have a look at this picture . (It will open in a new window.)
This new image shows the same chessboard and cylinder, but now the squares A and B are joined by two strips which are the same colour at all points.
What do you think now? Are squares A and B the same colour as each other or not?
Show this new picture to the same people you showed the first one to.
What do they think? Are the two squares the same colour or different colours?
Do any of them change their mind compared with their first answer?
What percentage think they are the same now?
If you look at this second set of data you've collected, does it persuade you to change your mind?
How could you convince yourself and others that the squares are the same colour or not?
Getting Started
How will you keep a record of your data?
How will you work out the percentage of people who think the squares are the same? How many people did you ask altogether?
If you were able to cut out one of the squares and actually put it next to the other square, then that might make the comparison easier.
Student Solutions
This was a tricky problem! Isabella from Sion Manning RC School for Girls said:
I think the squares are different colours, because the order in which squares appear on the board are - black, white, black, white etc. On either side of square B are two black squares therefore B must be a lighter square - whereas A is a darker square.She then asked ten people whether they thought the squares were the same colour or not. Here is a table of her results:
| Person | Same | Different |
| A | x | |
| B | x | |
| C | x | |
| D | x | |
| E | x | |
| F | x | |
| G | x | |
| H | x | |
| I | x | |
| J | x |
She goes on to say:
80% of people think different colours.
20% of people think the squares are the same colours.
I still think square A and B are different colours.
Then she showed the same people the second picture. Here is the table of her results this time:
| Person | Same | Different |
| A | x | |
| B | x | |
| C | x | |
| D | x | |
| E | x | |
| F | x | |
| G | x | |
| H | x | |
| I | x | |
| J | x |
1 person changed their mind - 30% of people think the squares
are the same colour.
Did this second survey change Isabella's mind? She wrote:
No, because it is still clear that square B, is supposed to be white.
However after pasting the picture into "Paint" and colour sampling it, I discovered that the squares are the same colour (so says the computer software). Also the colour of the actual letters "A" and "B" change in both sets of data, which perhaps causes your eyes to think they're the same colour.
That's an interesting observation about the colour of the letters, Isabella, and I agree with you, it might well help to "trick" your eyes.
Rohaan from Longbay Primary School explained further:
On chessboard no.1 square B is white not grey. if you take the cylinder away there would be no shadow so square B would not look grey. On chessboard no.2 if you compare the strips with square A they are the same colour. If you compare the strips with square B they're the same colour, so it makes sense that square A and square B are the same colour.
Well done to all of you.
Teachers' Resources
The optical illusion in this problem creates a good opportunity for children to conduct an opinion poll. If each of them asks ten people outside school then you might decide to pool everyone's data and create a large sample, although this is not necessary at all - it depends on your focus. You might decide to place more emphasis on how the data is displayed.
What might be more interesting is to show just the second picture to a group of people who haven't seen the first and to see whether they immediately say the squares are the same colour. Alternatively, it might be interesting to show the first picture to a group and tell them the proportion of people who'd seen both images who thought the squares were the same colour to see whether they are influenced in any way by the statistics!
This website gives more information about the illusion and possible proofs.
