Classical means

Use the diagram to investigate the classical Pythagorean means.
Age
16 to 18
Challenge level
filled star empty star empty star
Exploring and noticing Working systematically Conjecturing and generalising Visualising and representing Reasoning, convincing and proving
Being curious Being resourceful Being resilient Being collaborative

Problem



For any two numbers $a$ and $b$ three classical Pythagorean means are defined, the arithmetic mean $A$, the geometric mean $G$ and the harmonic mean $H$ such that:

$$\eqalign{

A &= \frac{1}{2}(a+b) \cr

G &= \sqrt {ab}\cr

H &= \frac{2}{\frac{1}{a}+\frac{1}{b}}.}$$

(i) Prove that $H=\frac{G^2}{A}$.

Classical Means


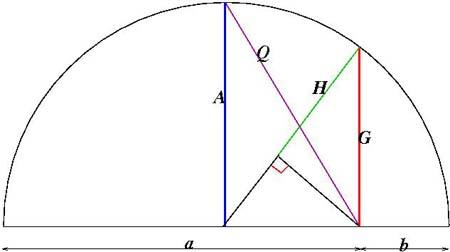
(ii) In this diagram the semicircle has diameter $a+b$. Prove that the lengths $A$, $G$ and $H$ (shown in blue, red and green) are equal to the three means and deduce from the diagram the inequality
$$A> G> H.$$


(iii) Prove from the diagram that the length $Q$ is equal to the quadratic mean (or root mean square) such that $$Q=\sqrt{\frac {a^2+b^2}{2}}.$$