Circles ad infinitum

A circle is inscribed in an equilateral triangle. Smaller circles touch it and the sides of the triangle, the process continuing indefinitely. What is the sum of the areas of all the circles?

Age

16 to 18

Challenge level

Exploring and noticing Working systematically Conjecturing and generalising Visualising and representing Reasoning, convincing and proving

Being curious Being resourceful Being resilient Being collaborative

Problem

A circle of radius 1 cm is inscribed in an equilateral triangle. A smaller circle is inscribed at each vertex touching the first circle and tangent to the two 'containing' sides of the triangle. This process is continued ad infinitum....

What is the sum of the circumferences of all the circles?

What is the sum of their areas?

Adding all the circumferences or adding all the areas, which sum grows faster?

Student Solutions

Thank you Tom from Wolgarston High School, Hidayah who does not name a school, Hamish and Jared from Ulverston Victoria High School, Eduardo from The British School, Manila, Justin from Skyview High School, Billings, MT USA and Fabian from Sabino High School in Tucson, Arizona, U.S.A. for sending your very good solutions to this problem.You all tackled the problem in a similar way.

Let $C$ be the centre of the central circle. Then $PS$ is a tangent to this circle. Then triangle $CSP$ is a 30-60-90 triangle and, given that $CS = 1 $ cm, it follows that $PS = \sqrt{3}$ cm and $PC = 2$ cm. Hence the height of the largest equilateral triangle is $3$ cm. ($PC$ + radius of $ 1$ cm.), the height of the next size equilateral triangle is $1$ cm. ($PC$ - radius of $1$ cm.) and the radius of the next size circle is $1/3$ cm.

Each circle is scaled down by a linear scale factor of $1/3$ and by an area scale factor of $1/9$.

There is one central circle and at each stage after that 3 new circles are added.

The 'total circumference' is the sum of the circumferences of all the circles, given by:

Total circumference = $ 2\pi + 3 ({ 2\pi\over 3} + {2\pi\over 9} + {2\pi \over 27}+ \ldots ) $

where the dots denote that the sum goes on for ever. Summing this infinite geometric series gives: $$ \mbox{Total circumference} = 2\pi + 2\pi (1 + {1\over 3} + {1\over 9} + {1\over 27} + \ldots ) = 2\pi + \frac{2\pi}{1 - 1/3} = 5\pi $$ The 'total area' is the sum of the areas of all the circles, given by:

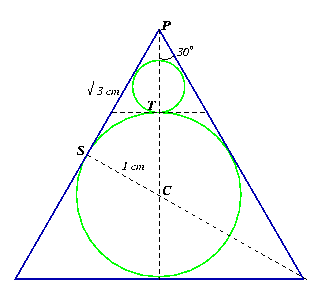
Total area = $ \pi + 3 ({\pi\over 9} + {\pi\over 9^2} + {\pi\over 9^3} + \ldots )$

where the dots denote that the sum goes on for ever. Summing this infinite geometric series gives: $$ \mbox{Total area} = \pi + {\pi\over 3} (1 + {1\over 9} + {1\over 9^2} + \ldots ) = \pi + \frac{\pi}{3(1 - 1/9)} = \frac{11\pi}{8} $$ Clearly, the total of the circumferences grows faster than the total of the areas because the scale factor 1/3 is bigger than the scale factor 1/9.

Or search by topic

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners

Circles ad infinitum

Problem

Getting Started

Student Solutions

Teachers' Resources