Calculus analogies
Consider these analogies for helping to understand key concepts in
calculus.
Age
16 to 18
Challenge level
Problem
In mathematics it is often useful to have different ways of viewing concepts in order to help to build up intuition; however we need to know to what extent analogies are mathematically trust-worthy. Imagine the following proposals for analogies concerning calculus -- some might be good analogies, some might sometimes work and some might simply not work at all.
Consider and test these proposed analogies for understanding aspects of calculus carefully, applying them to several examples. Which analogies are largely sound, and which fail to work? Provide examples of functions to exemplify your points.
Analogy: A curve is a road on a map
Imagine an analogy where the curve of a function represents a road drawn on a map. Imagine driving along this road, starting from the left (west)
Image
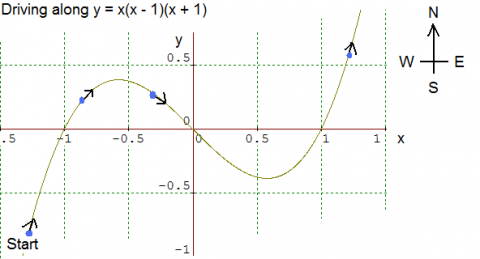
1. Sign of the derivative of a function at each point
The derivative of the function is positive when travelling towards the north, negative when travelling towards the south.
2. Sign of the second derivative of a function at each point
If your steering wheel is turned clockwise from the neutral position then the second derivative is negative. If it is turned anticlockwise from the neutral position then the second derivative at that point is positive.
3. Sign of the third derivative of a function at each point
If the steering wheel is in the process of turning in the anti clockwise direction then the third derivative is positive. If the steering wheel is in the process of turning in the clockwise direction then the third derivative is negative.
4. Differentiability condition at each point
The function is differentiable at points on the road when is it possible to drive along smoothly without having to suddenly turn the steering wheel.
5. Points of inflection
Points of inflection occur at the points, and only the points, where the steering wheel passes through the neutral position.
Note on terminology: The 'neutral position' is the position of the steering wheel in which the car travels forwards in a straight line. A clockwise turn from this position causes the car to turn right and an anticlockwise turn from this position causes the car to turn left.
Getting Started
It can be useful to hold your hands as if holding a wheel and
imagine driving along a road!
Don't forget to focus your attention on the main concepts in the problem and don't get distracted by questions such as what if the road is not flat etc. This is a mathematical idealisation!
Don't forget to focus your attention on the main concepts in the problem and don't get distracted by questions such as what if the road is not flat etc. This is a mathematical idealisation!
Teachers' Resources
Why do this problem?
This fun problem will hopefully prove incredibly useful to all students: having a sound geometrical visualisation for concepts in calculus is essential in any application beyond the simplest algebraic examples and also proves very useful in checking that calculations make sense. It will also be very useful for uncovering misconceptions about calculus.Possible approach
You might want to hand out
these cards in Word 2003 or pdf format so that students can more easily
consider the statements under discussion.
This need not be a long activity and can be used at any point
in the curriculum where the concepts in any of the 5
analogies have been encountered. You can focus on a couple of
the most relevant analogies if desired.
You could simply set up the situation and let the students
enter into discussion. Students can think about the ideas in small
groups and sketch 'road maps' on which to test their ideas.
Alternatively, you can sketch a curve with, say, 4 turning
points on the board and ask for a volunteer to model the motion of
the imaginary steering wheel as you trace your finger along the
curve. Another volunteer can record the motion of the steering
wheel, paying particular attention to the direction or speed of
turn. You could then sketch a more 'demanding' road and repeat the
exercise.
There are at least three levels of approach to this
problem:
1) Once students are intuitively clear as to which analogies
are largely reliable the lesson can move on and the analogies can
be referred to as a guide throughout subsequent study of
calculus.
2) Students can try to construct convincing justification that
the analogies are sound, including some thought on when the
analogies break down (i.e. what sorts of roads do the analogies
work for, and what sorts of 'pathological' roads do the examples
not work for?)
3) Students might try to come up with some analogies of their
own which others might test out. For example, other analogies for
the sign of the gradient might involve mountains, valleys or
hills.
Note that various misconceptions might be unearthed during
this task, and many more advanced concepts in mathematics might be
raised by students. See the possible support below for some of
these.
Key questions
Who can drive a car? Who can describe the motion of a wheel
through a journey?
Can you imagine driving along the road indicated on this
map?
For what sorts of crazy curves might these analogies not
work?
Can you give a clear justification for you results (using
words or algebra)?
What can we say about a car which is moving due north at
some point?
Possible extension
The key advanced extension is to try to create analogies for
other concepts in calculus. This is very open ended, but will
really get students thinking about calculus as the mathematics of
rates of change.
All students might naturally move on to the problem Patterns of
Inflection after trying this problem.
Possible support
Some students, who equate mathematics with algebra, might
struggle to see this as 'mathematics'. Reassure them that the
visualisation practiced and the explanations constructed are a key
part of advanced mathematical thinking.
Some students, even the most traditionally 'able', might find
the visualisation aspect of this problem extremely difficult. Such
students need to be encouraged not simply to give up and to
exercise this part of their mathematical brain. Perhaps others
in the group might try to explain the concepts to them?
Misconceptions or errors to look
out for are:
1. The steeper the gradient the more the wheel needs to be
turned
2. A function can be used to describe, say, a circle (No: A
function is single valued)
3. A point of inflection must also be a stationary point (No:
That is a stationary point of inflection)
Advanced concepts in mathematics
which might be raised in some form are:
1. What is a function as opposed to a curve?
2. What is a continuous / differentiable function?
3. What is curvature?
4. Are there functions which are only twice
differentiable?