Bobbly perimeter
Find the perimeter of this shape made of semicircles
Problem
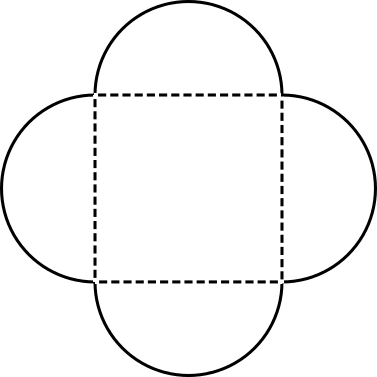
A square with perimeter 20 cm has a semicircle drawn onto each of its sides, as shown below.
What is the perimeter of the new shape? Give your answer in terms of $\pi$.
This problem is adapted from the World Mathematics Championships
What is the perimeter of the new shape? Give your answer in terms of $\pi$.
Image

This problem is adapted from the World Mathematics Championships
Student Solutions
Finding the lengths of the arcs
If the perimeter of the square is 20 cm, then each side must be 5 cm long.
The sides of the square are the diameters of the semicircles, so the circumferences of the full circles would be $5\times\pi=5\pi$ cm.
As shown in the diagram below, the 4 semicircles make up 2 full circles.

So the total perimeter is $5\pi+5\pi=10\pi$ cm.
Using scale factors
The sides of the square are the diameters of the semicircles, and so the circumferences of the full circles would be $\pi\times\text{diameter}=\pi\times\text{side length}$.
Each semicircle has only half the circumference of a full circle, so its length is $\frac{1}{2}\pi\times\text{side length}$.
So to go from a square to a semicircle, each side length is mutliplied by a scale factor of $\frac{1}{2}\pi$. So the perimeter must also be multiplied by this scale factor. So the perimeter of the new shape will be $20\times\frac{1}{2}\pi=10\pi$ cm.
If the perimeter of the square is 20 cm, then each side must be 5 cm long.
The sides of the square are the diameters of the semicircles, so the circumferences of the full circles would be $5\times\pi=5\pi$ cm.
As shown in the diagram below, the 4 semicircles make up 2 full circles.
Image

Using scale factors
The sides of the square are the diameters of the semicircles, and so the circumferences of the full circles would be $\pi\times\text{diameter}=\pi\times\text{side length}$.
Each semicircle has only half the circumference of a full circle, so its length is $\frac{1}{2}\pi\times\text{side length}$.
So to go from a square to a semicircle, each side length is mutliplied by a scale factor of $\frac{1}{2}\pi$. So the perimeter must also be multiplied by this scale factor. So the perimeter of the new shape will be $20\times\frac{1}{2}\pi=10\pi$ cm.
