Pairs of numbers
If you have ten counters numbered 1 to 10, how many can you put into pairs that add to 10? Which ones do you have to leave out? Why?
Problem
Pairs of Numbers printable sheet
If you have ten counters numbered 1 to 10, how many can you put into pairs that add to 10?
Can you use them all?
Why or why not?
Now put the counters into pairs to make 12.
- Can you use them all?
- Why or why not?
Now put the counters into pairs to make 13.
- Can you use them all?
- Why or why not?
Now put the counters into pairs to make 11.
- Can you use them all?
- Why or why not?
Getting Started
It might help to have counters numbered from $1$ - $10$ to do this problem.
Student Solutions
Thank you to everybody who sent in their ideas about this challenge. Lots of children didn't spot that there is only one of each counter available!
Sree and Tanishka from Moore Elementary in the USA wrote:
We did not use 1 and 2 in pairing (to make 12 and 13). 10 is the friendly number so easy to pair
I wonder what they mean by 'friendly number'? Why might it be easy to add one-digit numbers to ten?
Hughes Class from Pennyman Primary Academy in England agreed that 1 and 2 could often not be used, and they sent in a picture showing how they worked on this task:
We identified not all counters can be paired to make 12! 1 would need to be paired with 11.
We also found out 1 and 2 couldn't pair with any others because 1 would need to be paired with 12 and 2 would need to be paired with 11 when making 13.

Finn from Daneshill in the UK noticed that 5 and 6 can't be used to make 10 and 12, respectively:
Question 1 no because when you try and add 5 with any thing other than 5 it does not work and you don't have 2 5's
Question 2 no because when you add 6 it does not work because you don't have another 6
Jade had similar ideas, working systematically to find the pairs that add up to 10:
Find all pairs that add to 10:
1+9=10
2+8=10
3+7=10
4+6+10
Why not 5?
5+5=10 but we only have 1 counter numbered 5
Why not 10?
10+0=10 but we do not have a counter numbered 0
Rosa from Dulwich Wood Primary in the UK also worked systematically on this problem, and found that 11 was the only total that had no numbers left over:

Maya from St. Martin's Catholic Primary in England drew some diagrams to show the pattern:
I looked at number 1 and number 10 and realised it didn't add up to 12 so then I looked at 2 and 10 and it added up to 12. I looked at 3 and 9 and it added up to 12, then 4 and 8. I noticed a pattern because I saw they were in the order of 1 to 10 and I noticed they were getting closer to each other. It worked in the same way for the total of 11 but you could use all the numbers.

These diagrams make it really easy to see the pattern!
Jason from Sequoyah Middle School in the USA noticed that there is something happening with odd and even numbers here:
You can't use all of the numbers as pairs to add to ten as five and ten cannot be used. Ten cannot be used because it has to be in a pair, and 5 cannot be used because you only have one of each number. You can't use all the numbers in pairs to add to twelve as one and six won't work. One and ten do not add up to twelve, and six cannot be used twice. You also can't use all the numbers in pairs to add to thirteen because you can't use one or two, as being in a pair with ten does not have a sum of 13. However, every number can be used in pairs to get eleven because it's an odd number, so no number needs to be used twice to get it, and one and ten do have a sum of eleven, and every other combination works too.
This is interesting, Jason. Why does 11 being an odd number mean that no number needs to be used twice to get it?
The children at CHPS in Australia talked about using 'friends of ten' (number bonds to ten) to help them. Evie sent in this image to show one way of doing this:
In pairs of numbers I set up all the pairs like the tens which were easy because I learnt them from Friends of ten, ten then 11, 12 and 13 which was little more challenging but I completed them by alternating the friends of ten. Then I lay them out on a neat (Rainbow) sheet so they were easy to see.
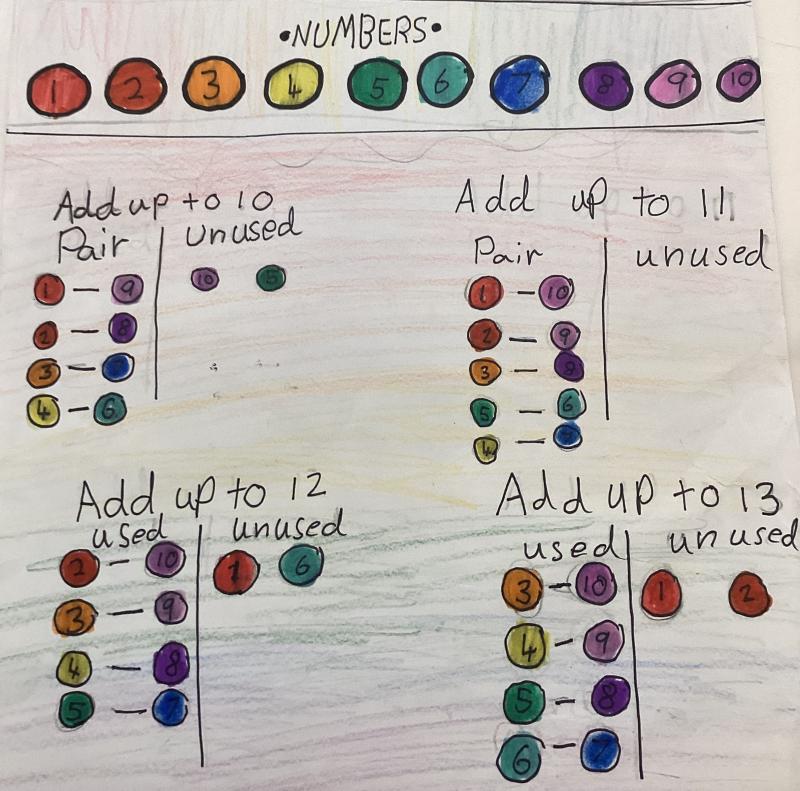
Monty, Eli, Alice, Sas and Maya from CHPS also worked systematically to find similar solutions. We often focus on number bonds to 10, but it looks like the number bonds to 11, 12 and 13 can be found in a similar way!
Kimaya, Mrunmayee, Aarav, Ruhi, Amey, Siddharth, Reyansh, Vihaan, Vivaan, Rudra, Aaryavir, Rudravir and Swara at Ganit Kreeda, Vichar Vatika in India thought hard about this problem. They decided that if the total chosen is the sum of the smallest number and the biggest number then it will be possible to use all of the numbers to make it - but only when there are an even number of numbers in the list.
This is a good idea. I wonder how this relates to Jason's idea about odd and even numbers?
If you'd like to see more of their ideas about this activity, take a look at Ganit Kreeda's full solution.
Teachers' Resources
Why do this problem?
This problem looks simple to start with, but it has a certain complexity. It is a great opportunity to encourage children to justify their thinking, which they may find quite difficult at first.
Possible approach
All children will need access to ten counters or number cards numbered from $1$ - $10$. Having counters to move around will help free up their thinking and means they can try out lots of ways without the fear of having something committed to paper which might be wrong. Some children may also need some unnumbered counters or Multilink cubes to help them with the calculations.
Key questions
What goes with this number to make $10$/$11$ etc?
Possible extension
Children could try to find other numbers of which can be made from pairs of the numbers $1$ - $10$. Are there any number which can't be used?
What can they do if they use the numbers from $1$ - $12$ instead of $1$ - $10$?
Possible support
Some learners may need support with the calculations, so having number lines, blank counters or other equipment available will be useful. This task offers children the chance to practice adding numbers in a meaningful context.
