Times tables shifts
In this activity, the computer chooses a times table and shifts it. Can you work out the table and the shift each time?
Problem
The numbers in the five times table are:
5, 10, 15, 20, 25 ...
I could shift these numbers up by 3 and they would become:
8, 13, 18, 23, 28 ...
In this activity, the computer chooses a times table and shifts it.
Can you work out the table and the shift each time?
Can you explain how you worked out the table and shift each time, and why your method will always work?
Levels 1 and 3 include tables up to 12.
Levels 2 and 4 include tables up to 20.
On levels 1 and 2, the numbers will always be the first five numbers in the times table.
On levels 3 and 4, the numbers could be any five numbers from the shifted times table.
You may be interested in the other problems in our Number Patterns Feature.
Getting Started
What is the same about numbers in a times table and the numbers in the shifted times table?
On level 3, how about putting the numbers in order?
Student Solutions
Thank you to everybody who sent us their strategies for this activity.
James from the UK noticed a pattern in the solutions:
The shift up and down adds up to make the times table that has been shifted.
This worked with all of the times tables and patterns.
Well spotted! I wonder why this happens?
Helly from CHPS explained how to use trial and improvement to find the solution:
I first ordered the numbers from biggest to smallest, then put them on a number line. Then I tried taking away different numbers to the ones I already had. (like 2,4 and 5) I saw that those numbers did not make any times tables patterns. After taking away three, I found that when you minus off three to each of these numbers, it creates numerals in the six times tables, This strategy - the trial and error strategy - will always work because you will always narrow down and get the numbers you need, even though if might not be the most efficient strategy.
Putting the numbers in order certainly seems to be a helpful starting point with this problem. The children at St. Mary's International School in Tokyo, Japan also did this, but then went on to use a slightly different strategy. Edward explained:
When finding which times table the numbers shifted from, just look at the two numbers which make the smallest difference. The difference is the times table the numbers shifted from.
When finding the shifted up by box, pick a number and find the nearest multiple the number in the table box that is before the number you picked.
When finding the number in the shifted down by box, pick a number and find the nearest multiple of the number you put in the table box that is after the number you picked.
Note: In levels 1 and 2 all the differences between the numbers will all be the same. When this happens, just take the same difference that any two numbers will have.
Ren shared this solution for level 1:
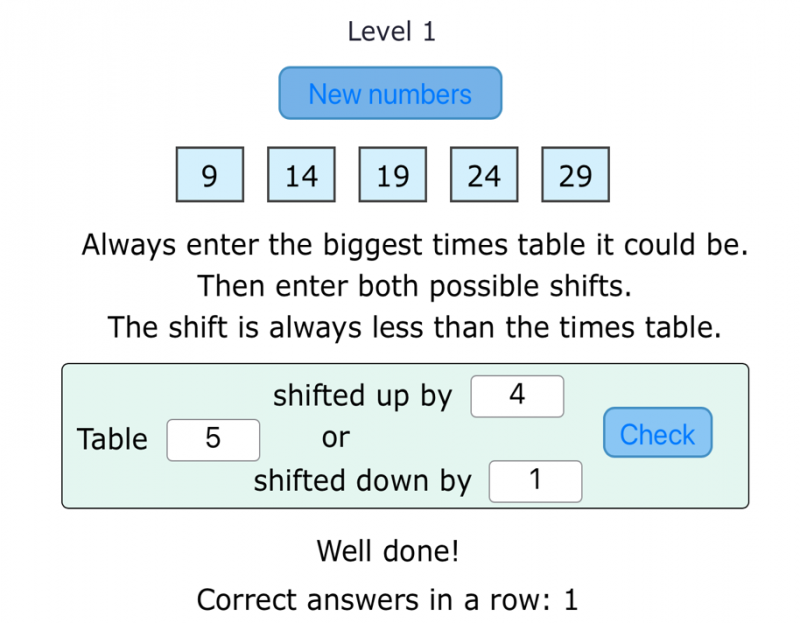
When the numbers are like this, the table has to be 5 because, 14-9=5 and that’s the table.
2. The "Shifted up by" has to be 4 be because 9-5=4 and that’s the "Shifted up by".
3. "Shifted down by" has to be 1 because 5-4=1 and that’s the "Shifted down by" will be.
Yoji shared this solution for level 2:
Level 2
9, 17, 25, 33, 41
If you subtract all the numbers by the one below it, it will be 8.
The ⬆️ is 1 because 9 is 1 greater than 8. This also works for 17-16. (The 16 is 8×2)
The ⬇️ is 7. This is because 8-1=7. This also works by doing 8×2-9.
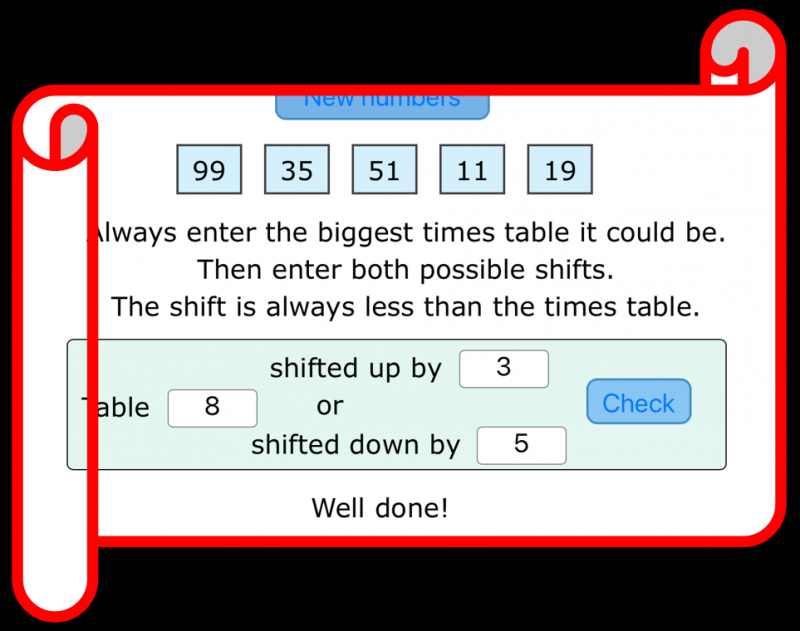
Akira solved level 3:
I had a go on Level 3. The hint helped me, where it said to put the numbers in order.
When I put the numbers in order, I did 19-11 which is eight, and shifted up by 3, and down by 5. It has a connection because 3+5 can be 8. It was pretty easy because it gave me low numbers.
Solution: 19-11=8 → 8-5 or 8-3 or 5+3=8
Number order:11,19,35,51,99
Yoji shared their solution for level 4:
13, 31, 25, 19, 67
I had a go on level 4
First, put the numbers in order.
13, 19, 25, 31, 67
Then you subtract each number from the number behind it.
19-13=6 25-19=6 31-25=6 67-31=36
As you can see, the answers are all multiples of 6.
That means that inside the box next to Tables will be 6.
Next in the "shifted up by" box, you will have to add 1.
The "shifted up by" box means that how much answer cannot be greater than the number in the tables.
If you do that, it will be 13-6=7, but the answer will be greater than 6.
To make it less than 6, you have to do 2×6 and get 12.
Then you do 13-12 and get 1.
Finally for the shifted down by, I multiplied 6 to the closest number to 13 that is greater than 13.(which is 18)
Then you do 18-13 and get 5. That is the answer.
Number order:11,19,35,51,9
We also received a similar solution from Joshua, Tom and Daichi. Take a look at Joshua, Tom and Daichi's full solution. It's very interesting to see how all of you solved this problem, step by step.
We received an anonymous solution which said:
Find the pattern: second number = first number + increase
Example: 14, 22, 30, 38, 46
Notice how each number is 8 more than the last number
Therefore this pattern is a shifted 8's times table
Finding the shift:
Using addition or subtraction on multiples of 8 to get the pattern
1. 16 - 2 = 14, meaning the table could have been shifted 2 down
2. 8 + 6 = 14, meaning the table could have been shifted 6 up
However, by this logic, you could also say that the table had also been shifted 10 down (since 24 is also a multiple of 8 and 24 - 10 = 14). If this pattern extends forever, there would be infinite amount of ways to shift it.
This is a good point - we can always keep shifting the times table further, by adding multiples of the times table to the shifted amount!
Teachers' Resources
Why do this problem?
This problem will help learners become more familiar with multiplication (times tables) facts, by encouraging them to think about number properties and therefore gain a deeper understanding of our number system. It also makes the inverse relationship between multiplication and division explicit. The interactivity will capture learners' curiosity so that they are motivated to find solutions and, in this way, they will be doing meaningful calculation practice.
In conjunction with the other tasks in this group, this task also offers the chance to focus on any of the five key ingredients that characterise successful mathematicians.
Possible approach
Explain to the group that you're thinking of a times table and ask them if they can work out which it is. Write these numbers on the board as you say them: 3, 6, 9, 12. What about 4, 8, 12, 16? 45, 50, 55, 60? How do they know?
Keep going until the class is confident and fluent at working out the times tables. To avoid shouting out, learners could write their answers on mini whiteboards.
Explain that you will now give the class some random numbers from a times table rather than the first four numbers. Write up, for example, 60, 20, 100, 50. Discuss that these are all in the 2, 5 and 10 times tables, but we're only interested in finding the largest possible times table, so we'll say these are numbers in the 10 times table.
Now show the interactivity from the problem and alert the children to the fact that it does something slightly different (but don't tell them what!). Generate a set of numbers using Level 1 and give the class a short time to discuss with their partner what they think the computer has done. Do the same a couple more times, without any whole-class sharing, but giving pairs a little time to refine their ideas. Then bring the class together and discuss what they think is going on. Link what they say to the terminology of "table" and "shift" that the computer uses. Emphasise that the table should always be the largest possible, and the shift should always be less than the table.
Ideally, each pair would now work at a computer or tablet to develop a method of finding the table and shift with ease. If that isn't possible, generate a dozen or so examples at appropriate levels, and write them on the board for the class to work on. Learners could also work in pairs and create examples for their partners to work out.
Once pairs are finding the table and shift easily, bring the class together. Generate a new example, using level 2 or 3, and ask a pair to talk through their thinking as they work towards the solution, but ask them to stop short of actually giving the answer. The rest of the class could write the answer on mini whiteboards once they've heard enough to work it out. Repeat, giving other pairs the opportunity to share their thinking.
Possible questions
What is the same between numbers in a times table and numbers in the shifted times table?
What can you learn from the difference between any two numbers in a shifted times table?
How do you find the shift once you've worked out the table?
Possible extension
Light the lights again uses some similar ideas. The Shifting Times Table problem is a Stage 3 version of this task. The tasks in this group would also provide useful follow-up to this one.
Possible support
Children may find a table square helpful as they work on this activity.
