Holes

I've made some cubes and some cubes with holes in. This challenge invites you to explore the difference in the number of small cubes I've used. Can you see any patterns?

Age

5 to 11

Challenge level

Primary

Algebra

Sequences

Exploring and noticing Working systematically Conjecturing and generalising Visualising and representing Reasoning, convincing and proving

Being curious Being resourceful Being resilient Being collaborative

Problem

Here we have three solid cubes and three cubes that have holes.

They're just the first three in a series that could go on and on.

I was wondering about the number of cubes used in each ...

Then I thought about the difference between those numbers.

So, for example, I found that the first cube, $3$ by $3$ by $3$, used $27$ cubes.

The same cube with holes used $20$ cubes, a difference of $7$.

You can call these types of cubes what you like but I called them "Solid" and "Frame".

How about exploring the numbers for the next few Solid and Frame cubes?

Do you notice any patterns?

Can you explain any of the patterns?

Student Solutions

Well done to everybody who discovered patterns in this sequence.

Nina from CHPS in Australia calculated the numbers for the first few solid and frame cubes, and noticed a pattern in the numbers for the frame cubes:

The 1st cube has 27 cubes with no holes, but with holes it has 20 cubes.

1st cube: (3 x 3)

Solid: 27

Frame: 20

Difference: 7

2nd cube: (4 x 4)

Solid: 64

Frame: 32

Difference: 32

3rd cube: (5 x 5)

Solid: 125

Frame: 44

Difference: 81

I notice that the frame is increasing by 12. With this information I can predict what the frame of a 6x6 cube would be.

Prediction

Solid: 216

Frame: 56

Good prediction! Lots of children agreed that the frame number is increasing by 12 each time, but nobody could quite explain why this was happening.

Nico from Twyford School in the UK calculated some more of the numbers, and found a way to calculate the number of cubes in a frame of any size:

Solid	Frame	Difference
3 by 3 by 3 = 27	20	7
4 by 4 by 4 = 64	32	32
5 by 5 by 5 = 125	44	81
6 by 6 by 6 = 216	56	160
7 by 7 by 7 = 343	68	275
8 by 8 by 8 = 512	80	432
12 by 12 by 12 = 1728	128

Patterns

3 by 3 by 3 is 3³ which is 27. So to work out any solid cube you just times the number by itself and again.

To work out the frame you add on 12 from the last time.

To calculate the number of cubes in a frame you take away the number 3 (from the side length) and then multiply it by 12 and then add 20 to it.

I agree that this formula works, Nico - I wonder why it works?

We received several solutions from the children at Pakuranga Heights School in New Zealand. J noticed a different pattern, which you can check by looking at the numbers in Nico's table above:

The differences of the solid and frame cubes their times tables go up in sequence E.G 7,8,9.

This is a very interesting pattern, J. I wonder if this is a coincidence for the first few numbers, or if this will always be true?

Emma from St Elizabeth Catholic Primary School in the UK found the following patterns:

Patterns:
. The number of cubes in a solid is always cube x cube x cube (for instance 2x2x2=8, 6x6x6=216)
. The number of cubes in the frames are increasing by 12 (8, 20, 32, 44, 56, 68 and so on).
. To get the difference between the solids and the frames you need to use a formula:
(cube-2 x cube-2) x 6 + (cube-2 x cube-2 x cube-2)
For instance for a 6x6x6 cube, the frame is 6x6x6 minus (4x4)x6 + 4x4x4+160. So 216 solid-160=56 cubes in a frame

I agree that this formula works as well, Emma. I wonder why it works?

Om from St Leonard’s Primary School in Exeter, UK explained why this formula works for calculating the frame numbers:

I found a relationship between solid and frame numbers. I made a formula that can convert from solid to frame numbers.

n = length of side

formula = n³ - (n-2)³ - 6(n-2)²

I came to this by first working out that in the middle of a normal cube, one block deep, there is a cube which is the (side length - 2)³. In a frame, this wouldn't be there, so if I take this out of a cube, it will be a hollow cube. At this point, I still had to make the 'windows' of the hollow cube. I noticed there were six windows and each window had a width of 2 less than the width of the cube. Since the holes were all square (i.e. they have the same width and height), I multiplied the area of the windows by the number of windows which is always 6.

Then, I subtracted them both from the solid cube and the chunk of the equation saying -(n - 2)³ - 6(n - 2)² is the difference between the two figures.

6³ - (6 - 2)³ - 6(6 - 2)² = frame

216 - 4³ - (6 × 4²) = frame

216 - 64 - 96 = frame = 56

This is a very clear explanation, Om. I wonder how the n³ - (n-2)³ - 6(n-2)² formula and the 12(n - 3) + 20 formula that Nico found can both give the same answers for the frame numbers?

Yuto from St. Mary’s International School in Japan found the same formula, and also drew some pictures to represent the different stages of the formula. Take a look at Yuto's full solution to see their ideas.

We also had a similar solution sent in by Joshua from St Mary's. Thank you to everybody who sent in their thoughts about this problem!

Dharma from Holywell C of E Primary School in the UK sent in this table exploring some patterns in the difference between the solid numbers and the frame numbers:

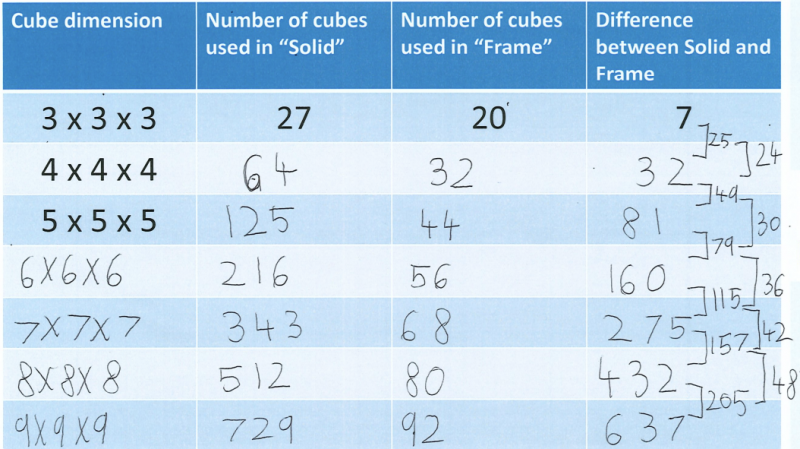
Dharma explained:

The differences of the differences of the differences, are going up in the six times table. If you keep finding the differences you will get 0.

This is very interesting, Dharma - at first glance it doesn't look like there is a pattern in these numbers, but you've managed to find one!

If anybody has had some practice finding the nth term of different types of sequences, it might be interesting to have a think about how we can tell from Dharma's 'differences of the differences of the differences' statement that the formula for this column will include an n³ term.

I wonder whether any of the formulas on this page can help explain the fact that J noticed earlier, that the difference between the solid and frame numbers for a cube of side length n is always in the n+4 times table?

Teachers' Resources

Holes

Here we have three solid cubes and three cubes that have holes.

They're just the first three in a series that could go on and on.

I was wondering about the number of cubes used in each ...

Then I thought about the difference between those numbers.

So, for example, I found that the first cube, $3$ by $3$ by $3$, used $27$ cubes.

The same cube with holes used $20$ cubes, a difference of $7$.

You can call these types of cubes what you like but I called them "Solid" and "Frame".

How about exploring the numbers for the next few Solid and Frame cubes?

Do you notice any patterns?

Can you explain any of the patterns?

Why do this problem?

This problem gives opportunities for pupils to explore, to discover, to analyse and communicate. It's a real catalyst for pupils' curiosity. It allows pupils to approach it in whatever way they find most helpful. It also provides opportunities for using and extending visualising skills. The activity also opens out the possibility of pupils asking “I wonder what would happen if . . .?” showing their resilience and perseverance.

Possible approach

You could show the group models of the first solid cube and first frame cube. Invite them to talk to a partner about them and to share their observations with the whole class. You could then ask pairs to suggest what the 'next' two cubes would look like. You could also ask them to explain why they think you started with a $3$ by $3$ by $3$ cube rather than, say, a $2$ by $2$ by $2$ one.

You may like to challenge learners to find the number of small cubes each is made up of and they could share their methods for doing so. Some may need to make their own model to help them, but others will be able to calculate the number of cubes using certain facts they know. By sharing different methods, some children may take on a new method because they find it works better for them compared with the original way they chose. Pupils can then work on the challenge itself in pairs or small groups.

Give the class the freedom to choose their own way of recording their findings and share these in the plenary, as well as sharing results. Encourage explanations of the number patterns, rather than just 'pattern spotting'.

Key questions

Tell me about about what you've found.

Are there other ways of showing what you've found?

Possible extension

Learners could explore what happens when they count the square surfaces that are visible on each small cube.

Possible support

Having plenty of interlocking cubes available will aid some children, whereas others may wish to use computer drawing programs and/or spreadsheet software.

Or search by topic

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners

Holes

Problem

Getting Started

Student Solutions

Teachers' Resources

Holes

Why do this problem?

Possible approach

Key questions

Possible extension

Possible support