Escher tessellations
This practical investigation invites you to make tessellating
shapes in a similar way to the artist Escher.
Age
7 to 11
Challenge level
Problem
I've been seeing tessellations in lots of different places and realise that they are being used in so many situations such as tiling on both walls and floors.
At visits to various garages and big buildings I've seen one particular style of tile that's good to create and tessellate. Here's how it goes.
A - You start with a rectangle that is twice as long as it is wide.
B - Then you make a roof shape at one end - this one happens to be as high as a quarter of the base of the triangle it has made.
C - You then put the same triangle on the other end.
D - On one side you take a bite out just the same size as the roof triangle.
E - Then do the same at the other end of the opposite side.
Image
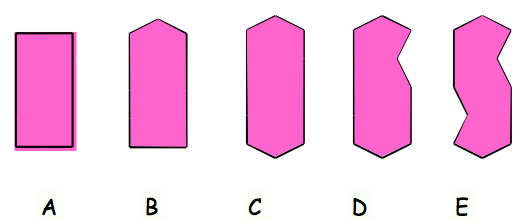
Now you have the tile shape for tessellating like the floors I've seen.
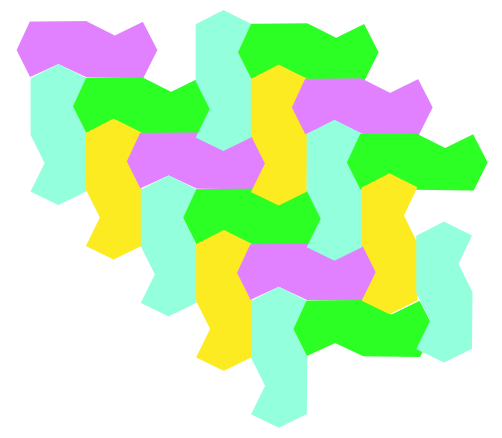
One way I've seen is like this:
Image
At another garage I saw a tile like this:
Image
These were put together like this [I've coloured them to see the pattern more easily]:
Image
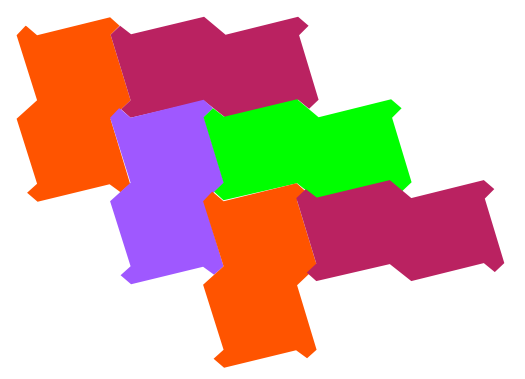
or
Image
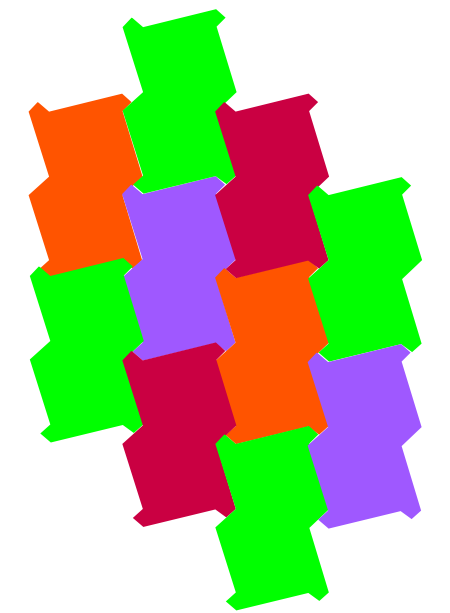
Now it's time for you to try and find some ways of putting shapes - like these two examples - together!
Let us know how you made your shape and do send us some pictures of your tessellations.
Getting Started
You'll need a number of the shapes to get going and they really do need to be accurate.
Can you find some way of copying your shape several times? You might, for example, be able to get someone to put thick paper or thin card through a photocopier..
If youhave a digital camera that really makes recording a lot easier.
Student Solutions
Thanks to Jannis from Long Bay Primary for her tessellations:
Image
Do send us some of your own if you try this investigation too.
Teachers' Resources
Escher Tesselations
I've been seeing tessellations in lots of different places and realise that they are being used in so many situations such as tiling on both walls and floors.
At visits to various garages and big buildings I've seen one particular style of tile that's good to create and tessellate. Here's how it goes.
A - You start with a rectangle that is twice as long as it is wide.
B - Then you make a roof shape at one end - this one happens to be as high as a quarter of the base of the triangle it has made.
C - You then put the same triangle on the other end.
D - On one side you take a bite out just the same size as the roof triangle.
E - Then do the same at the other end of the opposite side.
Image
Now you have the tile shape for tessellating like the floors I've seen.
One way I've seen is like this:
Image
At another garage I saw a tile like this:
Image
These were put together like this [I've coloured them to see the pattern more easily]:
Image
or
Image
Now it's time for you to try and find some ways of putting shapes - like these two examples - together!
Let us know how you made your shape and do send us some pictures of your tessellations.
Why do this problem?
This very practical activity creates an opportunity for investigating properties of shapes in an informal way. Asking them to tackle this in pairs or small groups will mean that talking to each other will be necessary, and this discussion can reveal a great deal to you about their knowledge and mathematical skills.Possible approach
It is important to consider what part the recording of this work plays in the development of children's concepts relating to tessellation.Key questions
Possible extension
Those of you who are Escher fans will probably have resources that can extend this activity.You may have carried out transformations (like the first one on the rectangle) on other shapes.
A very simple idea can be explored with equilateral triangles - an Escher favourite:
Image
Image
These can be put together in different ways.
Image
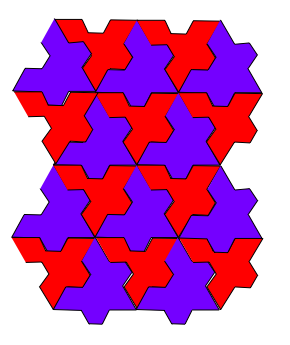
Image
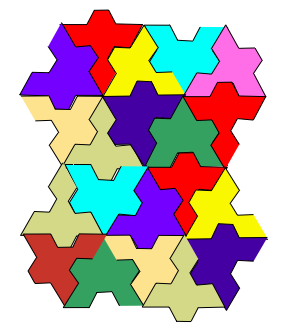
Again there's lots of opportunity for discussion with certain pupils. For example, "Is there always a need for two different kinds of the transformed triangles?"