Diagonal sums Sudoku
Solve this Sudoku puzzle whose clues are in the form of sums of the numbers which should appear in diagonal opposite cells.
Problem
by Henry Kwok
Image
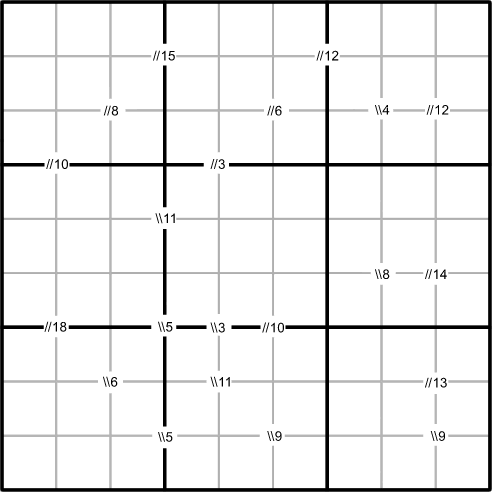
Rules of Diagonal Sums Sudoku
Like the standard Sudoku, this Sudoku has two basic rules:
- Each column, each row and each box (3$\times$3 subgrid) must have the numbers 1 to 9.
- No column, row or box can have two squares with the same number.
The puzzle can be solved with the help of clue-numbers which are written after slash marks on the intersections of border lines. Each clue-number is the sum of two digits in the two squares that are diagonally adjacent to each other. The position of each pair of diagonally adjacent squares is indicated by either two forward slash marks // or two backward slash marks \\.
For example, the //12 on the border of the top right hand box means that possible pairs of numbers in the cells above-right and below left are:
3 and 9, 9 and 3; 4 and 8, 8 and 4; 5 and 7, or 7 and 5 respectively.
Similarly, the \\6 in the bottom left box means that possible pairs of numbers in the cells above-left and below-right are:
1 and 5, 5 and 1; 2 and 4, or 4 and 2 respectively.
Getting Started
Caitlin kept a record of the order in which she filled the Sudoku.
She filled the cell marked 1 first, then the cell marked 2, then the cell marked 3...
You might like to retrace her route to fill in the cells in the same order, though this is just one possible route through the problem.
You can print her journey here.
Student Solutions
| 5 | 1 | 3 | 9 | 6 | 2 | 7 | 8 | 4 |
| 4 | 8 | 6 | 7 | 3 | 5 | 1 | 2 | 9 |
| 7 | 2 | 9 | 8 | 1 | 4 | 6 | 3 | 5 |
| 8 | 6 | 5 | 2 | 7 | 9 | 3 | 4 | 1 |
| 1 | 7 | 4 | 6 | 5 | 3 | 2 | 9 | 8 |
| 3 | 9 | 2 | 1 | 4 | 8 | 5 | 6 | 7 |
| 9 | 5 | 8 | 3 | 2 | 7 | 4 | 1 | 6 |
| 2 | 4 | 1 | 5 | 8 | 6 | 9 | 7 | 3 |
| 6 | 3 | 7 | 4 | 9 | 1 | 8 | 5 | 2 |
