The clockmaker's birthday cake
The clockmaker's wife cut up his birthday cake to look like a clock
face. Can you work out who received each piece?
Problem
The clockmaker's wife was a mathematician. On his birthday she invited five other couples to tea. She made a birthday cake decorated to look like a clock face with numbers made from pink icing.
She cut up the cake into twelve slices with a number on each slice:
Image
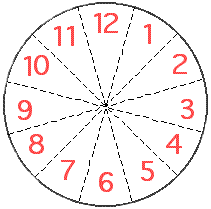
The slices that she gave each couple added to the same number. What was the number?
The clockmaker had the slice with $12$ on it because it was his birthday. One guest wanted $7$ because it was her lucky number. All the slices that went to men added to a number equal to those that went to the women.
How could this be arranged?
Getting Started
What do all the numbers on the cake add to? How could you use this
total to work out the sum of the numbers on each couple's slices?
Student Solutions
Charles sent in his solution:
All the numbers together add up to $1+2+3+...+12=78$. There are six couples, each with the same total amount, so this must be $78/6=13$. So each couple's numbers add up to $13$.
I'm going to write the pairs in brackets, like this ( , ). The first number is the one for the man, and the second for the woman. So we know we have $(12, 1)$ and $(6, 7)$. We want the ladies' numbers and the men's numbers each to add up to $78/2=39$. Here's a way we could do it:
$(12, 1)
(6, 7)
(2, 11)
(10, 3)
(5, 8)
(4, 9)$
And $12+6+2+10+5+4=39=1+7+11+3+8+9$.
Teachers' Resources
Why do this problem?
This problem practises addition, subtraction, multiplication and division in an interesting and thought-provoking way.Key questions
What do all the numbers on the cake add to?
How many couples are there?
How can you use this total to work out the sum of the numbers on each couple's slices?
