Reach 100
Choose four different digits from 1-9 and put one in each box so that the resulting four two-digit numbers add to a total of 100.
Problem
Here is a grid of four boxes:
You must choose four different digits from 1 - 9 and put one in each box. For example:
| 5 | 2 |
| 1 | 9 |
This gives four two-digit numbers:
52 (reading along the first row)
19 (reading along the second row)
51 (reading down the left-hand column)
29 (reading down the right-hand column)
In this case their sum is 151.
Try a few examples of your own.
Is there a quick way to tell if the total is going to be even or odd?
Your challenge is to find four different digits that give four two-digit numbers which add to a total of 100.
How many ways can you find of doing it?
You may be interested in the other problems in our Mastering Mathematics: Developing Generalising and Proof Feature.
This problem is adapted from Make 200 from 'Mathematical Challenges for Able Pupils Key Stages 1 and 2', published by DfES.
Getting Started
Simply writing out the numbers $1 - 9$ along the top of your paper can be a great help.
Imagine arranging your four two-digit numbers in columns to do the addition sum, like this:
$5 \: 2$
$1 \; 9$
$5 \; 1$
$2 \; 9$
If the total has to be $100$, think about how the four digits you choose could add to make the $0$ in the units column, then look at the tens column.
Student Solutions
We had nearly 100 submissions for this challenge. Thank you to everyone who sent in their ideas.
Tipu who learns at home in India wrote :
My mother helped me type up what I did and thought.
Here is a glimpse of what arrived

and I recommend that you look at the whole document here: Tipu.docx
Well done, Tipu, you have described carefully what you did and given clear reasons for your thinking.
Alex, Jocelyn and Mackenzie from the Maths Club at South Walney Junior School wrote:
To start with, we realised that the bigger numbers 85/94 make a bigger target number. From now on, we chose smaller numbers.
We then started to look at 1/2/3/4 and tried different switching arrangements - this did not work. We noticed that the bottom right digit in the grid was used twice. So we started using the number 9 - this didn't work.
Then, systematically we tried 8. We put the 8 in its position, we doubled this digit to make 16, then added 3 + 1 to make a multiple of 10. We did this because we realised that in the column addition, the units digit must end with a zero! When we put these numbers into their grid position we worked out the 4th missing digit - which was 2!.
So our solution was 21, 38, 23 & 18.
We also found a solution for 7 in the bottom right grid using the same strategy. Our solution is 14, 27, 12 & 47.
We couldn't find a solution for the other numbers - but this raised the question 'What was so special about the numbers 7 & 8???' We are still looking for an answer to this...
. . . . .
Y5 at Rickmansworth Park JMI also responded to this challenge.
Here is the start of their work and below you can download the whole document that is well worth a read.

The full document can be viewed here: Y5.docx
Kriti from Bootham Junior School wrote:
Imagine the letters A, B, C, and D stand for 4 numbers.
(A x 10) + B + (C x 10) + D + (A x 10) + C + (B x 10) + D: (20 x A) + (11 x B) + (11 x C) + (2 x D) = 100
The eleven times table is:
1 x 11 = 11 can be eliminated because (B + C) is at least 3
2 x 11 = 22 can be eliminated for the same reason
3 x 11 = 33 can be eliminated because 67 is an odd number and (20 x A) + (2 x D) is an even number
4 x 11 = 44
5 x 11 = 55 can be eliminated for the same reason
6 x 11 = 66
7 x 11 = 77 can be eliminated for the same reason
8 x 11 = 88 can be eliminated because (20 x A) + (2 x D) is at least 24
9 x 11 = 99 can be eliminated for the same reason
A = 2, B = 3, C = 1, and D = 8,
A = 1, B = 2, C = 4, and D = 7, are the only solutions. ARE THEY?
Emad, Omar, Mehak, Nihal and Kishaaragan from Northgate Primary (Crawley) wrote:
First we tried making the units add up to ten so that there was a place holder of zero in the units. After lots of attempts we found this method wasn't working, so we tried making the units add up to 20. Because this meant you had to carry the 2 across to the tens column, the tens column had to add up to 8. When you do this you have a zero in the units and in the tens. This means you can make 100. If the units value is less or more than 20 there will no possibility of reaching 100. This made our working out quicker because we were able to stop the calculations when we knew they wouldn't work. We also tried making solutions with zero to see what happened, this helped us find lots more answers. Here are some of the solutions we found: 21 and 38, 14 and 27, 29 and 30, 23 and 18, 20 and 48, 19 and 27, 16 and 07.
Just to remind you - you had to use the digits 1 through to 9 so zero was not allowed, but if we did allow zero, 16 and 07 work.
Zach sent in a pdf which is a wonderfully clear account of how he tackled the problem. You can see the start of it below and go here to read it all: Zach.pdf
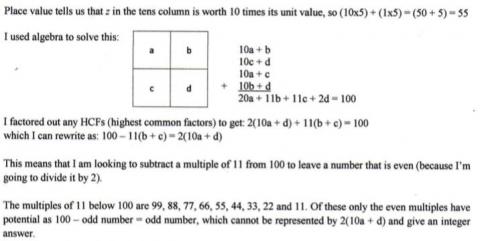
Paz from King's College, The British School of Alicante in Spain, sent in the following:
I worked on this puzzle with six other students in Year 6: Enrique, Pablo, Ivan, José Luis, Aldo and Archie. We decided to try lots of combinations and explain to each other what we noticed to help us find the answers.
If our four boxes are:
| a | b |
| c | d |
We realised that a, b and c must be quite small otherwise the total quickly went above 100 when we did:
a b; + a c; + b d; + c d
We also realised that d is quite important because we add that twice in the units column. We looked carefully at how to make b + c + 2d end in a zero.
I found one answer: a = 1; b = 4; c = 2; d = 7
And we realised we could rearrange (transpose) this: a = 1; b = 2; c = 4; d = 7
Ivan also found a different pair of answers: a = 2; b = 3; c = 1; d = 8
Or: a = 2; b = 1; c = 3; d = 8
But we can't be sure that these are all of the answers. We looked for more but we couldn't find any others.
4/6 MEP team from Lumen Christi Primary School, Point Cook in Australia sent in another excellent document that I recommend you read. You can access it here: .doc or .pdf
Just before the end of 2015 we had some good ideas from Year 4, at Kowloon Junior School, Hong Kong you can see Here.doc
There certainly was a great response to this challenge, I'm sorry we have limited space and cannot show more. Thank you all so much and well done for explaining your thoughts and observations so clearly.
Teachers' Resources
Why do this problem?
This low threshold high ceiling problem challenges pupils' understanding of place value and is a good way to practise a particular method of written addition.
Possible approach
It would be good to start with the grid drawn on the board and for you to explain the challenge orally to the group. You could use the example in the problem itself so that the task is clear.
Ask for suggestions as to how they might start and give learners a few minutes to think on their own, then share their ideas with a partner. Open this out to the whole class so that a few pairs share their thoughts with everyone. The suggestions are likely to be quite general at this stage.
Allow children to work together on the problem in their pairs. They will find mini-whiteboards or paper useful for keeping track of their calculations. After some time, bring the group together again and discuss any insights they have gained. Some pairs may have thought about the cells in the grid which make up the units digits of the four numbers, others may have concentrated on the cells which contribute to the tens digits. In either case, encourage them to explain the restrictions they have noticed, and look out for those pairs who are working systematically through the options.
Key questions
Possible extension
What is the smallest total you can make? What is the largest? Can you make all the totals in between?
Add to 200 is a very similar task to this one, so offers opportunities to think in similar ways, but with the added challenge of there being many more possible solutions.
Two and Two also requires similar systematic thinking and could be a good problem for some children to try next.
Possible support
Having digit cards available for learners to physically manipulate will help those who are reluctant to commit ideas to paper.
