Skeleton
Can you reconstruct the long division calculation from the 'skeleton'?
Problem
Amazing as it may seem the three fives remaining in the following `skeleton' are sufficient to reconstruct the entire long division sum.
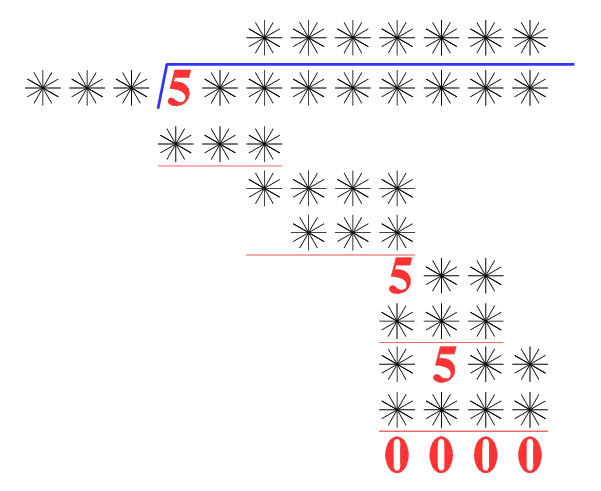
Student Solutions
There were two good solutions here, well done Chin Siang and James! The following carefully explained and well laid out solution came from Ong Chin Siang of Tao Nan School, Singapore. James Page of Hethersett High School gave the same answer and he said he used different `clues' to get his solution. A couple of James' clues may help you to get started and then you might like to try the problem for yourself before looking at Chin Siang's solution.
James' First Clue
- 0 0 - 0 - -
---------------------------
- - - ) 5 - - - - - - - -
- - -
-------
- - - -
- - -
-------
5 - -
- - -
-------
- 5 - -
- - - -
----------
0 0 0 0
| I know that these zeros have to be there because a 3 digit number cannot go into a 2 digit number and also not into some 3 digit numbers. |
Second Clue
- 0 0 - 0 - -
---------------------------
- - - ) 5 - - - - - - - -
- - -
-------
1 0 0 -
9 9 -
-------
5 - -
- - -
-------
- 5 - -
- - - -
----------
0 0 0 0
| Here we know the difference between a 4 digit number and a 3 digit number is 5 so it has to be 1004 - 999 or 1003 - 998 or 1002 - 997 or 1001- 996 or 1000 - 995. |
Here is the solution to the Skeleton problem done by Chin Siang.
X X X X X X X
-------------------
X X X ) 5 X X X X X X X X
X X X
X X X X
X X X
5 X X
X X X
X 5 X X
X X X X
0 0 0 0
Answer:
3 0 0 5 0 2 8
------------------
1 9 9 )5 9 8 0 0 0 5 7 2
5 9 7
1 0 0 0
9 9 5
5 5 7
3 9 8
1 5 9 2
1 5 9 2
0 0 0 0
Solution:
X X X X X X X
-------------------
X X X ) 5 X X
d
d
d
X X X
b
X X
c
d
d
d
-------- Number
z
e
e
f
-------- Number
y
5 X X
X X X
X 5 X X
X
a
X X
0 0 0 0
a = 5 because 5-5=0.
b = 5 because 5-5=0.
c = 1 because the number below is only 3 digits.
d = 0 and e = 9 because the difference between Number z and Number y is 5 (a single digit).
f = 5 because 1000-5=995.
So now, what is the divisor?
| 995 | = 1*995. This is impossible as we start with a number x less than 5XX. |
| = 5*199. This is possible! |
So...
X 0 0 5 X X X
-------------------
1 9 9 ) 5
g
h
0 0 0 X X X
5 X X ----------------------Number
x
1 0 0 0
9 9 5
5 X X ----------- Number
w
X X X ----------- Number
v
X 5 X X --------- Number
u
X 5 X X --------- Number
u
0 0 0 0
Number x /199 is an integer,
so, number x = 597.
5 gh = 597+1 = 598.
Number v cannot be 597 because 5XX-597 is never X5X.
The next smaller multiple of 199 is 398.
Number v = 398.
The lowest multiple of 199 that is X5XX = 8(199) = 1592.
Number u = 1592.
So, Number w = 398+159 = 557.
Thus, you will get...
3 0 0 5 0 2 8
------------------
1 9 9 )5 9 8 0 0 0 5 7 2
5 9 7
1 0 0 0
9 9 5
5 5 7
3 9 8
1 5 9 2
1 5 9 2
0 0 0 0
